Chapter: Modern Analytical Chemistry: Obtaining and Preparing Samples for Analysis
Minimizing the Overall Variance
Minimizing the Overall Variance
A final consideration in developing a sampling plan is to minimize the overall vari- ance for the analysis.
Equation 7.2 shows that the overall variance
is a function of the variance
due to the method and the variance
due to sampling. As we have seen, we
can improve the variance due to sampling
by collecting more samples of proper
size. Increasing the number of times we analyze each sample improves
the variance due to the method.
If ss2 is significantly greater
than sm2 , then the method’s variance can be ignored and equation 7.7 used to estimate the number of samples to analyze.
Analyzing any sample
more than once
will not improve
the overall variance, since the variance due to the method is insignificant.
If sm2 is
significantly greater than ss2, then we
only need to collect and analyze a single sample. The number of replicate
analyses, nr, needed to
minimize the error due to the method is given by an equation similar to
equation 7.7
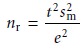
Unfortunately, the simple
situations just described are often the exception. In many
cases, both the sampling variance
and method variance
are significant, and both
multiple samples and replicate analyses
of each sample
are required. The over-
all error in this circumstance is given by
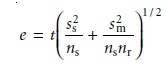 7.8
7.8
Equation 7.8 does not have a unique
solution because different combinations of ns
and nr give the
same overall error.
The choice of how many
samples to collect
and how many times
each sample should
be analyzed is determined by other concerns, such as the cost of collecting and analyzing samples,
and the amount
of available sample.
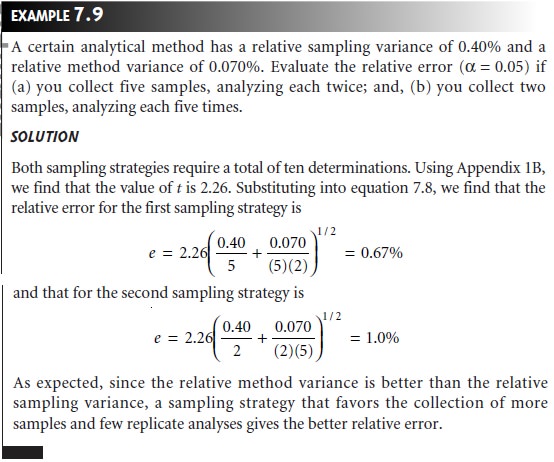
Related Topics