Ionic Equilibrium | Chemistry - Evaluate yourself - with Solutions | 12th Chemistry : UNIT 8 : Ionic Equilibrium
Chapter: 12th Chemistry : UNIT 8 : Ionic Equilibrium
Evaluate yourself - with Solutions
Evaluate yourself – 1
Classify the following as acid (or) base using Arrhenius concept i)HNO3 ii) Ba(OH)2 iii) H3 PO4 iv) CH3COOH
Answer:
acid : (i) HNO3 iii) H3PO3 iv) CH3COOH
base : ii) Ba (OH)2
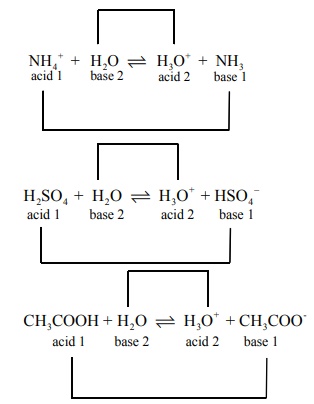
Write a balanced equation for the dissociation of the following in water and identify the conjugate acid –base pairs. i) NH4+ ii) H2SO4 iii) CH3COOH.
Answer:
Evaluate yourself – 3
Identify the Lewis acid and the Lewis base in the following reactions.
i. CaO+CO2 → CaCO3
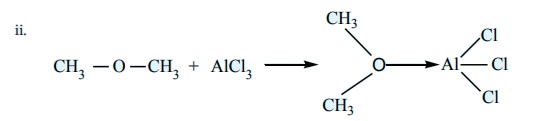
Answer:
i) CaO - Lewis base ; CO2 –Lewisacid
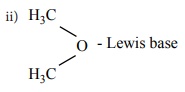
AlCl3 - Lewis acid
Evaluate yourself – 4
H3BO3 accepts hydroxide ion from water as shown below
H3BO3 (aq) + H2O(l) ↔ B(OH)4- +H+
Predict the nature of H3 BO3 using Lewis concept
Answer:
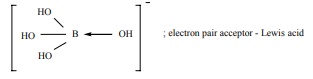
Evaluate yourself – 5
At a particular temperature, the Kw of a neutral solution was equal to 4 ×10-14. Calculate the concentration of [H3O+ ] and [OH- ].
Answer:
Given solution is neutral
∴ [ H3O+ ] = [OH- ]
Let [H3O+ ] = x ; then [OH- ] = x
Kw = [H3O+ ][OH- ]
4× 10-14 = x . x
x2 = 4 × 10-14
x = √ 4 × 10-14 = 2 ×10-7
Evaluate yourself – 6
a. Calculate pH of 10-8M H2 SO4
b. Calculate the concentration of hydrogen ion in moles per litre of a solution whose pH is 5.4
c. Calculate the pH of an aqueous solution obtained by mixing 50ml of 0.2 M HCl with 50ml 0.1 M NaOH
a) Answer
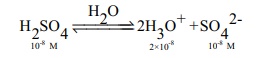
In this case the concentration of H2SO4 is very low and hence [H3O+ ] from water cannot be neglected
∴[H3O+] = 2 ×10-8 (from H2SO4 ) + 10-7
(from water)
= 10-8 (2+10)
= 12×10-8 = 1.2 ×10-7
pH = - log10[H3O+]
= - log10 (1.2 × 10-7)
= 7 - log101.2
= 7 -0.0791
= 6.9209
b) Answer
pH of the solution = 5.4
[H3O+] = antilog of (-pH)
= anitlog of (-5.4)
= antilog of (-6 + 0.6) = 6.6
= 3.981×10-6
i.e., 3.98 × 10-6 mol dm-3
c) Answer
No of moles of HCl = 0.2× 50 × 10-3 = 10 × 10-3
No of moles of NaOH = 0.1 × 50 × 10-3 = 5 × 10-3
No of moles of HCl after mixing = 10 × 10-3 - 5 × 10-3
= 5 ×10-3
after mixing total volume = 100mL
∴ Concentration of HCl in moles per litre = 5×10-3mole / 100×10-3L
[H3O+] = 5 ×10-2 M
pH = - log (5 × 10-2 )
= 2 - log 5
= 2 - 0.6990
= 1.30
Evaluate yourself – 7
Kb for NH 4 OH is 1.8 × 10-5 . Calculate the percentage of ionisation of 0.06M ammonium hydroxide solution.
Answer:
α = √(Kb / C) = 1.8 ×10-5 / 6 × 10-2
= √ 3×10-4
= 1.732 × 10-2
= 1.732 / 100 = 1.732%
Evaluate yourself – 8
a. Explain the buffer action in a basic buffer containing equimolar ammonium hydroxide and ammonium chloride.
b. Calculate the pH of a buffer solution consisting of 0.4M CH3COOH and 0.4M CH3COONa . What is the change in the pH after adding 0.01 mol of HCl to 500ml of the above buffer solution. Assume that the addition of HCl causes negligible change in the volume. Given: ( Ka = 1 . 8 ×10−5. )
a) Answer
Dissociation of buffer components
NH4OH (aq) ↔ NH4+ (aq) + OH- (aq)
NH4 Cl → NH4+ +Cl+
Addition of H+
The added H+ ions are neutralized by NH4OH and there is no appreciable decrease in pH.
NH4OH(aq) + H+ → NH4+ (aq) + H2O(l)
Addition of OH–
NH4 + (aq) + OH- (aq) → NH4OH (aq)
The added OH- ions react with NH4+ to produce unionized NH4OH. Since NH4OH is a weak base, there is no appreciable increase in pH
b) Answer
pH of buffer
CH3COOH(aq) ↔ CH3COO - (aq) + H + (aq)
0.4-α ↔ α + α
CH3 COONa(aq) →CH 3 COO - (aq) + Na+ (aq)
0.4 → 0.4 + 0. 4
[H+ ]= K a [CH 3 COOH] / [CH3COO- ]
[CH3COOH] = 0.4 - α ≈ 0.4
[CH3COO- ] = 0.4 + α ≈ 0.4
∴ [H+] = Ka (0.4) / (0.4)
[H+] = 1.8 ×10-5
∴ pH = - log (1.8 ×10-5 ) = 4.74
Addition of 0.01 mol HCl to 500ml of buffer
Added [H+ ] = 0.01 mol / 500 mL = 0.01 mol / 1/2L
= 0.02M
CH3 COOH(aq) ↔ CH3COO- (aq) + H+ (aq)
0.4-α ↔ α + α
CH3 COONa → CH3COO- +Na+
0.4 → 0.4 + 0.4
CH3COO- + HCl → CH3COOH+Cl-
(0.02) + 0.02 → 0.02 + 0.02
∴ [CH3COOH] = 0.4 - α + 0.02 = 0.42 - α ≈ 0.42
[CH3COO- ] = 0.4 + α - 0.02 = 0.38 + α ≈ 0.38
[H+] = [(1.8 × 10-5 ) (0.42)] / (0.38)
[H+ ] = 1.99 × 10-5
pH = - log (1.99 ×10-5 )
= 5 - log 1.99
= 5 - 0.30
= 4.70
Evaluate yourself – 9
a. How can you prepare a buffer solution of pH 9. You are provided with 0.1M NH4OH solution and ammonium chloride crystals. (Given: pKb for NH4OH is 4.7 at 25 C .
b. What volume of 0.6M sodium formate solution is required to prepare a buffer solution of pH 4.0 by mixing it with 100ml of 0.8M formic acid. (Given: pKa for formic acid is 3.75. )
a) answer
pOH = pKb + log ([salt]/[base])
We know that
pH + pOH = 14
∴ 9 + pOH = 14
⇒ pOH = 14 - 9 = 5
5 = 4.7 + log ([NH4Cl]/[NH4OH])
0.3=log ( [NH4Cl]/0.1)
[NH4Cl] / 0.1 = antilog of (0.3)
[NH4Cl] = 0.1M × 1.995
= 0.1995 M
= 0.2M
Amount of NH4Cl required to prepare 1 litre 0.2M solution
= Strength of NH4Cl × molar mass of NH4Cl
= 0.2 × 53.5
= 10.70 g
10.70 g ammonium chloride is dissolved in water and the solution is made up to one litre to get 0.2M solution. On mixing equal volume of the given NH4OH solution and the prepared NH4Cl solution will give a buffer solution with required pH value (pH = 9).
b) answer
pH = pKa +log ( [salt]/[acid] )
4 = 3.75+log ([sodium formate] /[formic acid])
[Sodium formate] = number of moles of HCOONa = 0.6 × V × 10−3
[formic acid] = number of moles of HCOOH
= 0.8 × 100 × 10−3
= 80 × 10−3
4 = 3.75 + log (0.6V/80)
0.25 = log (0.6V/80)
antilog of 0.25 = 0.6V/80
0.6V = 1.778 × 80
= 1.78 × 80
= 142.4
V = 142.4 mL / 0.6 = 237.33mL
Evaluate yourself – 10
Calculate the i) hydrolysis constant, ii) degree of hydrolysis and iii) pH of 0.05M sodium carbonate solution ( pKa for HCO3− is 10.26).
Answer:
Sodium carbonate is a salt of weak acid, H2CO3 and a strong base, NaOH, and hence the solution is alkaline due to hydrolysis.
Na2CO3 (aq) → 2Na+ (aq) + CO32- (aq)
CO32- (aq)+ H2O (l) ↔ HCO3- +OH-
i) h= √{ (Kw) / (Ka × C) }
= √ ( 1 × 10-14 / 5.5 × 10-11 × 0.05 )
h = 6.03 × 10-2
Given that pKa =10.26
pKa = -log Ka
i.e., Ka =antilog of (-pKa )
= antilog of (-10.26)
= antilog of (-11 + 0.74)
= 10-11 × 5.5
[antilog of 0.74 = 5.49 ≈ 5.5]
ii) Kh = Kw/Ka = ( 1×10-14 / 5.5 ×10-11 )
= 1.8 ×10-4
iii) pH = 7 + pKa/2 + logC/2
= 7 + (10.26/2) + (log 0.05)/2 = 7 + 5.13 −0.65
= 11.48
Related Topics