Test of Hypotheses For Equality of Means of Two Populations (Population Variances are Unknown)

TEST OF HYPOTHESES FOR EQUALITY OF MEANS OF TWO POPULATIONS (POPULATION VARIANCES ARE UNKNOWN)

Procedure:

Step-1 : Let µ_X and σ_X² be respectively the mean and the variance of Population -1. Also, let µ_Y and σ_Y² be respectively the mean and the variance of Population -2 under study. Here σ_X² and σ_Y² are assumed to be unknown.

Frame the null hypothesis as H₀: µ_X = µ_Y and choose the suitable alternative hypothesis from

(i) H₁: µ_X ≠ µ_Y (ii) H₁: µ_X> µ_Y (iii) H₁: µ_X< µ_Y

Step 2 : Let (X₁, X₂, …, X_m) be a random sample of m observations drawn from Population-1 and (Y₁, Y₂, …, Y_n) be a random sample of n observations drawn from Population-2, where m and n are large (m ≥30 and n ≥30). Here, these two samples are assumed to be independent.

Step 3 : Specify the level of significance, α.

Step 4 : Consider the test statistic

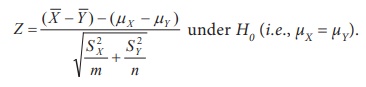
i.e., the above test statistic is obtained from Z considered in the test described in Section 1.11 by substituting S_X² and S_Y² respectively for σ_X² and σ_Y²

The approximate sampling distribution of the test statistic 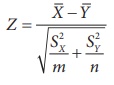 under H₀ is the N(0,1) distribution.

Step 5 : Calculate the value of Z for the given samples (x₁, x₂, ...,x_m) and (y₁, y₂, …, y_n) as

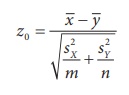
Here  and  are respectively the values of  and  for the given samples.

Also, s_x² and s_y² are respectively the values of S_X² and S_Y² for the given samples.

Step 6 : Find the critical value, z_e, corresponding to α and H₁ from the following table

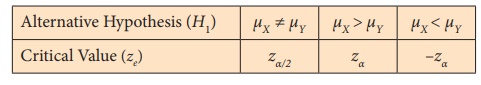
Step 7 : Make decision on H₀ choosing the suitable rejection rule from the following table corresponding to H₁.

Example 1.11

A Model Examination was conducted to XII Standard students in the subject of Statistics. A District Educational Officer wanted to analyze the Gender-wise performance of the students using the marks secured by randomly selected boys and girls. Sample measures were calculated and the details are presented below:

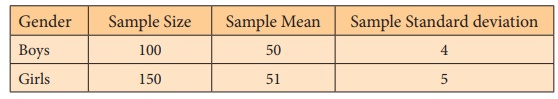
Test, at 5% level of significance, whether performance of the students differ significantly with respect to their gender.

Solution:

Step 1 : Let μ_X and μ_Y denote respectively the average marks secured by boys and girls in the Model Examination conducted to the XII Standard students in the subject of Statistics. Then, the null and the alternative hypotheses are

Null hypothesis: H₀: µ _X = µ_Y

i.e., there is no significant difference in the performance of the students with respect to their gender.

Alternative hypothesis: H₁ : µ _X ≠ µ_Y

i.e., performance of the students differ significantly with the respect to the gender. It is a two-sided alternative hypothesis.

Step 2 : Data

The given sample information are

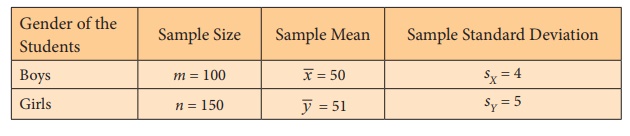
Since m ≥ 30 and n ≥ 30, both the samples are large.

Step 3 : Level of significance

 α= 5%

Step 4 : Test statistic

The test statistic under H₀ is

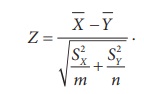
The sampling distribution of Z under H₀ is the N(0,1) distribution.

Step 5 : Calculation of the Test Statistic

The value of Z is calculated for the given sample informations from

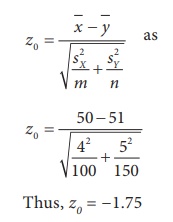
Step 6 : Critical value

Since H₁ is a two-sided alternative, the critical value at 5% level of significance is z_e = z_0.025 = 1.96.

Step 7 : Decision

Since H₁ is a two-sided alternative, elements of the critical region are determined by the rejection rule |z₀| ≥ z₀ . Thus it is a two-tailed test. But, |z₀|= 1.75 is less than the critical value z_e = 1.96. Hence, it may inferred as the given sample information does not provide sufficient evidence to reject H₀. Therefore, it may be decided that there is no sufficient evidence in the given sample to conclude that performance of boys and girls in the Model Examination conducted in the subject of Statistics differ significantly.