Shear Connections With Bearing Type Bolts

SHEAR CONNECTIONS WITH BEARING TYPE BOLTS

In this section the force transfer mechanisms of bearing and friction type of bolted connections are described.

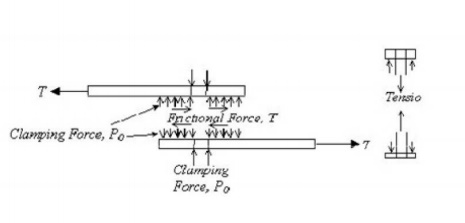
1. Force transfer of bearing type bolts

Figure 13 shows the free body diagram of the shear force transfer in bearing type of bolted connection. It is seen that tension in one plate is equilibrated by the bearing stress between the bolt and the hole in the plate. Since there is a clearance between the bolt and the hole in which it is fitted, the bearing stress is mobilised only after the plates slip relative to one another and start bearing on the bolt

.The section x-x in the bolt is critical section for shear. Since it is a lap joint there is only one critical section in shear (single shear) in the bolt .In the case of butt splices there would be two critical sections in the bolt in shear (double shear), corresponding to the two cover plates.

2. Design shear strength of bearing type bolts

The failure of connections with bearing bolts in shear involves either bolt failure or the failure of the connected plates. In this section, the failure modes are described along with the codal provisions for design and detailing shear connections. In connections made with bearing type of bolts, the behaviour is linear until

i) yielding takes place at the net section of the plate under combined tension and flexure or

 ii) shearing takes place at the bolt shear plane or

iii) failure of bolt takes place in bearing,

iv) failure of plate takes place in bearing and

v) block shear failure occurs. Of these,

i) and will be discussed in the chapter on tension members. The remaining three are described below.

3. Shearing of bolts

The shearing of bolts can take place in the threaded portion of the bolt and so the area at the root of the threads, also called the tensile stress area At, is taken as the shear area As. Since threads can occur in the shear plane, the area Ae for resisting shear should normally be taken as the net tensile stress area, An, of the bolts. The shear area is specified in the code and is usually about 0.8 times the shank area. However, if it is ensured that the threads will not lie in the shear plane then the full area can be taken as the shear area. A bolt subjected to a factored shear force (Vsb) shall satisfy   as per cl. 10.3.2 of IS 800:2007, where  . 10.3.3 of the code.

Here V_nsb = nominal shear capacity of a bolt, calculated by in which f_u = ultimate tensile strength of a bolt; n_n = number of shear planes with threads intercepting the shear plane; n, = number of shear planes without threads intercepting the shear plane; A_sb = nominal plain shank area of the bolt; and A_nb = net shear area of the bolt at threads, may be taken as the area corresponding to root diameter at the thread as given in Table 5 and ?_mb = 1.25. For bolts in single shear, either n_n or n_s is one and the other is zero. For bolts in double shear the sum of n_n and n_s is two.

Table 5 Tensile area of ordinary bolts (Grade 4.6)

Bolt size, d (mm)          12       16      20      22      24      27      30      36

Tensile stress area (mm2)        84.3   157    245    303    353    459    561    817

2.2. Bearing failure

If the connected plates are made of high strength steel then failure of bolt can take place by bearing of the plates on the bolts. If the plate material is weaker than the bolt material, then failure will occur by bearing of the bolt on the plate and the hole will elongate. The beating area is given by the nominal diameter of the bolt times the combined thickness of the plates bearing in any direction. A bolt bearing on any plate subjected to a factored shear force (Vsb) shall satisfy as  Vsb < = Vdb per cl. 10.3.2 of IS 800:2007, where as Vdb = Vdpb = Vnpb/?mb given by cl. 10.3.4 of the code where, ?mb = 1.25 and V_npb = bearing strength of a bolt, calculated as V_npb = 2.5k_bdtf_u where f_u = smaller of the ultimate tensile stress of the bolt and the ultimate tensile stress of the plate, d = nominal diameter of the bolt, t = summation of the thicknesses of the connected plates experiencing bearing stress in the same direction and k_b is smaller of e/3d₀, p/3d₀-0.25, f_ub/f_u, 1.0 where e, p = end and pitch distances of the fastener along bearing direction; d₀ = diameter of the hole; f_ub, f_u = Ultimate tensile stress of the bolt and the ultimate tensile stress of the plate, respectively.

The underlying assumption behind the design of bolted connections, namely that all bolts carry equal load is not true in some cases. In long joints, the bolts farther away from the centre of the joint will carry more load than the bolts located close to the centre. Therefore, for joints having more than two bolts on either side of the building connection with the distance between the first and the last bolt

exceeding 15d in the direction of load, the nominal shear capacity Vns, shall be reduced by the factor,

?lj, given by (Cl.10.3.2.1) ?_lj = 1.075 - l_j / (200 d) but 0.75 < ?_lj < 1.0 where, d= nominal diameter of the bolt Similarly, if the grip length exceeds five times the nominal diameter, the strength is reduced as specified in IS 800. In multi-bolt connections, due to hole mismatch, all the bolts may not carry the same load. However, under ultimate load, due to high bearing ductility of the plates considerable redistribution of the load is possible and so the assumption that all bolts carry equal load may be considered valid.