Weightless Material (γ = 0)

Weightless Material (γ = 0)

A weightless Coulomb material provides another case where Kötter’s equation can be integrated directly. Such an idealization is often used for concrete, cast iron, or other brittle building materials and occasionally for soil such as in the bearing capacity problems when self weight adds only to the resistance and not the driving force causing the collapse.

Forγ=0, Equation (12.14b) reduces to

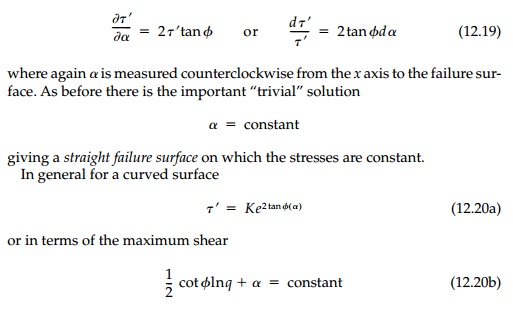
The shape of the curved slip surface on which these stresses act is easily found by considering Figure 12.8 in conjunction with Figure 12.6 where the

slip surface a' is the failure surface on which p =σ'csc Ï• is the stress resultant of the frictional component of strength. For Î³=0, p acts through 0 in the direction of the secondary slip surface at 90 - Ï• to the failure surface Î± from the horizontal. Therefore, in polar coordinates

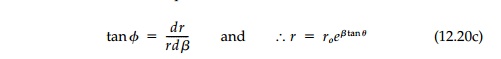
Thus with a frictional component of strength but neglecting weight, the failure surface is made up of straight line and/or log spiral sections combined in some way to satisfy the boundary conditions.