Measures of dispersion - Mean deviation | 11th Business Mathematics and Statistics(EMS) : Chapter 8 : Descriptive statistics and probability
Chapter: 11th Business Mathematics and Statistics(EMS) : Chapter 8 : Descriptive statistics and probability
Mean deviation
Mean deviation
Mean
Deviation (MD) is defined as the average of the absolute difference between the
items in a distribution and the mean or median of that series.
(i) Computation of Mean Deviation - Individual observations
If X 1 , X2 , X 3
,...Xn are n given observation then the mean
deviation about mean or median is as follows

NOTE
If the Mean deviation is computed from Median then in that case D shall denote deviations of the items
from Median, ignoring signs.
(ii) Computation of Mean Deviation - Discrete series
In
discrete series the formula for calculating mean deviation is
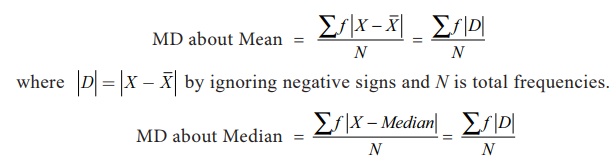
where |D| = |X – Median| by ignoring negative sign and N is total frequencies.
(iii) Calculation of Mean Deviation- Continuous Series
For calculating
Mean deviation in continuous series we have to obtain the midpoints of the
various classes and take the deviations of these mid points from mean or
median.
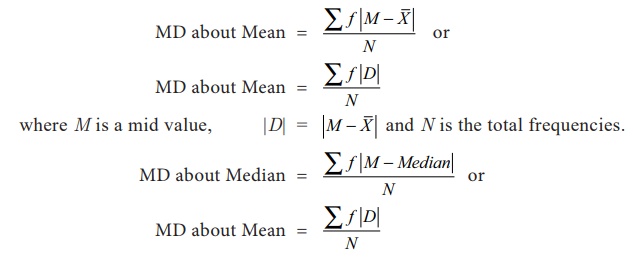
where M is a mid value, |D| = |M–Median| (by
ignoring negative sign) and N is the
total frequencies.
(iv) Relative Measure for Mean Deviation
The
relative measure corresponding to the mean deviation is called the coefficient
of mean deviation and it is obtained as follows
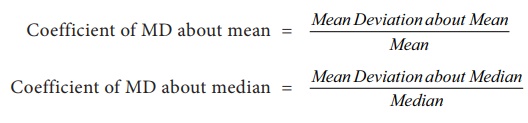
NOTE
However, in practice the arithmetic mean is more frequently used
in calculating the mean deviation. If specifically stated to calculate mean
deviation about median, median can be used.
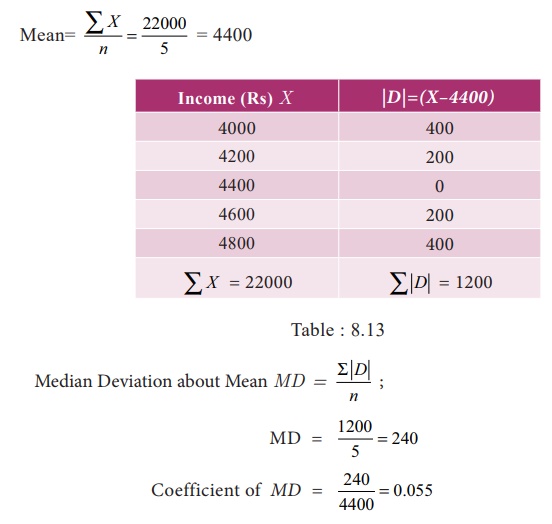
Example 8.19
Calculate
the Mean Deviation about mean and its coefficient of the income groups of five,
given below

Solution:
Calculation
of Mean Deviation about Mean
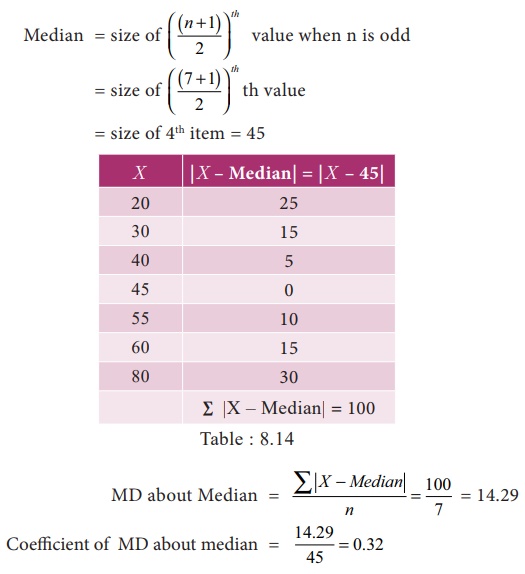
Example 8.20
Calculate
the mean deviation about median and its relative measure for seven numbers
given below: 55, 45, 40, 20, 60, 80, and 30.
Solution:
Arrange
the values in ascending order 20, 30, 40, 45, 55, 60, 80
Example 8.21
Calculate
the Mean deviation about mean for the following data.

Solution:
Calculation
of Mean Deviation about Mean
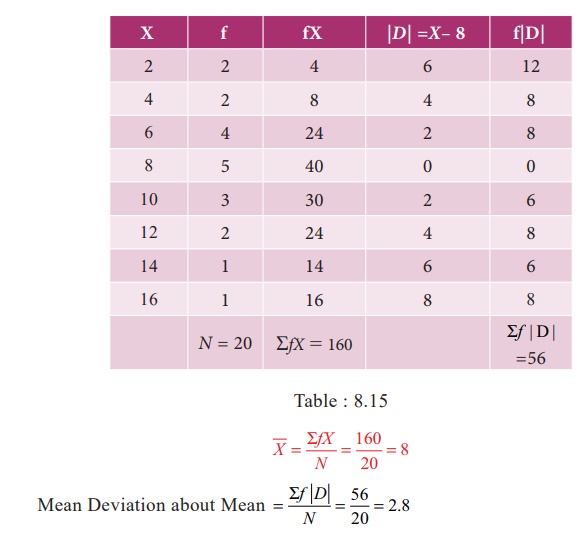
Example 8.22
Calculate
the Mean deviation about median and its relative measure for the following
data.
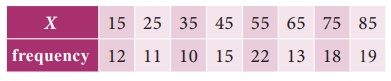
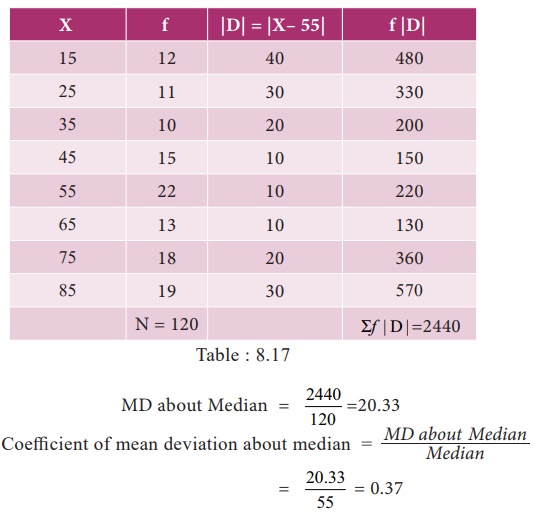
Solution:
Already
the values are arranged in ascending order then Median is obtained by the
following

Mean
deviation about Median
Example 8.23
Find out the coefficient of mean deviation about median in the following series
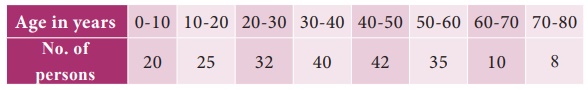
Calculations
have to be made correct to two places of decimals.
Solution:
Calculation
for median follows by the following table

N/2 = 212 / 2 = =106. Class interval corresponding to cumulative frequency 106 is (30 – 40). So, the corresponding values from the median class are L = 30, pcf = 77, f = 40 and c =10.
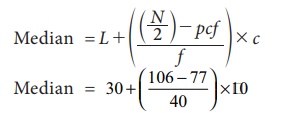
Median =
37.25 (corrected to two places of decimals)
Calculations
proceeded for mean deviation about the median.
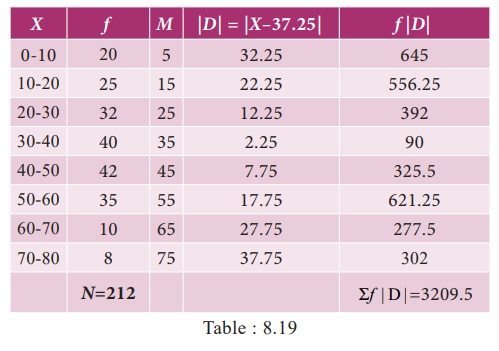
Then the
mean deviation about median is to be computed by the following

NOTE
The above problem can also be solved for mean deviation about
mean instead of median.
Exercise 8.1
1. Find
the first quartile and third quartile for the given observations.
2, 4, 6,
8, 10, 12, 14, 16, 18, 20, 22
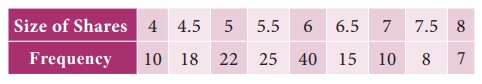
2. Find Q1 , Q3, D8
and P67 of the following
data :
3. Find
lower quartile, upper quartile, 7th decile, 5th decile and 60th percentile for
the following frequency distribution.

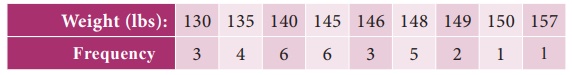
4. Calculate
GM for the following table gives the weight of 31 persons in sample survey.
5. The
price of a commodity increased by 5% from 2004 to 2005 , 8% from 2005 to 2006
and 77% from 2006 to 2007 . Calculate the average increase from 2004 to 2007?
6. An
aeroplane flies, along the four sides of a square at speeds of 100,200,300 and
400 kilometres per hour respectively. What is the average speed of the plane in
its flight around the square.
7. A man
travelled by car for 3 days. He covered 480 km each day. On the first day he
drove for 10 hours at 48 km. an hour. On the second day, he drove for 12 hours
at 40 km an hour and for the last day he drove for 15 hours at 32 km. What is
his average speed?
8. The
monthly incomes of 8 families in rupees in a certain locality are given below.
Calculate the mean, the geometric mean and the harmonic mean and confirm that
the relations among them holds true. Verify their relationships among averages.

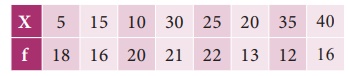
9. Calculate
AM, GM and HM and also verify their relations among them for the following data
10. Calculate
AM, GM and HM from the following data and also find its relationship:

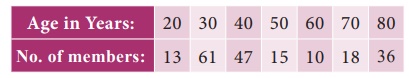
11. Calculate
the quartile deviation and its coefficient from the following data:
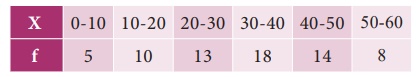
12. Calculate
quartile deviation and its relative measure from the following data:
13. Compute
mean deviation about median from the following data:
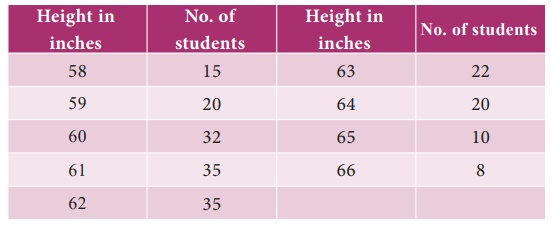
14. Compute
the mean deviation about mean from the following data:

15. Find
out the coefficient of mean deviation about median in the following series
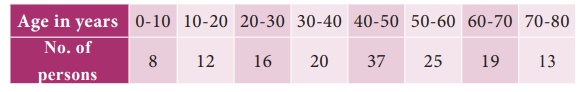
Related Topics