Exercise 3.2: Polynomial Equation in Geometry

EXERCISE 3.2

1. If k is real, discuss the nature of the roots of the polynomial equation 2x2 + kx + k = 0 , in terms of k.

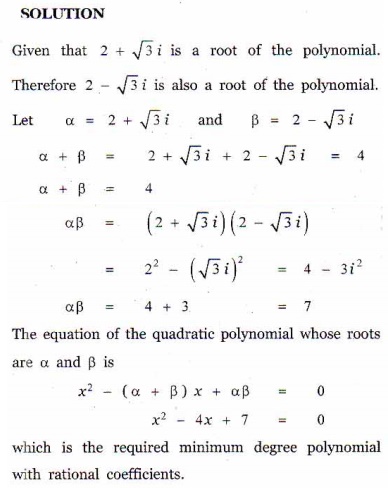
2. Find a polynomial equation of minimum degree with rational coefficients, having 2 + √3i as a root.

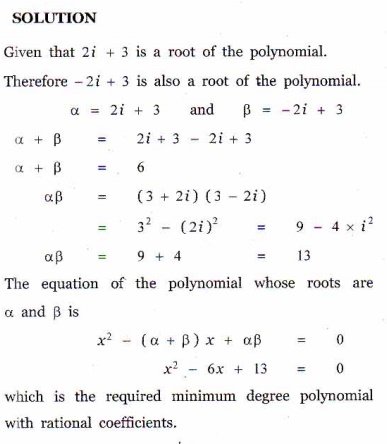
3. Find a polynomial equation of minimum degree with rational coefficients, having 2i + 3 as a root.

4. Find a polynomial equation of minimum degree with rational coefficients, having √5 - √3 as a root.

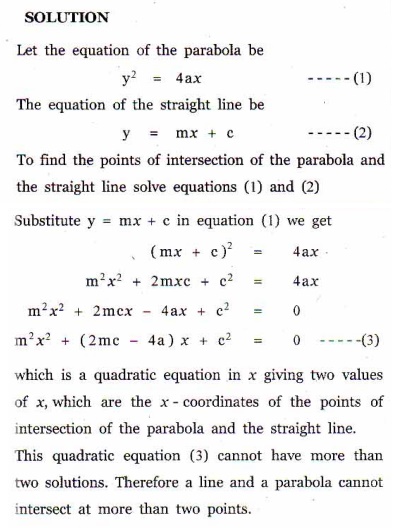
5. Prove that a straight line and parabola cannot intersect at more than two points.

Answers:

1. When k < 0 , the polynomial has real roots.

When k = 0 or k = 8 , the roots are real and equal.

When 0 < k < 8 the roots are imaginary.

When k > 8 the roots are real and distinct.

2. x2 - 4x + 7 = 0

3. x2 - 6x +13 = 0

4. x4 -16x2 + 4