Differential Equations - Summary | 12th Business Maths and Statistics : Chapter 4 : Differential Equations
Chapter: 12th Business Maths and Statistics : Chapter 4 : Differential Equations
Summary
Summary
·
A differential equation is an equation
with a function and one or more of its derivatives.
(i.e) an equation with the function y = f (x)
and its derivatives 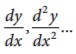 , ... is called differential equation.
, ... is called differential equation.
·
Order of the highest order derivative
present in the differential equation is the order of the differential equation.
·
Degree is the highest power of the highest order
derivative in the differential equation, after the equation has been cleared
from fractions and the radicals as for as the derivatives are concerned.
·
A function which satisfies the given differential
equation is called its solution. The solution which contains as many arbitrary
constants as the order of the differential equation is called a general
solution and the solution free from arbitrary constants is called particular
solution.
·
To form a differential equation from a given
function we differentiate the function successively as many times as the number
of arbitrary constants in the given function and then eliminate the arbitrary
constants.
·
In an equation it is possible to collect all the
terns of x and dx on one side and all the terms of y and dy on the other
side, then the variables are said to be separable. Thus the general form of
such an equation is f (x)dx
= g (y)dy (or) f (x)dx
+ g (y)dy
= 0 By direct integration, we get the
solution.
·
A differential equation which can be expressed in
the form dy/dx = f ( x, y) or dx/dy = g ( x, y) where f ( x, y) and g ( x, y) are homogeneous function of
degree zero is called a homogeneous
differential equation.
·
A differential equation of the form dx/dy + Py = Q where P and Q are constants or
functions of x only is called a first
order linear differential equation.
·
A general second order linear differential equation
with constant coefficients is of the form a
d2y/ dx2 + b dy/dx
+ cy = f ( x)
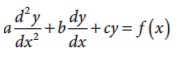
Related Topics