Formation of ordinary differential equation

Formation of ordinary differential equation:

Consider the equation f ( x, y ,c₁ ) = 0 -------(1) where c₁ is the arbitrary constant. We form the differential equation from this equation. For this, differentiate equation (1) with respect to the independent variable occur in the equation.

Eliminate the arbitrary constant c from (1) and its derivative. Then we get the required differential equation.

Suppose we have f ( x, y ,c₁ ,c₂ ) = 0 . Here we have two arbitrary constants c₁ and c₂ . So, find the first two successive derivatives. Eliminate c₁ and c₂ from the given function and the successive derivatives. We get the required differential equation.

Note

The order of the differential equation to be formed is equal to the number of arbitrary constants present in the equation of the family of curves.

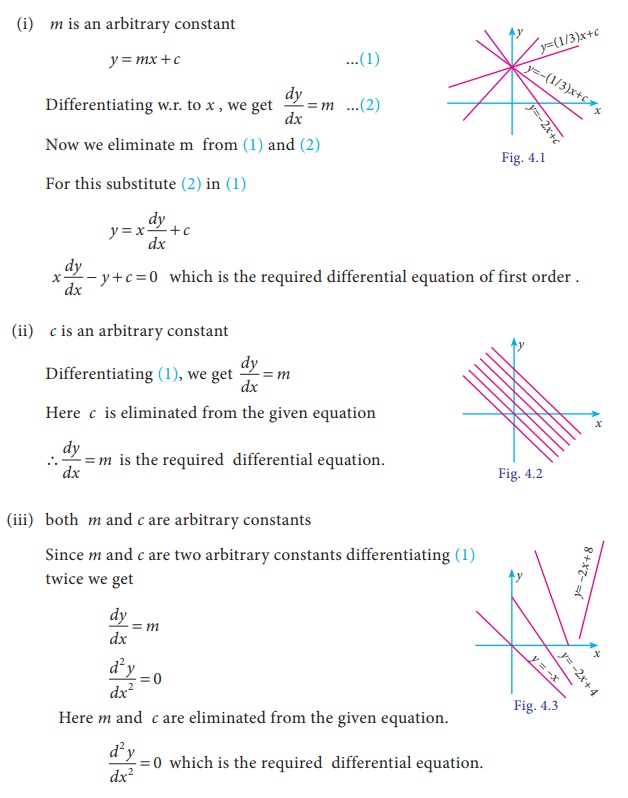
Example 4.2

Find the differential equation of the family of straight lines y=mx+cwhen (i) m is the arbitrary constant (ii) c is the arbitrary constant       (iii) m and c both are arbitrary constants. 

Solution:

Example 4.3

Find the differential equation of the family of curves y= a/x + b where a and b are arbitrary constants.

Solution:

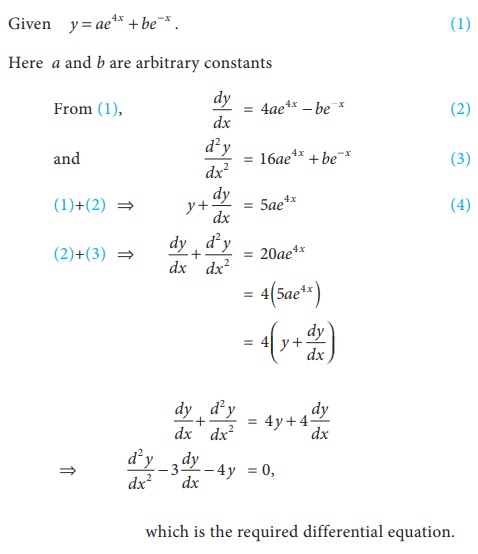
Example 4.4

Find the differential equation corresponding to y = ae^4x + be^−x where a, b are arbitrary constants,

Solution:

Example 4.5

Find the differential equation of the family of curves y = e^x ( a cos x + b sin x) where a and b are arbitrary constants.

Solution :