Introduction

Differential Equations

Introduction

Differential equations, began with Gottfried Wilhelm Leibnitz. He was a Germen Philosopher, mathematicians, and logician.

In lower class, we have studied algebraic equations like 5x − 3( x − 6) = 4x , x² − 7x + 12 = 0, | 18x – 5 | = 3 . The goal here was to solve the equation, which meant to find the value (or values) of the variable that makes the equation true.

For example, x = 9 is the solution to the first equation because only where 9 is substituted for x both sides of the equation are identical.

In general each type of algebraic equation had its own particular method of solution; quadratic equations were solved by one method equations involving absolute values by another, and so on. In each case, an equation was presented, and a certain method was employed to arrive at a solution, a method appropriate or the particular equation at hand.

These same general ideas carry over to differential equations, which are equations involving derivatives. There are different types of differential equations, and each type requires its own particular solution method.

Many problems related to economics, commerce and engineering, when formulated in mathematical forms, lead to differential equations. Many of these problems are complex in nature and very difficult to understand. But when they are described by differential equations, it is easy to analyse them.

A differential equation is an equation with a function and one or more of its derivatives. (i.e) an equation with the function y = f(x) and its derivatives dy/dx,  d²y/dx² ..... is called differential equation.

For example,

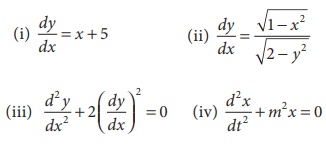
Differential equations are of two types. One is ordinary differential equations and other one partial differential equations. Here we study only ordinary differential equations.