Definition, Formula, Solved Example Problems | Mensuration | Mathematics - Volume of a right circular cone | 10th Mathematics : UNIT 7 : Mensuration
Chapter: 10th Mathematics : UNIT 7 : Mensuration
Volume of a right circular cone
Volume of a right circular cone
Let r and h be the radius and height of a cone then
its volume
V = 1/3 × πr2h cu. units.
Demonstration
·
Consider a right circular cylinder and three right circular cones
of same base radius and height as that of the cylinder.
·
The contents of three cones will exactly occupy the cylinder.
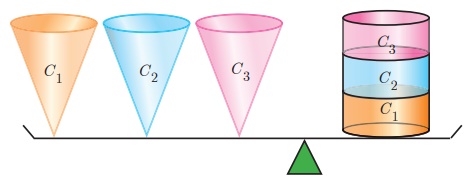
From, Fig.7.28 we see that,
3× (Volume of a cone) = Volume of cylinder
= πr 2h cu. Units
Volume of a cone = 1/3 πr2h cu. Units
Example 7.19
The volume of a solid right circular cone is 11088 cm3. If its
height is
24 cm then find the radius of
the cone.
Solution
Let r and h be the radius and height of the cone respectively.
Given that, volume of the cone = 11088 cm3
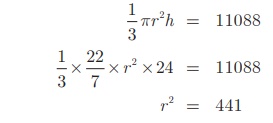
Therefore, radius of the cone r=21 cm
Example 7.20 The ratio of the volumes of two cones is 2:3. Find the ratio of
their radii if the height of second cone is double the height of the first.
Solution Let r1 and h1 be the radius and height of the cone-I and let r2 and h2 be the radius and height of the
cone-II.
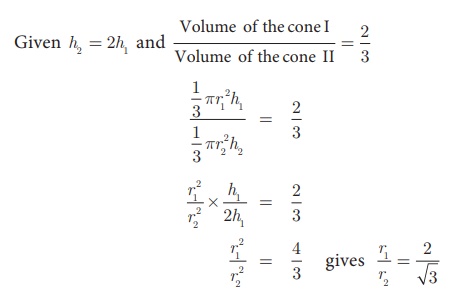
Therefore,
ratio of their radii = 2 : √3
Related Topics