Sources of electromagnetic Waves

Sources of electromagnetic Waves

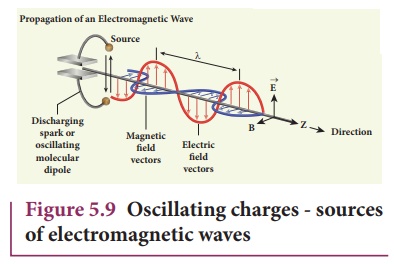
Any stationary source charge produces only electric field (refer Unit 1). When the charge moves with uniform velocity, it produces steady current which gives rise to magnetic field (not time dependent, only space dependent) around the conductor in which charge flows. If the charged particle accelerates, in addition to electric field it also produces magnetic field. Both electric and magnetic fields are time varying fields. Since the electromagnetic waves are transverse waves, the direction of propagation of electromagnetic waves is perpendicular to the plane containing electric and magnetic field vectors.

Any oscillatory motion is also an accelerating motion, so, when the charge oscillates (oscillating molecular dipole) about their mean position as shown in Figure 5.9, it produces electromagnetic waves.

Suppose the electromagnetic field in free space propagates along z direction, and if the electric field vector points along y axis then the magnetic field vector  will be mutually perpendicular to both electric field and the propagation vector direction, which means

E _y = E sin( k z −ωt)

B _x = B sin( k z −ωt)

where, E_o and B_o are amplitude of oscillating electric and magnetic field, k is a wave number, ω is the angular frequency of the wave and  k^ (unit vector, here it is called k propagation vector) denotes the direction of propagation of electromagnetic wave.

Note that both electric field and magnetic field oscillate with a frequency (frequency of electromagnetic wave) which is equal to the frequency of the source (here, oscillating charge is the source for the production of electromagnetic waves). In free space or in vacuum, the ratio between E_o and B_o is equal to the speed of electromagnetic wave, which is equal to speed of light c.

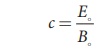
In any medium, the ratio of E_o and B_o is equal to the speed of electromagnetic wave in that medium, mathematically, it can be written as

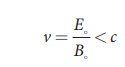
Further, the energy of electromagnetic waves comes from the energy of the oscillating charge.

EXAMPLE 5.3

Compute the speed of the electromagnetic wave in a medium if the amplitude of electric and magnetic fields are 3 × 10⁴ N C^-1 and 2 × 10^-4 T, respectively.

Solution

The amplitude of the electric field, E_o = 3 × 10⁴ N C^-1

The amplitude of the magnetic field, B_o = 2 × 10^-4 T. Therefore, speed of the electromagnetic wave in a medium is

= 3 ×10⁴ / 2 ×10^−4 = 1.5×10⁸ ms^−1