Reduction Formulae

Reduction Formulae

Certain definite integrals can be evaluated by an index-reduction method. In this section, we obtain the values of the following definite integrals:

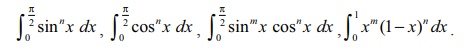
We also obtain the value of the improper integral ^∞∫₀ e^−x xⁿ dx .

The method of obtaining a reduction formula has the following steps:

Step 1 : Identify an index (positive integer) n in the integral.

Step 2 : Put the integral as I_n.

Step 3 : Applying integration by parts, obtain the equation for I_n in terms of I_n−1 or I_n−2.

The resulting equation is called the reduction formula for I_n.

We list below a few reduction formulae without proof:

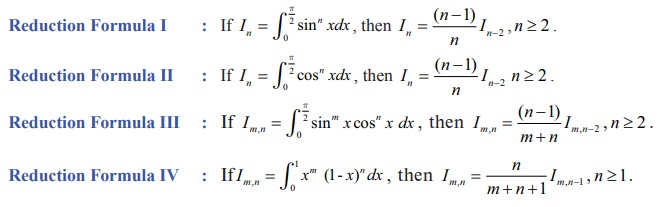
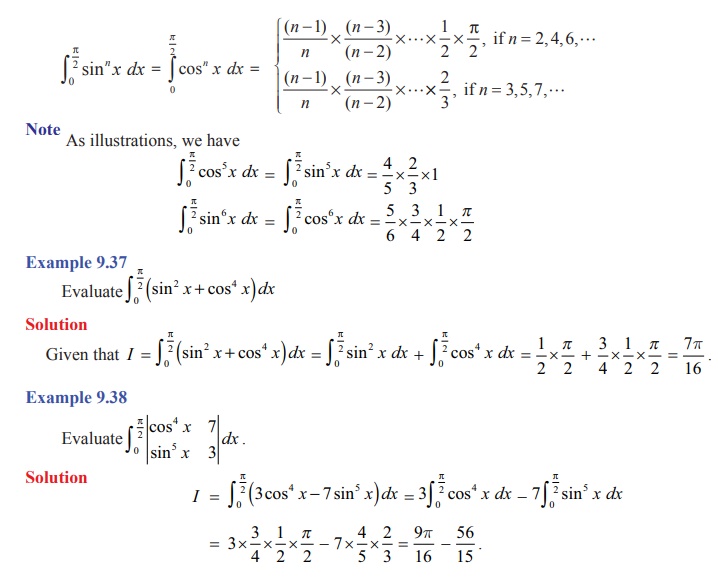
Using the reduction formulas I and II, we obtain the following result (stated without proofs):

By applying the reduction formula III iteratively, we get the following results (stated without proof):

(i) If n is even and m is even,

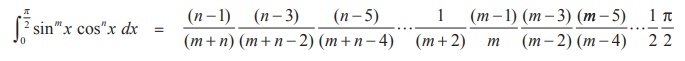
(ii) If n is odd and m is any positive integer (even or odd), then

Note

If one of m and n is odd, then it is convenient to get the power of cos x as odd. For instance, if m is odd and n is even, then

Example 9.39

Find the values of the following:

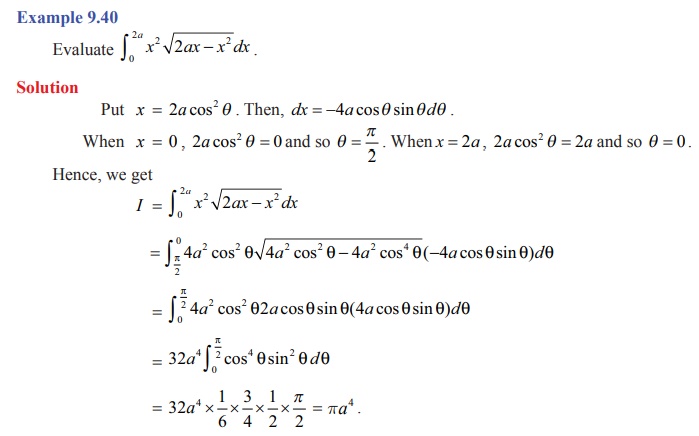
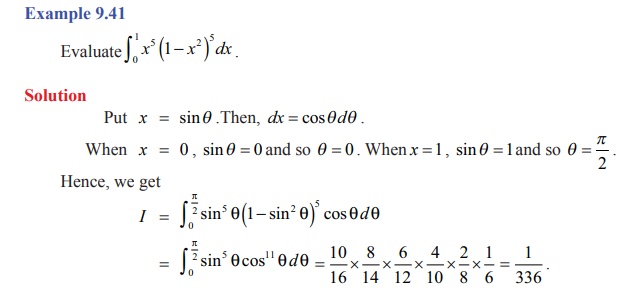
By applying the reduction formula III iteratively, we get the following results (stated without proof):