Exercise 9.9: Volume of a solid obtained by revolving area about an axis

EXERCISE 9.9

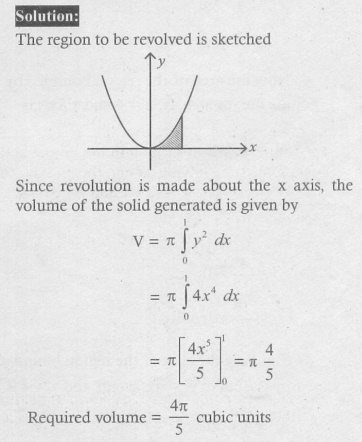
1. Find, by integration, the volume of the solid generated by revolving about the x-axis, the region enclosed by y = 2x2 , y = 0 and x = 1.

2. Find, by integration, the volume of the solid generated by revolving about the x-axis, the region enclosed by y = e−2x y = 0, x = 0 and x = 1

3. Find, by integration, the volume of the solid generated by revolving about the y-axis, the region enclosed by x2 = 1+ y and y = 3 .

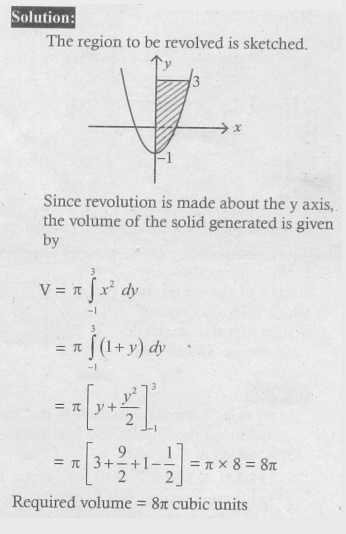
4. The region enclosed between the graphs of y = x and y = x2 is denoted by R, Find the volume generated when R is rotated through 360° about x-axis.

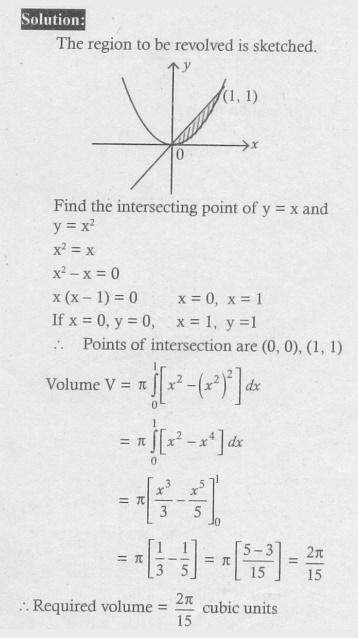
5. Find, by integration, the volume of the container which is in the shape of a right circular conical frustum as shown in the Fig 9.46.

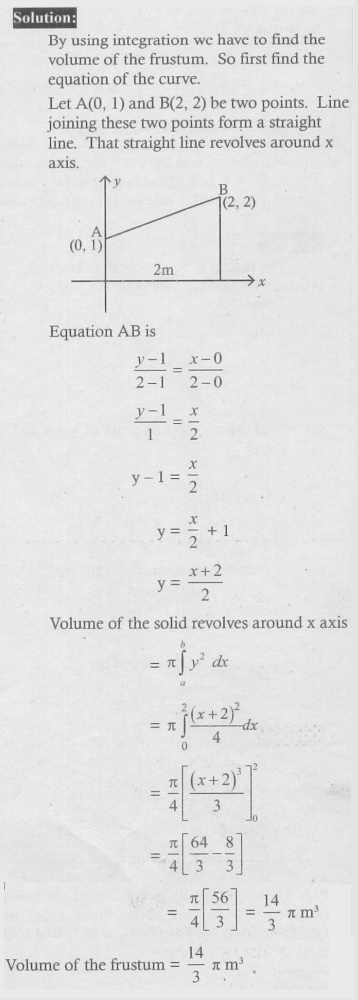
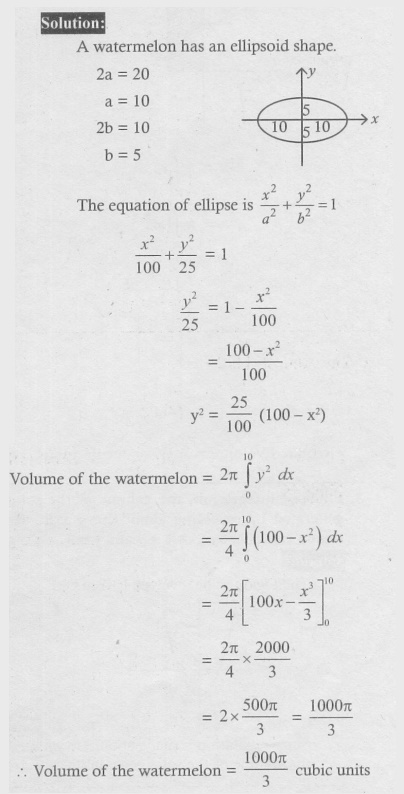
6. A watermelon has an ellipsoid shape which can be obtained by revolving an ellipse with major-axis 20 cm and minor-axis 10 cm about its major-axis. Find its volume using integration.

Answers:

1. 4Ï€ / 5

2. π/4 [1− e−4]

3. 8 π

4. 2Ï€/15

5. 14/3 πm3

6. 1000 πcm3