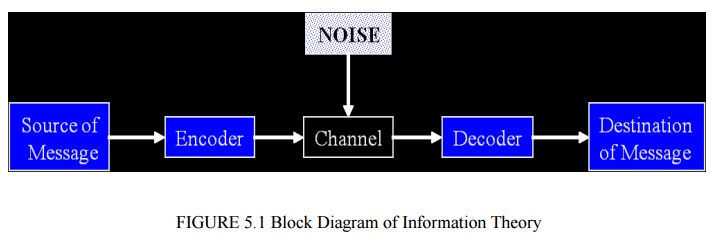
Chapter: Communication Theory : Information Theory
Information Theory
Information Theory
Information
theory is a branch of science that deals with the analysis of a communications
system. We will study digital communications – using a file (or network
protocol) as the channel Claude Shannon Published a landmark paper in 1948 that
was the beginning of the branch of information theory We are interested in
communicating information from a source to a destination In our case, the
messages will be a sequence of binary digits Does anyone know the term for a binary
digit.
One
detail that makes communicating difficult is noise noise introduces uncertainty
Suppose I wish to transmit one bit of information what are all of the
possibilities tx 0, rx 0 - good tx 0, rx 1 - error tx 1, rx 0 - error tx 1, rx
1 - good Two of the cases above have errors – this is where probability fits
into the picture In the case of steganography, the noise may be due to attacks
on the hiding algorithm. Claude Shannon introduced the idea of
self-information.
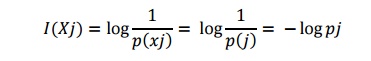
Suppose
we have an event X, where Xi represents a particular outcome of the
Consider
flipping a fair coin, there are two equiprobable outcomes: say X0 = heads, P0 =
1/2, X1 = tails, P1 = 1/2 The amount of self-information for any single result
is 1 bit. In other words, the number of bits required to communicate the result
of the event is 1 bit. When outcomes are equally likely, there is a lot of
information in the result. The higher the likelihood of a particular outcome,
the less information that outcome conveys However, if the coin is biased such
that it lands with heads up 99% of the time, there is not much information
conveyed when we flip the coin and it lands on heads. Suppose we have an event
X, where Xi represents a particular outcome of the event. Consider flipping a
coin, however, let‘s say there are 3 possible outcomes: heads (P = 0.49), tails
(P=0.49), lands on its side (P = 0.02) – (likely much higher than in reality).
Ø Information
There is
no some exact definition, however:Information carries new specific knowledge,
which is definitely new for its recipient; Information is always carried by
some specific carrier in different forms (letters, digits, different specific symbols,
sequences of digits, letters, and symbols , etc.); Information is meaningful
only if the recipient is able to interpret it. According to the Oxford English
Dictionary, the earliest historical meaning of the word information in English
was the act of informing, or giving form or shape to the mind. The English word
was apparently derived by adding the common "noun of action" ending
"-action the information materialized is a message.
Information
is always about something (size of a parameter, occurrence of an event, etc).
Viewed in this manner, information does not have to be accurate; it may be a
truth or a lie. Even a disruptive noise used to inhibit the flow of
communication and create misunderstanding would in this view be a form of
information. However, generally speaking, if the amount of information in the
received message increases, the message is more accurate. Information Theory
How we can measure the amount of information? How we can ensure the correctness
of information? What to do if information gets corrupted by errors? How much
memory does it require to store information? Basic answers to these questions
that formed a solid background of the modern information theory were given by
the great American mathematician, electrical engineer, and computer scientist
Claude E. Shannon in his paper ―A
Mathematical Theory of Communication‖ published in ―The Bell System Technical Journal in October, 1948.
A
noiseless binary channel 0 0 transmits bits without error, What to do if we
have a noisy channel and you want to send information across reliably?
Information Capacity Theorem (Shannon Limit) The information capacity (or
channel capacity) C of a continuous channel with bandwidth BHertz can be
perturbed by additive Gaussian white noise of power spectral density N0/2, C=B
log2(1+P/N0B) bits/sec provided bandwidth B satisfies where P is the average
transmitted power P = Eb Rb ( for an ideal system, Rb= C). Eb is the
transmitted energy per bit, Rb is transmission rate.
Related Topics