Exercise 2.1 (Parts of a Circle)

Exercise 2.1

1. Fill in the blanks:

(i) The ratio between the circumference and diameter of any circle is _______. [Answer: π ]

(ii) A line segment which joins any two points on a circle is a ___________. [Answer: chord]

(iii) The longest chord of a circle is __________.  [Answer: diameter]

(iv) The radius of a circle of diameter 24 cm is _______. [Answer: 12 cm]

(v) part of circumference of a circle is called as _______. [Answer: an arc]

2. Match the following:

(i) Area of a circle - (a) 1/4 πr²

(ii) Circumference of a circle - (b) (π + 2)r

(iii) Area of the sector of a circle - (c) πr²

(iv) Circumference of a semicircle - (d) 2 π r

(v) Area of a quadrant of a circle - (e) θº/360° × πr²

[Answer: (i) − c, (ii) −d (iii) − e, (iv) − b, (v) – a]

(i) Area of a circle c. π r²

(ii) Circumference of a circle d. 2π r

(iii) Area of the sector of a circle e. [θ° / 360°] × π r²

(iv) Circumference of a semicircle b. (π  + 2)r

(v) Area of a quadrant of a circle a. 1/4 πr²

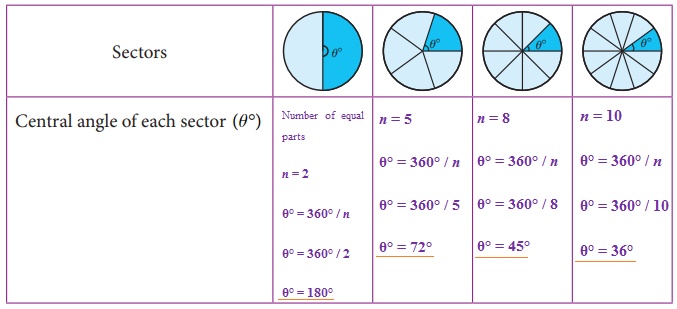
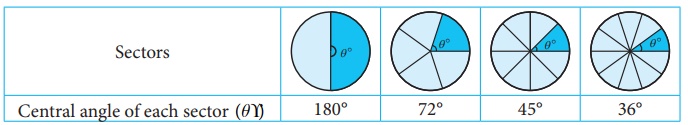
3. Find the central angle of the shaded sectors (each circle is divided into equal sectors).

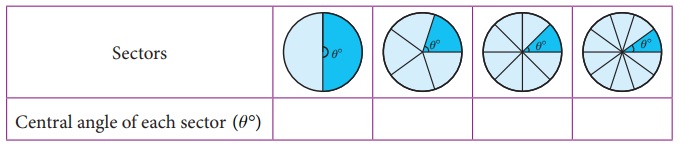
Solution:

4. For the sectors with given measures, find the length of the arc, area and perimeter. (π=3.14)

(i) central angle 45º, r = 16 cm (ii) central angle 120º, d =12.6 cm

Solution:

(i) Central angle 45°, r = 16 cm

Length of the arc l = [ θ° / 360° ] × 2πr units

l = [ 45° / 360° ] × 2 × 3.14 × 16 cm

l = 1/8 × 2 × 3.14 × 16 cm

l = 12.56 cm

Area of the sector = [ θ° / 360° ] × πr² sq.units

A =  [ 45° / 360° ] × 3.14 × 16 × 16

A = 100.48 cm²

Perimeter of the sector P = l + 2r units

P = 12.56 + 2(16) cm

P = 44.56 cm

(ii) central angle 120°, d =12.6 cm

∴ r = 12.6 / 2 cm

r = 6.3 cm

Length of the arc l = [ θ° / 360° ] × 2 πr units

l = [ 120° / 360° ] × 2 × 3.14 × 6.3 cm

l = 13.188 cm

l = 13.19 cm

Area of the sector  A = [ θ° / 360° ] × πr² sq.units

A =  [ 120° / 360° ] × 3.14 × 6.3 × 6.3 cm²

A = 3.14 × 6.3 × 2.1 cm²

A = 41.54 cm²

Perimeter of the sector P = l + 2r cm

P = 13.19 + 2(6.3) cm

P = 13.19 + 12.6 cm

P = 25.79 cm

5. From the measures given below, find the area of the sectors.

(i) length of the arc = 48 m, r = 10 m (ii) length of the arc = 50 cm, r = 13.5 cm

Solution:

(i) Length of the arc = 48 m, r = 10 m

Area of the sector A = lr/2  sq. units

l = 48 m

r = 10 m

= [ 48 × 10 ]/2 m²

= 24 × 10 m²

 = 240 m²

Area of the sector = 240 m²

(ii) Length of the arc = 50 cm, r = 13.5 cm

Length of the arc l  = 50 cm

Radius r = 13.5 cm

Area of the sector A = (lr/2)  sq. units

A = [50 × 13.5] / 2

A = 25 × 13.5 cm

A = 337.5 cm

Area of the sector A = 337.5 cm²

6. Find the central angle of each of the sectors whose measures are given below. (π = 22/ 7)

(i) area = 462 cm², r = 21 cm (ii) length of the arc = 44 m, r = 35 m

Solution:

(i) area = 462 cm², r = 21 cm

Radius of the sector = 21 cm

Area of the sector = 462 cm²

 lr / 2  = 462

  [ l × 21] / 2 = 462

l = [ 462 × 2 ] / 21

l = 22 × 2

Length of the arc l = 44 cm

[ θ° / 360° ] × 2πr = 44 cm

[θ° / 360°] × 2 × [22/7] × 21 = 44 cm

θ° = [ 44 × 360 × 7 ] / [ 2 × 22 × 21 ]

θ° = 120°

∴ Central angle of the sector = 120°.

(ii) length of the arc = 44 m, r = 35 m

Length of the arc = 44 cm

r = 35 cm

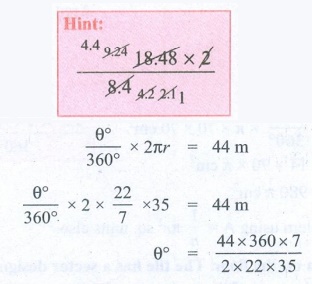
[θ° / 360°] × 2πr = 44 cm

[θ° / 360] × 2 × [22/7] × 35 = 44 cm

θ° = [ 44 × 360 × 7] / [ 2 × 22 × 35]

= 72°

Central angle = 72°

7. A circle of radius 120 m is divided into 8 equal sectors. Find the length of the arc of each of the sectors.

Solution:

Radius of the circle r = 120 m

Number of equal sectors = 8

∴ Central angle of each sector = 360° / n

θ° = 360° / 8

θ° = 45°

Length of the arc l = [ θ° / 360°] × 2πr units

= [45° / 360°] × 2π × 120 m

Length of the arc = 30 × π m

Another method:

l = [ 1/n ] × 2πr = [1/8] × 2 × π × 120 = 30 π m

Length of the arc = 30 π m

8. A circle of radius 70 cm is divided into 5 equal sectors. Find the area of each of the sectors.

Solution:

Radius of the sector r = 70 cm

Number of equal sectors = 5

∴ Central angle of each sector = 360° / n

θ° = 360° / 5

θ° = 72°

Area of the sector = [θ° / 360°] × πr² sq.units

 =  [72° / 360°] × π × 70 × 70 cm²

= 14 × 70 × π  cm²

= 980 π cm²

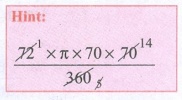
Note : We can solve this problem using A = (1/n) πr² sq. units also.

9. Dhamu fixes a square tile of 30 cm on the floor. The tile has a sector design on it as shown in the figure. Find the area of the sector. (π = 3.14) .

Solution:

Side of the square = 30 cm

∴ Radius of the sector design = 30 cm

Given the design of a circular quadrant.

Area of the quadrant = (1/4)  × πr² sq.units

 = (1/4) × 3.14 × 30 × 30 cm²

= 3.14 × 15 × 15 cm²

∴ Area of the sector design = 706.5 cm² (approximately)

10. A circle is formed with 8 equal granite stones as shown in the figure each of radius 56 cm and whose central angle is 45º. Find the area of each of the granite stones. (π = 22/7)

Solution:

Number of equal sectors ‘n’ = 8

Radius of the sector ‘r’ = 56 cm

Area of each sector = (1/n) πr² sq.units

= (1/8) × (22/7) × 56 × 56  cm² = 1232 cm²

Area of each sector = 1232 cm² (approximately)

Answer:

Exercise 2.1

1. (i) π (ii) chord (iii) diameter (iv) 12 cm (v) circular arc

2. (i) c (ii) d (iii) e (iv) b (v) a

3. 

4. 

5. (i) 240 m² (ii) 337.5 cm²

6. (i) θ = 120º (ii) θ = 72º

7. 30 π m

8. 980π cm²

9. 706.5 cm² (approximately)

10. 1232 cm² (approximately)