Characteristics of the Unit Cell

Characteristics of the Unit Cell

In this section, we discuss some of the important parameters, which are used to describe the crystal structure of the material. A unit cell is characterized by the following parameters.

·        Number of atoms per unit cell.

·        Coordination number

·        Atomic Radius

·        Packing factor (or) packing density

No of atoms per unit cell:

The number of atoms possessed by a unit cell is known as number of atoms per unit cell.

The distribution of atoms is different for different lattice structure. This can be determined if the arrangement of atoms inside the cell is known.

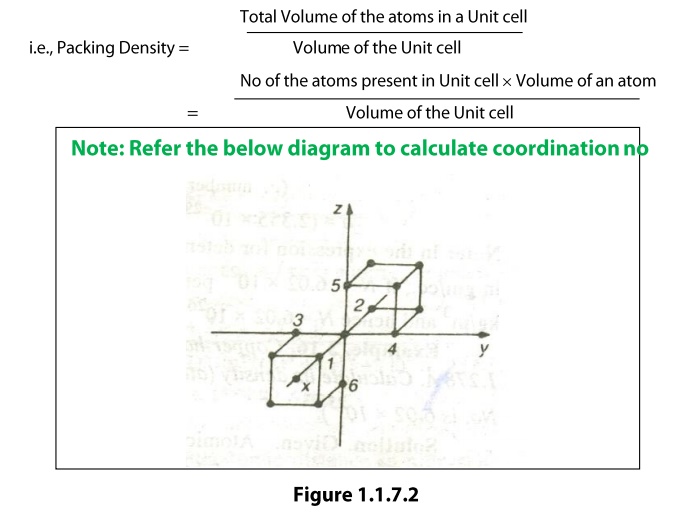
Coordination Number

The coordination number of an atom in a crystal is the number atoms directly surrounding with that atom. If the coordination number is high, then the structure will be more closely packed. It signifies the tightness of packing of atoms in the crystal.

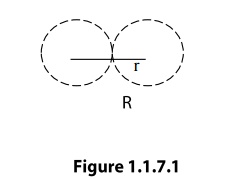
Atomic radius

Atomic radius is defined as half of the distance between any two nearest neighboring atoms, which have direct contact with each other, in a crystal of a pure element. It is usually expressed in terms of cube edge ‘a’(Lattice paramenter).

Packing Factor

The packing density is the ratio between the total volume occupied by the atoms or the molecule in an unit cell and the volume of the unit cell.

A simple cubic (SC) unit cell consists of eight corner atoms as shown in the figure

(i)                Number of atoms per unit cell

In actual crystal, each and every corner atoms is shared by eight adjacent unit cells. Therefore, each and every corner atoms contribute 1/8 of its part to aone unit cell. Hence the total number of atoms present in the unit cells is 1/8 x 8 = 1 atom.

(ii)             Coordination number

The coordination number of a simple cubic unit can be calculated as follows. Let us consider any corner atom; there are four nearest neighbors in its own plane. There is another nearest neighbor in a plane, which lies just above this atom, and another just below this atom. Therefore, the total number of nearest neighbors is six and hence the coordination number is 6.

(iii)           Atomic radius

For a simple cubic unit cell, the atomic radius is given by, r=a/2, where ‘a’ is the side of the unit cell and is equal to the distance between centers of the two nearest atoms.

(iv)           Packing factor

Number of the atoms per unit cell=1

Volume of one atom v = 4/3 πr³

Where r is the atomic radius

Slide of the unit cell, a=2r

Atomic radius r=a/2

Total volume of the unit cell V=a³

We know the packing factor= v/V

Substituting for v and V, we have

Substituting a=2r , we get

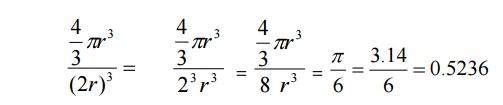
Thus 52% of the volume of the simple cubic unit cell is occupied by atomes and the remaining 48% of the volume of the unit cell is Vacant.

Thus the packing density is 52%. Since the packing density is very low, SC has loosely packed structure.