Chapter: Basic Electrical and electronics : Electric Circuits and Measurements
AC Circuits and KirchhoffŌĆÖs law
AC Circuits
Prerequisites:
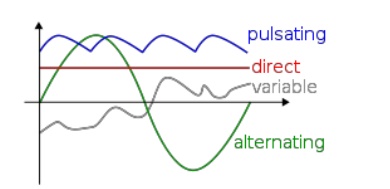
An
alternating current (AC) is an electrical current, where the magnitude of the
current varies in a cyclical form, as opposed to direct current, where the
polarity of the current stays constant.
The usual waveform of an AC circuit is generally
that of a sine wave, as this results in the most efficient transmission of
energy. However in certain applications different waveforms are used, such as
triangular or square waves
Introduction:
Used generically, AC refers to the form in which
electricity is delivered to businesses and residences. However, audio and radio
signals carried on electrical wire are also examples of alternating current. In
these applications, an important goal is often the recovery of information
encoded (or modulated) onto the AC signal.
KirchhoffŌĆÖs law:
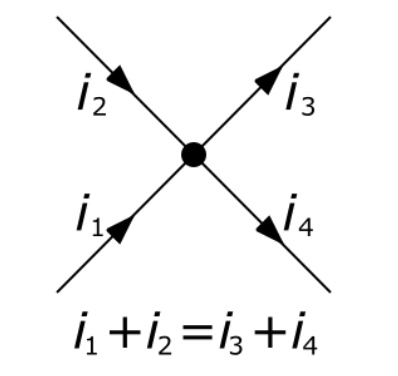
Kirchhoff's Current Law:
First law (Current law or Point
law): Statement:
The sum
of the currents flowing towards any junction in an electric circuit equal to
the sum of currents flowing away from the junction.
Kirchhoff's
Current law can be stated in words as the sum of all currents flowing into a
node is zero. Or conversely, the sum of all currents leaving a node must be
zero. As the image below demonstrates, the sum of currents Ib, Ic,
and Id, must equal the total current in Ia. Current flows
through wires much like water flows through pipes. If you have a definite
amount of water entering a closed pipe system, the amount of water that enters
the system must equal the amount of water that exists the system. The number of
branching pipes does not change the net volume of water (or current in our
case) in the system.
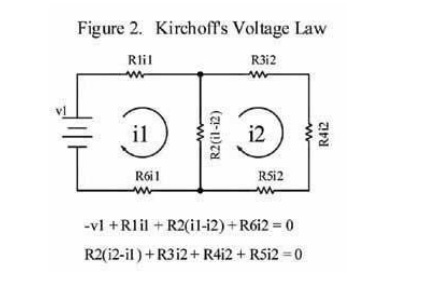
Kirchhoff's Voltage Law:
Second law (voltage law or Mesh law): Statement:
In any
closed circuit or mesh, the algebraic sum of all the electromotive forces and
the voltage drops is equal to zero.
Kirchhoff's
voltage law can be stated in words as the sum of all voltage drops and rises in
a closed loop equals zero. As the image below demonstrates, loop 1 and loop 2
are both closed loops within the circuit. The sum of all voltage drops and
rises around loop 1 equals zero, and the sum of all voltage drops and rises in
loop 2 must also equal zero. A closed loop can be defined as any path in which
the originating point in the loop is also the ending point for the loop. No
matter how the loop is defined or drawn, the sum of the voltages in the loop
must be zero
Related Topics