Water inlet design - Aquaculture Engineering

Water inlet design

Correct design of the inlet flow arrangement to the tank is necessary to ensure even distribution and mixing of the new incoming water and if self-cleaning is to be attained. This requires the inlet water pipe to enter below the water surface in the tank and the water must pass through a narrow nozzle. The force of the inlet water can then be utilized to create a flow pattern inside the tank (Fig. 13.9). It is also important to spread the incoming water throughout the water column.

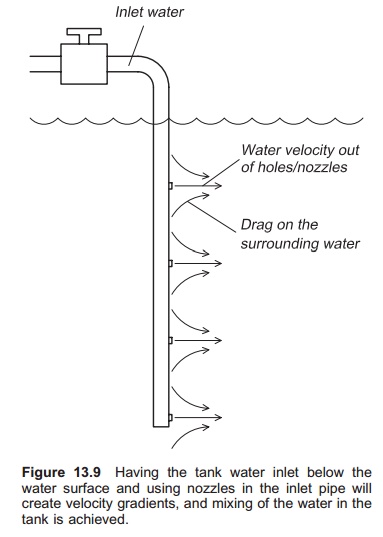
 This can be achieved by the use of several holes, splits or nozzles in the inlet pipe below the water surface. The force of the incoming water will now be distributed throughout the water column, not just in one place. Improved distribution of the new oxygen-rich incoming water is also achieved.

The impulse, as the force caused by the inlet water is called, depends on the water flow and water velocity; it can be expressed as follows for tanks with a circular flow pattern:

F =rQ (v₂− v₁)

where:

F  =impulse

ρ = water density

Q =water flow

v₁=velocity of the water in the tank

v₂=velocity out of the holes in the inlet pipe.

This equation shows that by increasing Q, the impulse will increase. The same result is achieved by increasing the velocity of the water emerging from the holes in the inlet pipe. Decreasing the cross-sectional area of the holes will increase the velocity of the water. However, the increased velocity will increase the turbulence and hence the head loss. Recommended values are below 1.5 m/s in the inlet pipe, while the velocity in the hole (or split or nozzle) should be below 1.2 m/s.⁷

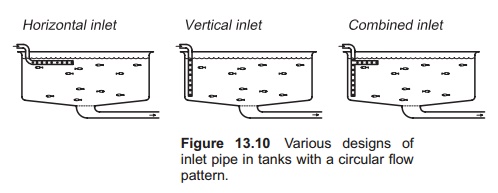
The inlet pipe can be arranged in several ways depending on the tank design (Fig. 13.10). In tanks with a circular flow, a horizontal spray inlet has the advantage of creating good water distribution (primary flow), but the secondary flow is not optimal. The vertical spray inlet creates both good primary and secondary flow, and is therefore preferred. It is also possible to use a combined vertical and horizontal inlet with good results.

Normally a vertical spray inlet will be placed about one fish width away from the tank wall, so that the fish can pass behind, and to avoid too much friction from the tank walls. If it is too close to the wall, friction against the wall will reduce the impulse. However, in a low tank designed with a diameter : depth ratio of less than 0.2, the inlet has to be placed further into the tank closer to the drain, to create a good flow pattern. In silos is it especially difficult to get good inlets and effective transfer of the impulse, and hence effective water exchange throughout the water volume. However it may be possible to use several water inlets in the tanks to improve the flow pattern; testing of the velocity profile is recommended in such cases. Depending on the current velocity from the holes in the inlet pipe, the current velocity in tanks with circular flow is normally in the range 0.15–0.25 m/s.⁷

In raceways it has proved to be difficult to create an inlet that distributes the water in a uniform way throughout the entire cross-sectional area and total length. It is important that the impulse is distributed over as large a part of the cross-sectional area as possible. Because of the continuous reduction in water flow velocity close to the bottom due to friction, there have been experiments in which water was added at several places over the length of the raceway to improve the velocity over the total area; this, however, increases the costs.

The velocity of the inlet water out of the holes or nozzles in the inlet pipe (V₂) depends on the design of the nozzle (hole), the area and the amount of water that has to pass. It can be expressed as follows:

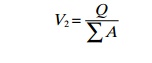
where:

V₂=velocity out of the nozzles

Q =water flow out of the nozzles

ΣA = total cross-sectional area of all the nozzles.

The relation between the water velocity in the inlet pipe and the velocity out of the nozzles will be as follows:

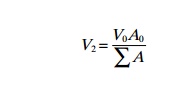
where:

V₀=velocity in the inlet pipe

A₀=area of the inlet pipe.

Example

An inlet pipe to a circular tank is designed for a water flow (Q) of 50 l/min. Suggest an appropriate pipe diameter and area of the nozzles (holes).

First, calculate the area of the inlet pipe:

A = Q/V

Transform the units so that they correspond and take a maximum water velocity of 1.5 m/s.

50 l/min = 0.00083 m³/s

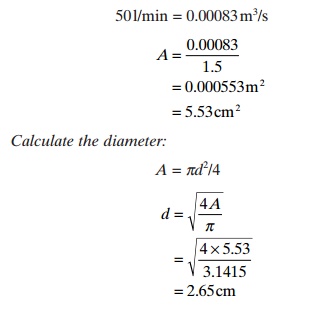
In practice the nearest standard dimension will be used.

Calculate the total nozzle/area (cross-sectional area) using a velocity of 1.2 m/s.

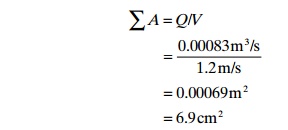
This must then be divided by the number of holes used in the total water column.

To force the water through the inlet pipe results in a head loss. Because of this, a minimum head (available water pressure) is necessary to get the water to flow through the nozzles/holes. Different shapes of the nozzles/holes will result in different head loss because of different degrees of turbu-lence created in the nozzles. This must be taken into consideration when constructing the inlet pipe.

Example

Head loss in the inlet pipe

The inlet pipe has a diameter of 63 mm and a water flow of 300 l/min is used. This gives a water velocity of about 1.6 m/s. Find the head loss when increasing the water velocity from 1 to 2.5 m/s (f = 0.024).

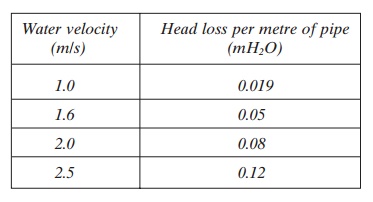
With a water velocity of 2.5 m/s in the inlet pipe, it is necessary to have a pressure in the pipe of 0.1 mH₂O per metre of pipeline to achieve the necessary water flow.