Chapter: Electrical and electronics : Circuit Theory : Transient Response For DC Circuits
Solved Problems: Transient Response For DC Circuits
1. An
alternating current of frequency 60Hz has a maximum value of 12A
1.
Write down value of current for instantaneous
values
2.
Find the value of current after 1∕360 second
3.
Time taken to reach 9.6A for the first time.
In the
above cases assume that time is reckoned as zero when current wave is passing
through zero and increase in positive direction.
Solution:
Given:
F = 60Hz
Im = 12A
W = 2πf = 2π x 60 = 377 rad∕sec
(i). Equation of
instantaneous value is i = Im Sin wt
i = 12 Sin 377t
(ii). t = 1∕360sec
i = 12 Sin (377∕360) = 12 Sin 1.0472 = 10.3924
A
i = 10.3924 A
(iii). i = 9.6 A
9.6 = 12 Sin 377t Sin377t = 0.8 377t = 0.9272
2. A 50 Hz, t = 2.459 x 10-3sec
alternating voltage of 150V (rms) is applied
independently
a.
Resistance of 10Ω
b.
Inductance of 0.2H
c.
Capacitance of 50uF
Find the expression for the instantaneous
current in each case. Draw the phasor diagram in each case.
Solution:
Given ,
F = 50Hz
V = 150 V

Im = Vm∕R = 212.13∕10 = 21.213
ф = 0
For pure resistive current circuit phase
different ф
i = Im Sin wt = Im Sin 2πft
i = 21.213 Sin (100 πt) A
Phasor
diagram: Case (ii):
L = 0.2H
XL = wL =2πfL XL = 2π x
50 x0.2
XL = 62.83
Im = Vm∕XL = 212.13∕62.83 = 3.37A
Ф A = -900 = π∕2 rad
In pure Inductive circuit, I lags V by 90
degree i= Im Sin (wt - ) A
i = 3.37 Sin Ф (wt - Ф) A
i = 3.37 Sin
(100 πt - π∕2) A
Phasor diagram
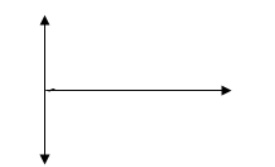
Case(iii):
C= 50uf
Xc = 1∕wC = 1∕2πfC
Xc = 1∕(2π x 50x 50 x 10-6) = 63.66
Im = Vm∕Xc = 212.13∕63.66 = 3.33 A
In pure
capacitive circuit, current leads voltage by 900
= 90o = π∕2 rad
i = Im Sin (wt +ф ) A
i = 3.33 Sin (wt +ф ) A
i = 3.33 Sin (100 πt + π∕2) A
Phasor Diagram:

3. An alternating current i= 414 Sin (2π x 50 x
t) A is passed through a series circuit of a resistance of 100 ohm and an
inductance of 0.31831 H. find the expression for the instantaneous values of
voltage across,
a. The
resistance ,
b. Inductance
c. Capacitance
Solution:

Given
i = 414 Sin (2π x 50 t) A
R = 100 Ω
L = 0.31831 H
XL
= 2π x 50 x 0.31831 = 100 Ω
(i) Voltage across Resistance:
VR
= iR = 1.414 sin (2π x 50 t) x 100
VR
= 141.4 Sin (2π x 50 t) V
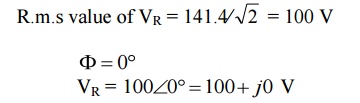
(ii) Voltage across Inductance:
VL = i XL = 1.414 Sin (2π
x 50 t + 900) x 100
VL = 141.4 Sin (2π x 50 t + 900)
V
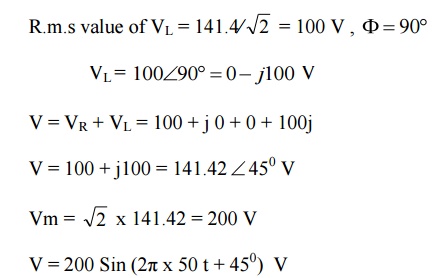
4. The wave form of the voltage and current of a circuit are given by
e= 120 Sin (314 t)
i= 10 Sin (314 t + π∕6)
Calculate the value of resistance, capacitance
which is connected in series to form the circuit. Also, Draw wave forms for
current, voltage and phasor diagram. Calculate power consumed by the circuit.
Solution:
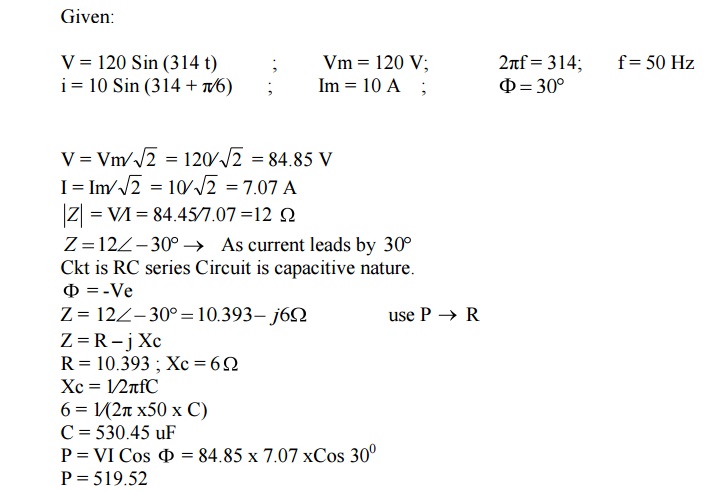
5. A
resistance of 120 Ω and a capacitive reactance of 250Ω are connected in series
across a AC voltage source. If a current 0.9 A is flowing in the circuit find
out,
(i).
Power factor
(ii).
Supply voltage
(iii).
Voltage across resistance and capacitance
(iv).
Active power and reactive power
Solution:
Given :
R = 120Ω
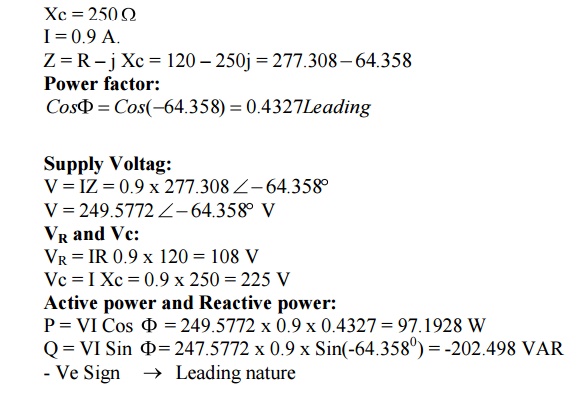
6. A
series circuit consisting of 25 Ω resistor, 64mH inductor and 80uF capacitor to
a 110V, 50Hz, Single phase supply as shown in fig. Calculate the current,
Voltage across individual element and overall p.f of the circuit. Draw a neat
phasor diagram showing
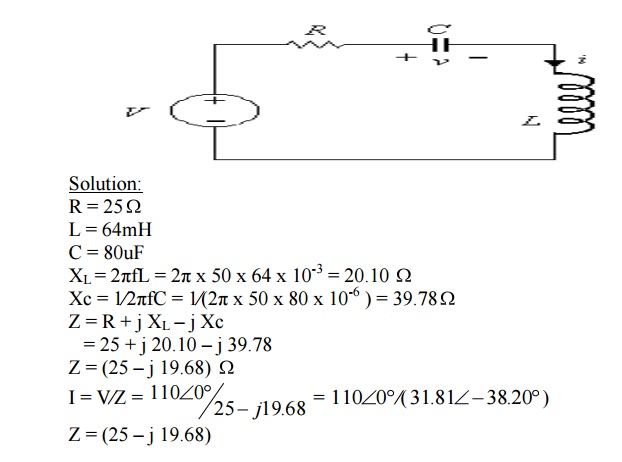

7. A series circuit having pure resistance of
40 , pure inductance of 50.07mH and a capacitance is connected across a 400V,
50Hz Ac supply. This R, L, C combination draws a current of 10A. Calculate
1.
Power factor of circuit
2.
Capacitor value
Solution:
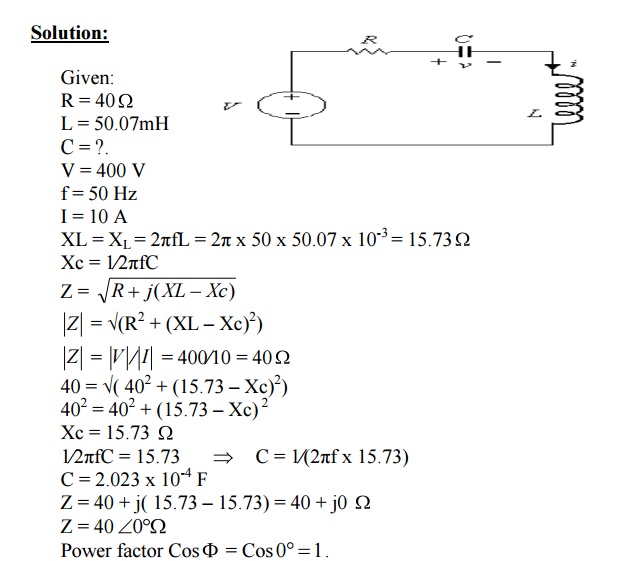
Related Topics