Chapter: Mechanical : Heat and Mass Transfer : Conduction
Solved Problems - Heat and Mass Transfer - Conduction
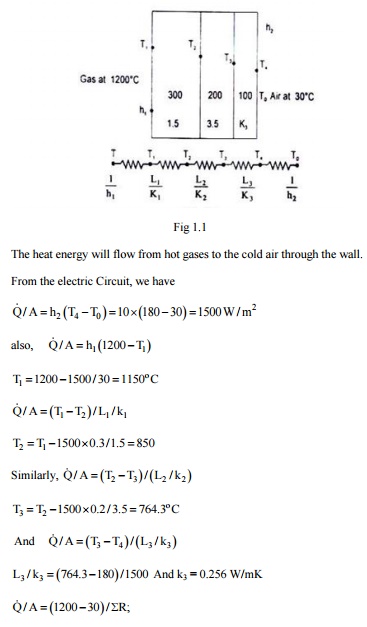
1. A composite wall
consists of three layers of thicknesses 300 mm, 200mm and100mm with thermal
conductivities 1.5, 3.5 and is W/m K respectively. The inside surface is
exposed to gases at 1200°C with convection heat transfer coefficient as 30W/m2K.
The temperature of air on the other side of the wall is 30°C with convective
heat transfer coefficient 10 Wm2K. If the temperature at the outside
surface of the wall is 180°C, calculate the temperature at other surface of the
wall, the rate of heat transfer and the overall heat transfer coefficient.
Solution: The
composite wall and its equivalent thermal circuits is shown in the figure.


2.Derivethe General Heat Conduction
Equation for an Isotropic Solid with
Constant
Thermal Conductivity in Cartesian
coordinates.
Any physical phenomenon is generally accompanied by
a change in space and time of its physical properties. The heat transfer by
conduction in solids can only take place when there is a variation of
temperature, in both space and time. Let us consider a small volume of a solid
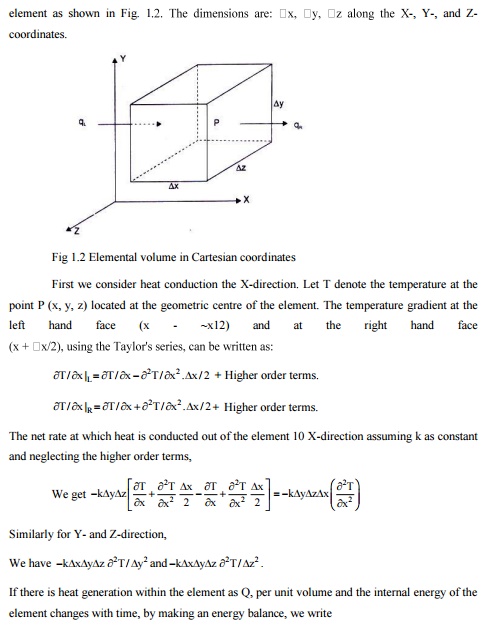
It should be noted that
Fourier law can always be used to compute the rate of heat transfer by
conduction from the knowledge of temperature distribution even for unsteady
condition and with internal heat generation.
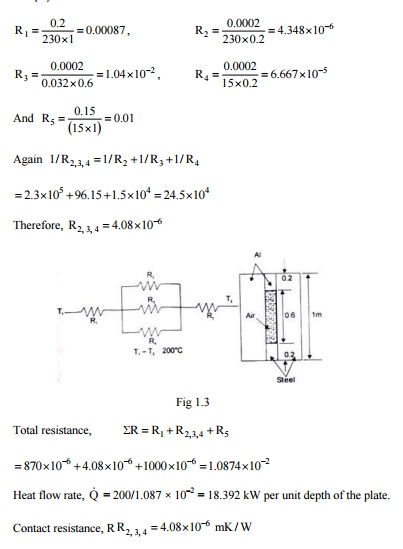
A 20 cm thick slab of aluminums (k = 230
W/mK) is placed in contact with a 15 cm thick stainless
steel plate (k = 15 W/mK). Due to roughness, 40 percent of the area is in
direct contact and the gap (0.0002 m) is filled with air (k = 0.032 W/mK). The
difference in temperature between the two outside surfaces of the plate is
200°C Estimate (i) the heat flow rate, (ii) the contact resistance, and (iii)
the drop in temperature at the interface.
Solution: Let
us assume that out of 40% area m direct contact, half the surface area is occupied
by steel and half is occupied by aluminums.
The physical system and its analogous electric
circuits is shown in Fig. 1.3.
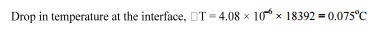
4. A steel pipe. Inside
diameter 100 mm, outside diameter 120 mm (k 50 W/m K) IS Insulated with a40mm
thick hightemperature Insulation(k = 0.09 W/m K) and another Insulation 60 mm
thick (k = 0.07 W/m K). The ambient temperature IS 25°C. The heattransfer
coefficient for the inside and outside surfaces are 550 and 15 W/m2K
respectively. The pipe carries steam at 300oC. Calculate (1) the
rate of heat loss by steam per unit length of the pipe (11) the temperature of
the outside surface .
Solution: A cross-section
of the pipe with two layers of insulation is shown Fig. 1.4with its analogous
electrical circuit.
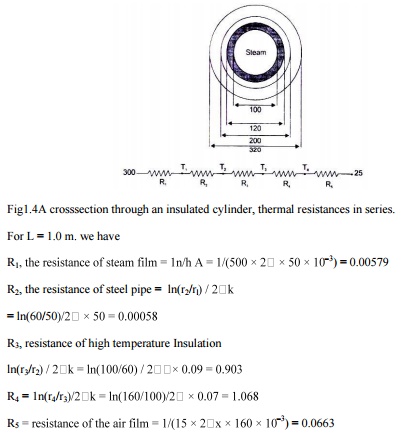
Fig1.4A crosssection through an insulated cylinder,
thermal resistances in series.
For L = 1.0 m.

5. Steel balls 10 mm in
diameter (k = 48 W/mK), (C = 600 J/kgK) are cooled in air at temperature 35°C
from an initial temperature of 750°C. Calculate the time required for the
temperature to drop to 150°C when h = 25 W/m2K and density p = 7800 kg/m3. (AU
2012).
Solution: Characteristic
length, L = VIA = 4/3 pr3/4
pr2 = r/3 = 5 × 10-3/3m
Bi = hL/k = 25 × 5 × 10-3/ (3 × 48) = 8.68 × 10-4<<
0.1,
Since the internal resistance is negligible, we make
use of lumped capacity analysis: Eq. (3.4),
( T -T¥) / ( Ts -T¥)=exp(-Bi
Fo) ; (150 35) / (750 35) = 0.16084
\Bi × Fo = 1827; Fo =
1.827/ (8. 68 × 10-4) 2.1× 103
Or, at/
L2 = k/ ( rCL2)t
= 2100 and t = 568 = 0.158 hour
We can also compute the change in the internal
energy of the object as:
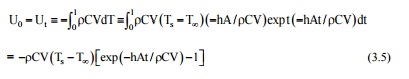
= -7800
× 600 × (4/3) p(5 × 10-3)3
(750-35) (0.16084 - 1)
= 1.47
× 103 J = 1.47 kJ.
If we allow the time't'
to go to infinity, we would have a situation that corresponds to steady state
in the new environment. The change in internal energy will be U0 - U¥ = [ rCV(
Ts -T¥) exp(-
¥)- 1] = [ rCV(
Ts -T¥].
We can also compute the instantaneous heal transfer
rate at any time.
Or. Q = - rVCdT/dt
= - rVCd/dt[ T¥+ ( Ts -T¥
)exp(-hAt/ rCV) ]
= hA( Ts -T¥)[exp(-hAt/ rCV)) and for t = 60s,
Q = 25 × 4 × 3.142 (5 ×
10-3)2(750 35) [exp( -25 × 3 × 60/5 × 10-3 ×
7800 × 600)] = 4.63 W.
6.Aluminums fins 1.5 cm
wide and 10 mm thick are placed on a 2.5 cm diameter tube to dissipate the
heat. The tube surface temperature is 170°C
ambient temperatures is 20°C.
calculate the heat loss per fin. Take h = 130 W/m2 C and K = 200 W/m2
C for aluminums.
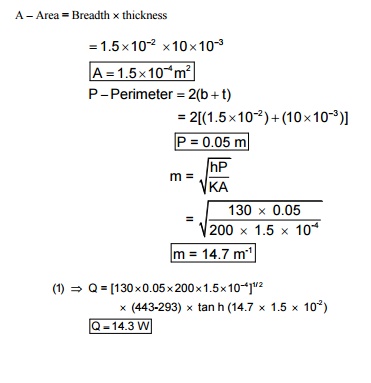
Given
Wide of the fin b = 1.5 cm = 1.5 ´10-2 m
Thickness t = 10 mm = 10 ´10-3 m
Diameter of the tube d = 2.5 cm = 2.5 ´10-2 m
Surface temperature Tb = 170°C + 273 = 443 K
Ambient temperature T¥
= 20°C + 273 = 293 K
Heat transfer co-efficient h = 130 W/m2°C
Thermal conductivity K = 200 W/m°C
Solution
Assume fin end is insulated, so this is short fin
end insulated type problem.
Heat transfer [short fin, end insulated]
Q = (hPKA)1/2 (Tb - T¥) tan h(mL)
……..(1) [FromNoHMT.41] data
book
Where
A –Area = Breadth ´thickness
Related Topics