Definition, Formula, Solved Example Problems, Exercise | Trigonometry | Mathematics - Radian Measure | 11th Mathematics : UNIT 3 : Trigonometry
Chapter: 11th Mathematics : UNIT 3 : Trigonometry
Radian Measure
Radian Measure
Initially right
triangles were used to define trigonometric ratios and angles were measured in
degrees. But right triangles have limitations as they involve only acute
angles. In degrees a full rotation corresponds to 360â—¦ where the choice of 360 dates back thousands of years to the
Babylonians. They might have chosen 360 based on the number of days in a year.
But it does have the nice property of breaking into smaller angles like 30â—¦, 45â—¦, 60â—¦, 90â—¦ and 180â—¦. In 17th century, trigonometry was extended to Physics and
Chemistry where it required trigonometric functions whose domains were sets of
real numbers rather than angles. This was accomplished by using correspondence
between an angle and length of the arc on a unit circle. Such a measure of
angle is termed as radian
measure . For theoretical
applications, the radian is the most common system of angle measurement.
Radians are common unit of measurement in many technical fields, including
calculus. The most important irrational number π plays a vital role in
radian measures of angles. Let us introduce the radian measure of an angle.
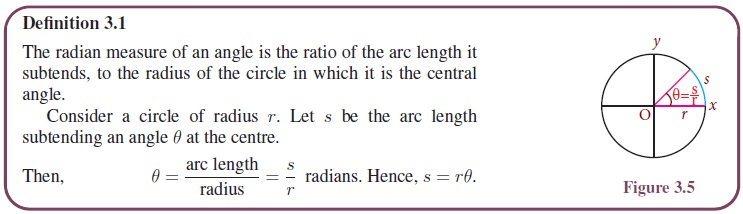
![]() (i) All circles are
similar. Thus, for a given central angle in any circle, the ratio of the
intercepted arc length to the radius is always constant.
(i) All circles are
similar. Thus, for a given central angle in any circle, the ratio of the
intercepted arc length to the radius is always constant.
(ii) When s = r, we
have an angle of 1 radian. Thus, one radian is the angle made at the centre of
a circle by an arc with length equal to the radius of the circle.
(iii) Since the
lengths s and r have same unit, θ is unitless and thus, we do not use any
notation to denote radians.
(iv) θ = 1 radian
measure, if s = r
θ = 2 radian measure,
if s = 2r
Thus, in general θ = k
radian measure, if s = kr.
Hence, radian measure
of an angle tells us how many radius lengths, we need to sweep out along the
circle to subtend the angle θ.
(v) Radian angle
measurement can be related to the edge of the unit circle. In radian system, we
measure an angle by measuring the distance travelled along the edge of the unit
circle to where the terminal side of the angle intercepts the unit circle .
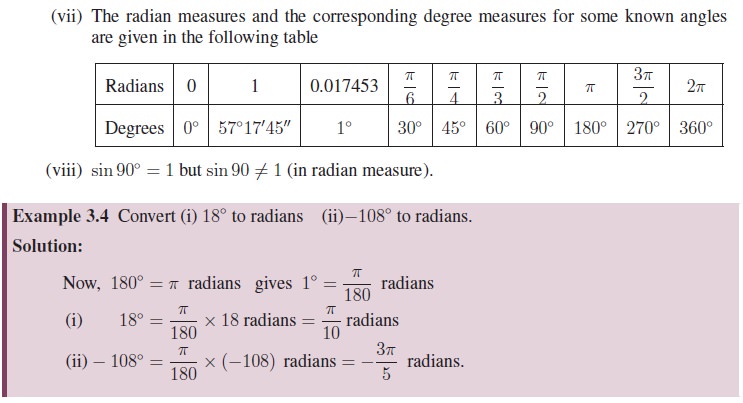
1. Relationship between Degree and Radian Measures
We have degree and
radian units to measure angles. One measuring unit is better than another if it
can be defined in a simpler and more intuitive way. For example, in measuring
temperature, Celsius unit is better than Fahrenheit as Celsius was defined
using 0â—¦ and 100â—¦ for freezing and
boiling points of water. Radian measure is better for conversion and
calculations. Radian measure is more convenient for analysis whereas degree
measure of an angle is more convenient to communicate the concept between
people. Greek Mathematicians observed the relation of π which arises from circumference of a circle and thus, π plays a crucial role in radian measure.
In unit circle, a full
rotation corresponds to 360â—¦ whereas, a full
rotation is related to 2Ď€ radians, the circumference of the unit
circle. Thus, we have the following relations:
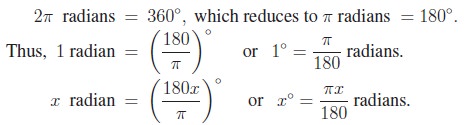
Observe that the scale
used in radians is much smaller than the scale in degrees. The smaller scale
makes the graphs of trigonometric functions more visible and usable. The above
relation gives a way to convert radians into degrees or degrees into radians.


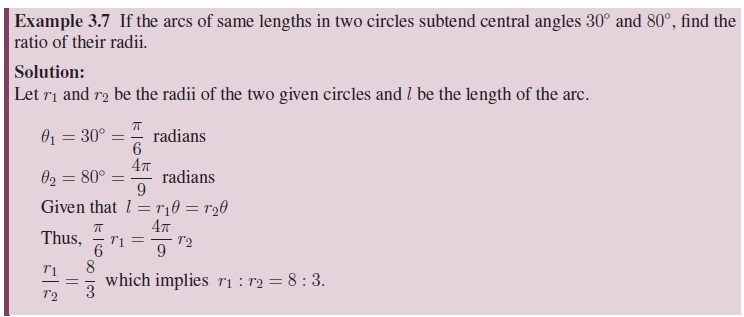

Related Topics