Trigonometry | Mathematics - Properties of Triangle | 11th Mathematics : UNIT 3 : Trigonometry
Chapter: 11th Mathematics : UNIT 3 : Trigonometry
Properties of Triangle
Properties of Triangle
One important use of
trigonometry is to solve practical problems that can be modeled by a triangle.
Determination of all the sides and angles of a triangle is referred as solving
the triangle. In any triangle, the three sides and three angles are called
basic elements of a triangle. Pythagorean theorem plays a vital role in finding
solution of the right triangle. The law of sines and the law of cosines are
important tools that can be used effectively in solving an oblique triangle ( a
triangle with no right angle). In this section, we shall discuss the
relationship between the sides and angles of a triangle and derive the law of
sines and the law of cosines.

Notation: Let ABC be a triangle. The angles of ŌłåABC corresponding to the vertices A, B, C are denoted by A, B, C them selves. The
sides opposite to the angles A, B, C are denoted by a, b, c respectively. Also we use the symbol Ōłå to denote the area of a
triangle.
The circle which
passes through the three vertices of a triangle is called circumcircle of the
triangle. The centre and the radius R of the circumcircle are known as
circumcentre and circumradius respectively.
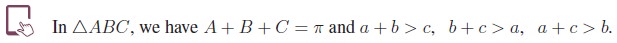
Related Topics