Financial Management - Tutorial Problems and Worked out Problems

Tutorial Problems And Worked Out Problems

1. Simple Interest

Illustration: 1

If you invest Rs 10,000 (P0) in a bank at simple interest of 7% (i) per annum, what will be the amount at the end of three (n) years?

Solution

Future Value, FVn = P0 + SI = P0 + P0(i)(n) = 10,000 + 10,000(0.07)(3) = 12,100

Illustration 2:

2,000 (P0) is deposited in a bank for two (n) years at simple interest of 6% (i). How much will be the balance at the end of 2 years?

Solution

Required balance is given by

FVn = P0 + P0(i)(n) = 2,000 + 2000 (0.06)( 2) = 2,000 + 240 = ` 2,240.

Illustration 3:

Find the rate of interest if the amount owed after 6 (n) months is ` 1,050 (A), borrowed amount being ` 1,000 (P0).

Solution

We know

FVn = P0 + P0(i)(n)

i.e. 1,050 = 1,000 + 1,000(i)(6/12) Or 1,050-1,000 = 500(i) Therefore (i) = 50/500 = 0.10

i.e. (i) = 10%

Compound Interest

Illustration 4: Determine the compound interest for an investment of Rs 7,500 at 6 % compounded half- yearly. Given that (1+i) n for i = 0.03 and n = 12 is 1.42576.

i = 6 / 2×100        = 0 03,

n = 6 × 2 = 12, P = 1,000

Compound Amount = 7,500(1+0.03)12 = 7,500 × 1.42576 = 10,693.20 Compound Interest = 10,693.20 – 7,500 = 3,193.20

Illustration 5: 2,000 is invested at annual rate of interest of 10%. What is the amount after 2 years if the compounding is done?

(a)Annually?

(b) Semi annually?

(c) Monthly?

(d) Daily?

Ans wer:

(a) The annual compounding is given by:

FV2 = P (1 + i) n, n being 2, i being 10/100 = 0.1 and P being 2000

(b) For semiannual compounding n = 2 × 2 = 4, i = 0.1/2 = 0.05

FV4 = 2,000 (1 + 0.05)4 = 2,000 × 1.2155 = 2,431

(c) For monthly compounding, n = 12 × 2 = 24, i = 0.1/12 = 0.00833 FV24 = 2,000 (1.00833)24 = 2,000 × 1.22029 = 2440.58

(d) For daily compounding, n = 365 × 2 = 730, i = 0.1/ (365) = 0.00027 FV730 = 2,000 (1.00027)730 = 2,000 × 1.22135 = 2,442.70

FV4 = 2,000 (1 + 0.05)4 = 2,000 × 1.2155 =2,431

Effective Rate of Interest

It is the actual equivalent annual rate of interest at which an investment grows in value when interest is credited more often than once a year. If interest is paid m times in a year it can be found by calculating:

Ei = (1+ i/m) m -1

Illustration 6: If the interest is 10% payable quarterly, find the effective rate of interest.

Solution

E = (1+ 0.1/4) 4 -1 = 0.1038 or 10.38%

Present Value

Illustration 7: What is the present value of Re. 1 to be received after 2 years compounded annually at 10%?

Solution

Here FV n = 1, i = 0.1

Required Present Value = FV n (1+i) −n

Thus, Re. 0.83 shall grow to Re. 1 after 2 years at 10% compounded annually.

Illustration 8. Future Value of A Series of Payments : -

Calculate the Future value at the end of 5 years of the following series of payment at 10% rate of interest.

R1 = Rs.1000 at the end of 1st year 

R2 = Rs.2000 at the end of 2nd year. 

R3 = Rs.3000 at the end of 3rd year. 

R4 = Rs.2000 at the end of 4st year. 

R5 = Rs. 1500 at the end of 5t h year

Vn  = R1(1+i)n-1 + R2(1+i)n-2 + R3 (1+i)n-3 + R4 (1+i)n-4 + Rn

=   1000(1+.10)5-1 + 2000(1+.10)5-2 + 3000(1+.10)5-3 + 2000(1+.10)5-4 + 1500

=   1000(1.10)4 + 2000(1.10)3 + 3000(1.10)2 + 2000(1.10)1  + 1500

=     1000(1.464)+2000(1.3310)+3000(1.21) +2000(1.10) +1500

= 1464 + 2662 +3630 +2200+1500

Vn = 11456

Another Method: -

Using Compounding Factor Table

Future value of the end of 5 years = 11456

Compound Value of Annuity

Mr. A deposits Rs. 1000 at the end of every year, for a 4 years and the deposits earnscompound interest at 10% per annum. Determine how much money he will have at the end of 4 years.

R ( 1+i) n-1  + (1+i) n-2 + ……… + 1)

1000 ( 1+.10) 4-1 + (1+.10) 4-2 +(1+.10)4-3 + 1) 1000 ( 1+.10) 3 + (1+.10) 2 +(1+.10)1 + 1) 1000 ( 1.3310+1.2100+1.1000+ 1) 1000(4.6410)

=4641

(OR)

a)     Using compounding Factor table Vn = ® (ACFin)

=     1000 x 4.641

=     4641

Compound Value of Annuity Due :

1.     Mr. X deposits Rs. 5000 at beginning of each year of 5 years in a bank and the deposit earns a compound interest at 8% per annum. Determine how many money be will have at the end of 5 years.

=(R )(( 1+i) n  + (1+i) n-1 + ……… + (1+i)1)

= 5000[(1+.08)5 + (1+.08)5-1+ (1+.08)5-2+(1+.08)5-3 +(1+.08)1]

= 5000[(1+.08)5 + (1+.08)4+ (1+.08)3+ (1+.08)2 +(1+.08)1]

= 5000[(1.0800)5+ (1.0800)4+ (1.0800)3+(1.0800)2+(1.0800)1]

= 5000(1.4693+1.3605+1.2597+1.664+1.0800)

= 5000(6.3359) = 31679.5

b) Using compounding factor table

Vn= ® (ACFin) (1+P)

= 5000x5.867 (1+.08) = 5000 x 5.867 (1.08) = 31681

B. Discount (or) present value technique: -

You have an opportunity to buy a debenture today and you will get back Rs, 1000 after one year. What will you be willing to pay for the debenture today if your time performance for money is 10% per annum?

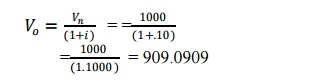
Present value Vo = Future value (Vn) x DFin

= 1000 x 0.90909

= 909.0900

Present Value Series of Payment

Calculate present value of the following cash flows assuming a discount rate of 10%

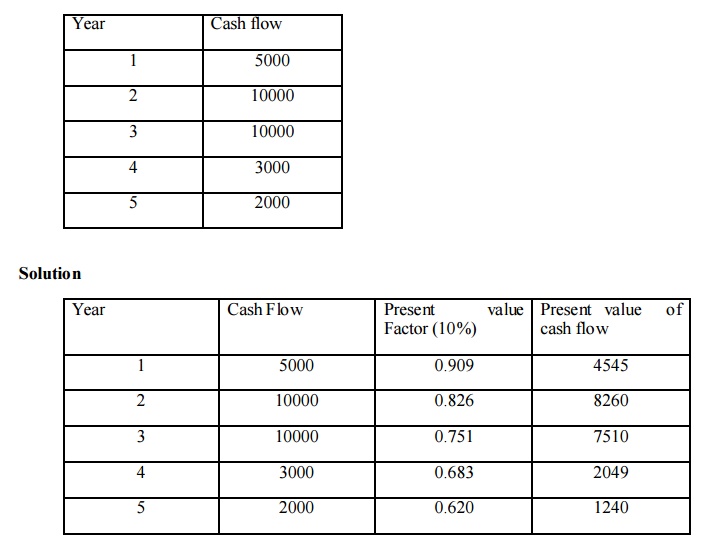
Total present value of cash flow = 23604

Present Value of an Annuity

1. Mr. X has to receive Rs. 2000 per year for 5 years. Calculate present value of equity assuming that he can earn interest on his investment at10% P.A.

Vo = (R) (ADFin)

= 2000 x 3.791

= 7582

Present Value of an Annuity Due:-

1. Mr. A has to receive Rs. 1000 at the beginning of each year for 5 years. Calculate the present value of an annuity due assuming 10% rate of interest.

Vo = (1000) (3.791) (1+-10)

= 1000 x 3.791 (1.10)

= 4170

Present Value of an Annuity Growing at Constant Rates

1. Mr. X has rented out a portion of his house for 4 years at an annual rent Rs. 6000 units with the stipulation that rent will increase by 10% every year. If the required rate of return is 15%. What is the present value of the expected series of Rent?

a)  Calculate  the series of payment of rent

Valuation Of Bonds

ILLUSTRATION

Let us assume the face value of the bond is $1,000 (maturity value $1,000). The bond has a 10% coupon rate payable semi-annually and the yield to maturity (return) is 9%. The bond matures in 5 years period from now. What is the value of the bond?

Interest 1 till 10 = $50 per semi-annual period. ($100 annually) n=10 because 5 years x 2 payments per period.

Yield to maturity = 9%, therefore, semi-annual YTM (return) =9/2 = 4.5% or 0.045 Solving for the above equation, we get

Bond price = $1,040 (rounded)

Payback period

Illuatration

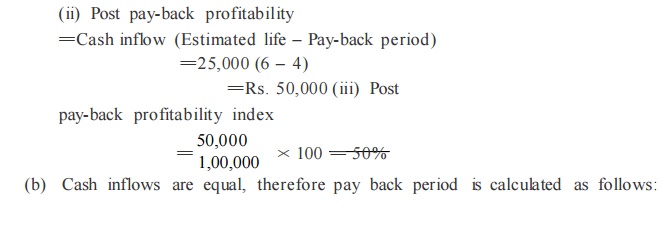
If the cash flows are equivalent, how the payback period is to be calculated?

The cost of the project is Rs.1,00,000. The annual earnings of the project is Rs.20,000. Calculate the payback period.

Payback period = Average Annual Earnings / Initial Investment = 1,00,000 / 20,000 = 5 years

It is obviously understood that, Rs.20,000 of annual earnings (cash inflows) requires 5 years time period to get back the original volume of the investment.

Illuatration

If the cash flows are not equivalent, how the payback period is to be calculated?

The cost of the project  is Rs.1,00,000. The annual earnings of the project are as

follows

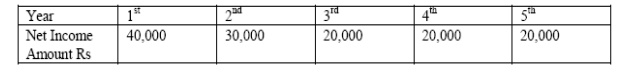
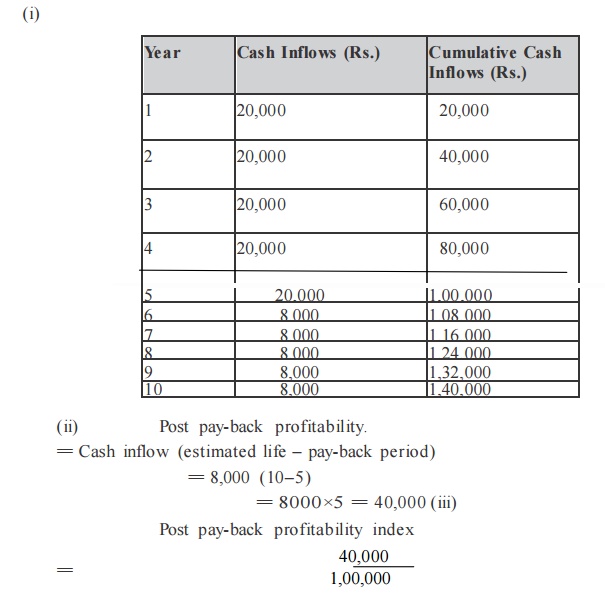
·        The ultimate aim of determining the cumulative cash inflows to find out how many number o f years taken by the firm to recover the initial investment.

The next step under this method is to determine the cumulative cash flows

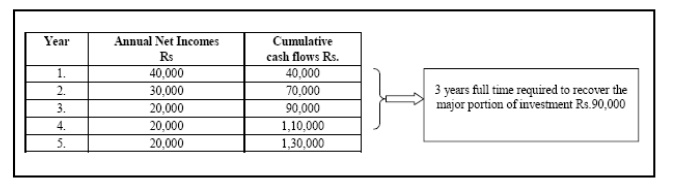
The uncollected portion of the investment is Rs,10,000. This Rs.10,000 is collected from the 4th year Net income / cash inflows of the enterprise. During the 4th year the total earnings amounted Rs.20,000 but the amount required to recover is only Rs.10,000. For earning Rs.20,000 one full year is required but the amount required to collect it back is amounted Rs.10,000. How many months the firm may require to collect Rs.10,000 out of the entire earnings Rs.20,000.

v        Payback period consists of two different components

v   Payback period for the major portion of the investment collection in full course - E.g.: 3 years

v   Payback period for the left /uncollected portion of the investment

v   For the second category 0.5 years

v   = 10000/20000 = 0.5 years

Total payback period= 3 Years +.5 year = 3.5 years

Illustration

A project costs Rs.2,00,000 and yields and an annual cash inflow of Rs.40,000 for 7 years. Calculate payback period

First step is identify the nature of the annual cash inflows

In this problem, the annual cash inflows are equivalent throughout life period of the

project

Pay Back Period = 2,00,000/ 40,000 = 5years

Illustration

Calculate the payback period for a project which requires a cash outlay of Rs.20,000 and generates cash inflows of Rs 4,000 Rs.8,000 Rs. 6,000 and Rs. 4,000 in the first, second, third, and fourth year respectively

First step is to identify the nature of the cash inflows The cash inflows are not equivalent/constant

v   Cost of the project is to be recovered Rs.20,000. The project takes 3 full year‘s time period to recover the major portion of the initial investment which amounted Rs.18,000 out of Rs.20,000

v   The remaining amount of the initial investment is recovered only during the fourth year.

v   The left portion Rs.2,000 has to be recovered only from the fourth year cash inflows of Rs.4,000.

v   Pay Back Period = Pay Back period of the major portion + Pa y Back period of the remaining portion

v   Pay Back period of the major portion = 3 years

Illustration  A  project  cost  Rs.       500000  and  yield  annually  a  profit  Rs.  80000,  after depreciation at 12% per annum but before tax 50%.     Calculate payback period. 

Profit before tax   =       80000         

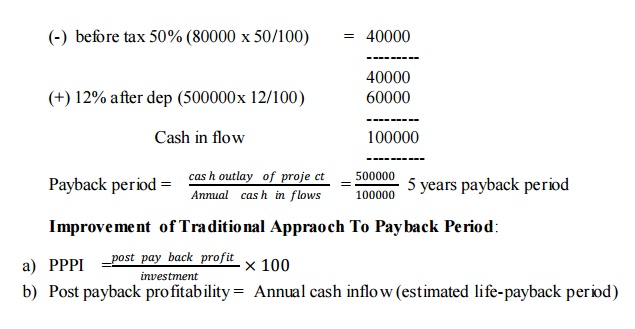
Illustration

Calculate post payback profitability index. Initial outlay Rs. 50000, Annual cash flow(after tax before depreciation) Rs. 10000, Estimated life 8 years.

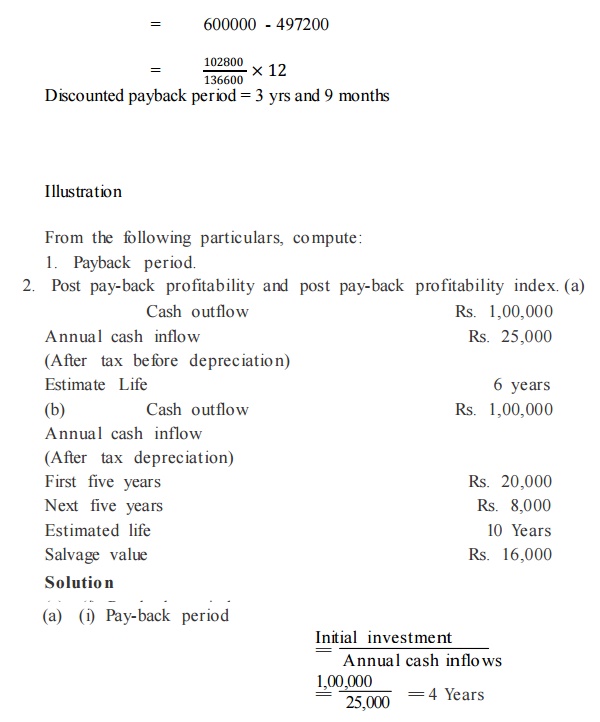
Discounted payback period

Illustration

Calculate discounted payback period from the information given below.

Cost of project = Rs. 600000

Life of project = 5 years

Annual cash inflows Rs. 200000 for each year

Cutoff rate 10%

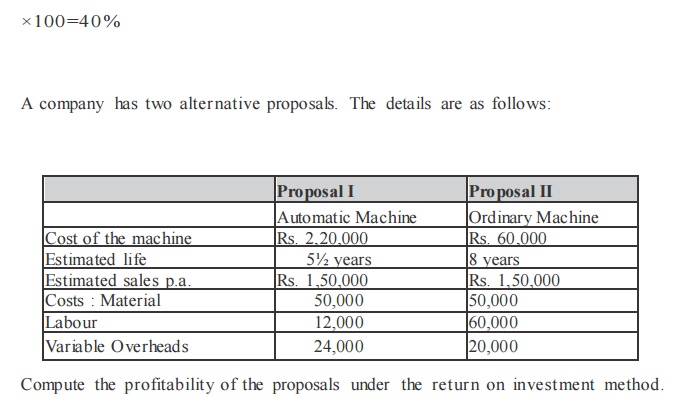
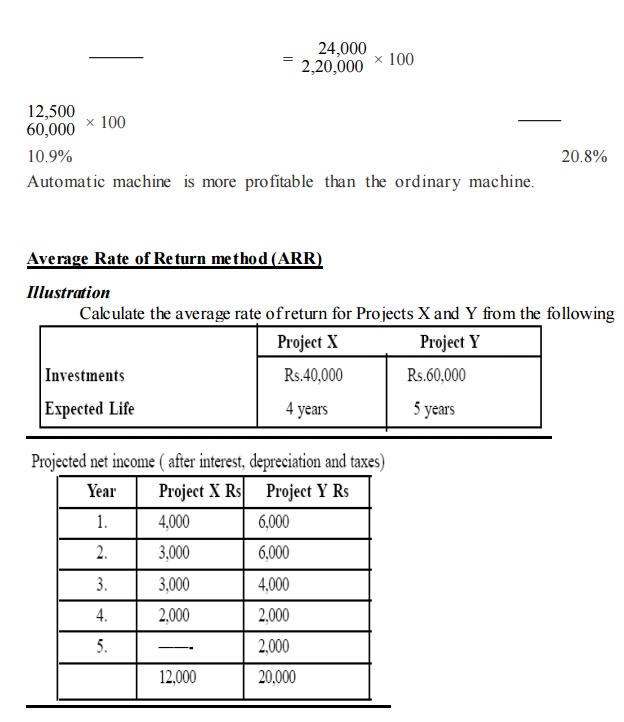
v   If the required rate of return is 10% which project should be undertaken?

v   Average Rate of Return = Original Investment / Average Annual Income X100

v   The first step is to find out the average annual income of the two different projects X and Y

v   Average Annual Income Total income throughout the Project / Life of the Project

v   Average Annual Income (Project X) = Rs. 12,000 / 4 years = Rs. 3,000

v   Average Annual Income (Project Y) = Rs. 20,000 / 5 years = Rs. 4,000

v   The next step is to find out the Average rate of return:

v   Average rate of return (Project X) = Rs. 3,000 / Rs.40,000 X100 =7.5%

v   Average rate of return (Project Y) = Rs.5,000/ Rs. 60,000 X 100 = 8.33%

v   Both the projects are lesser than the given required rate of return. These two projects are not advisable to invest only due to lesser accounting rate of return.

Net present value method(NPV) 

Illustration

Calculate the NPV of 2 projects and suggest which of 2 projects should be accepted assuming a discount rate 10%

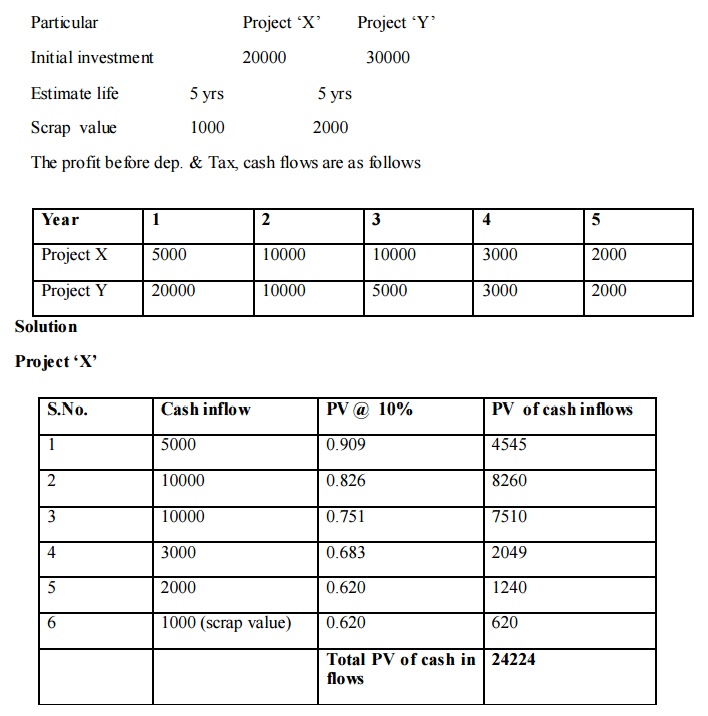
NPV = PV cash of inflow - PV of cash outflows = 24224 - 20000 NPV = 4224

Project Y

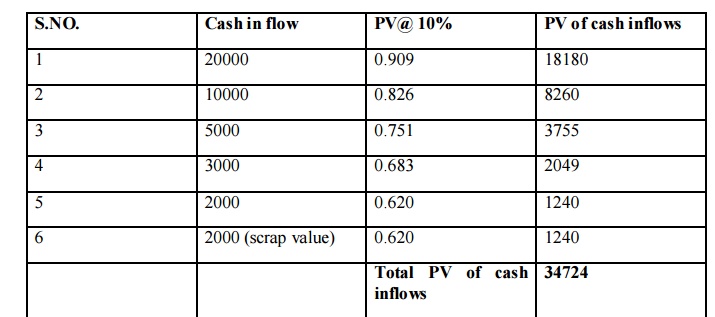
NPV = PV of cash inflow  - PV of cash outflows

NPV = 34724 – 30000       

NPV = 4724    

Comment:       

NPV of project y is higher than the NPV of project x. Hence, it is suggested that project y should be selected.

Illustration

A company is considering investment in a project that cost Rs. 2 lakh the project that cost Rs. 2 lakh the project has an expected life of 5 years and zero solvage (scrap) value. The company uses straight line method of depreciation (40,000),Tax Rate is 40%

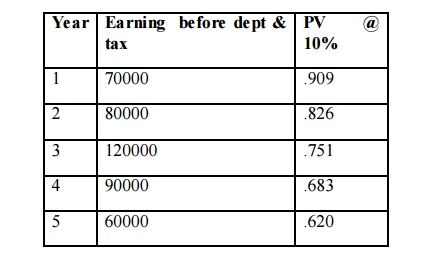
You are require to calculate the Net present value @ 10 % and advice the company

Solution

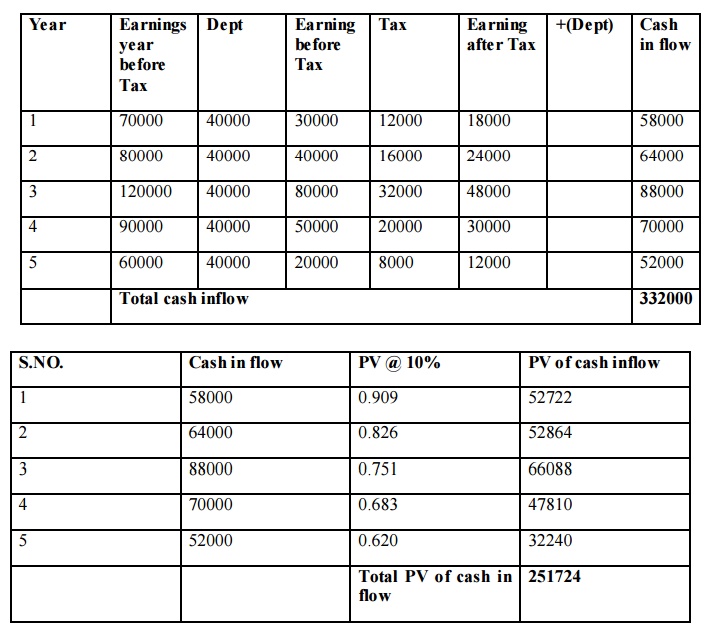
NPV = PV of cash inflow – PV of cash outflow

= 251724 – 200000

=       51724

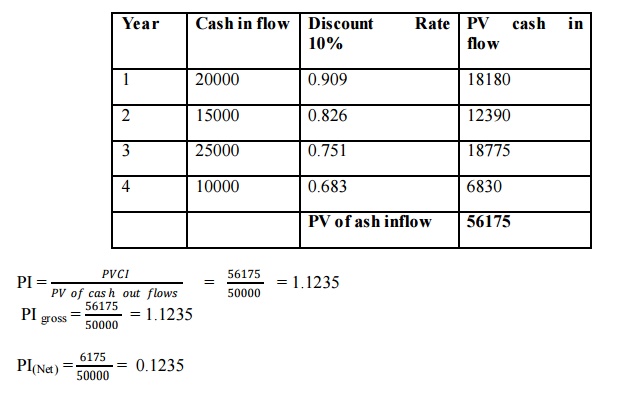
Profitability Index Method (or) Benefit cost Ratio: -

Initial cash outlay of a project Rs. 50000, cash flows 20000, 15000, 25000, 10000 for 4 years Rate of Discount 10% calculate PI

Illustration

Inte rnal Rate of Return Method (IRR)

Illustration

1) Initial outlay Rs. 50000, life of an asset 5 years Annual cash flow RS. 12500, calculate IRR

Illustration

Initial outlay Rs. 50000, life of an asset 5 years Annual cash flow Rs. 12500, Calculate IRR

Present value of annuity table 8 % approximately

IRR = 8 %

Illustration

When the annual cash flows over the life of the asset.

Initial investment Rs. 60000, Life of the Assets 4 years

1st year - 15000

2nd year – 20000

3rd year – 30000

4th year -20000 Calculate the IRR

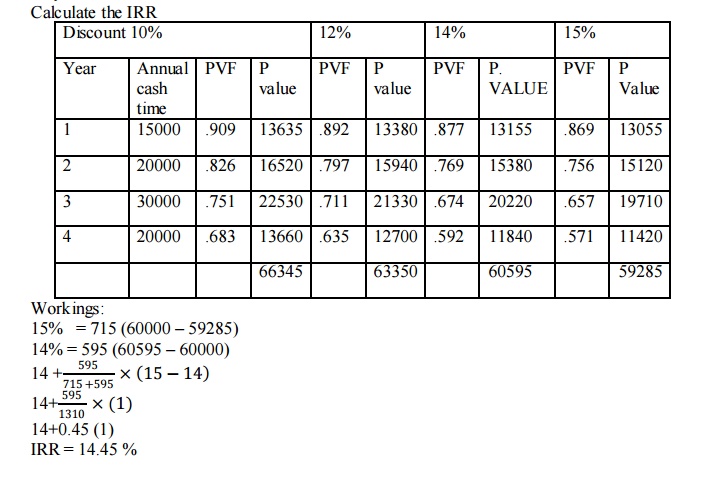
Dividend Price Approach

Illustration

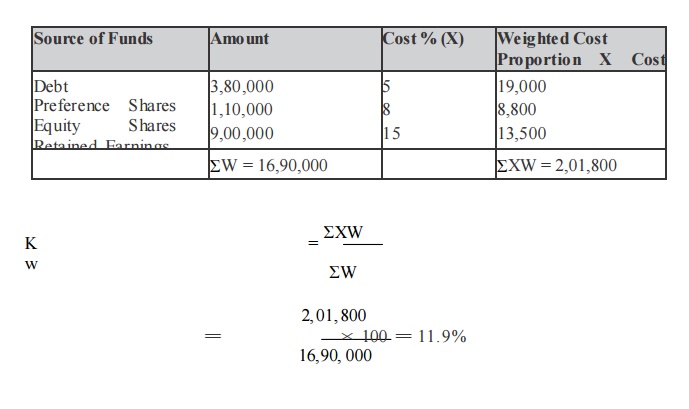
A company has on its books the following a mounts and specific costs of each type of capital.

Solution

A. Book value 

Computation of Weighted Average Cost of Capital

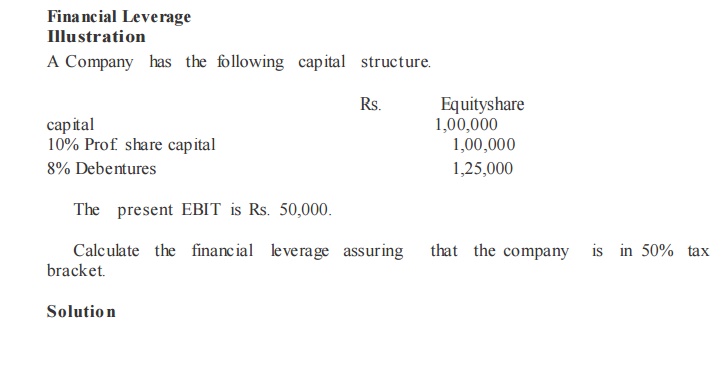
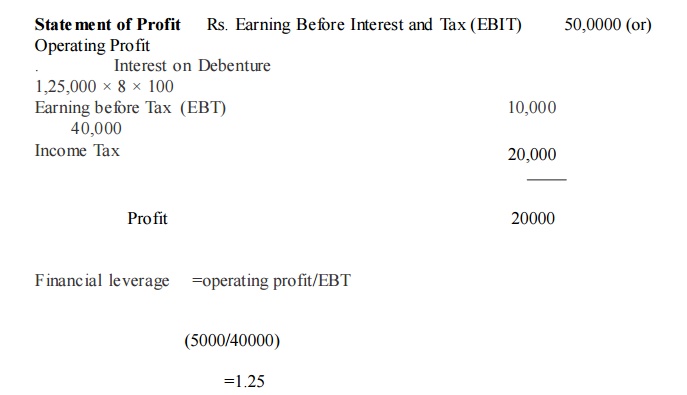
Ope rating Leverage

Illustration

From the following selected operating data, determine the degree of operating leverage. Which company has the greater a mo unt of business risk? Why?

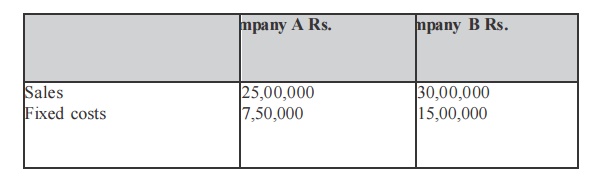
Variable expenses as a percentage of sales are 50% for company A and 25% for company B.

Solution

Statement of Profit

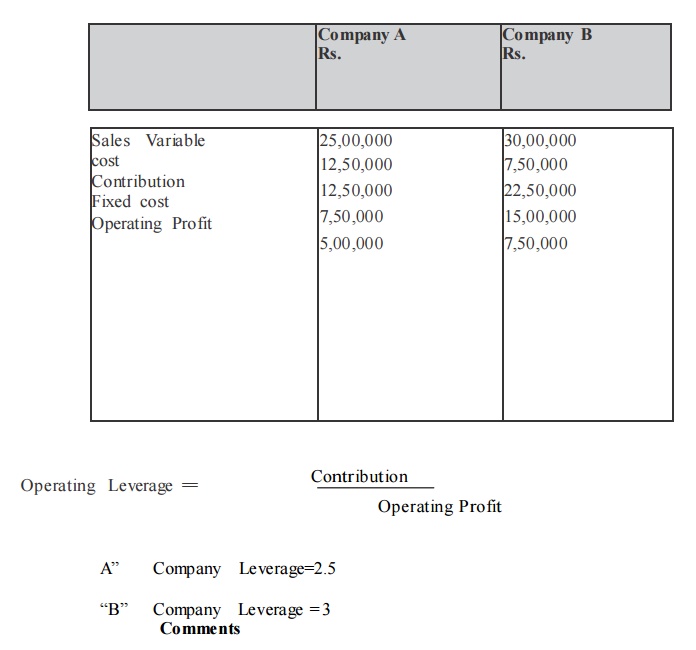
Operating leverage for B Company is Aigher than that of A Company; B Company has a higher degree of operating risk. The tendency of operating profit may vary portionately with sales, is higher for B Company as compared to A Company.

Illustration

The following information is available for two companies.

ILLUSTRATION

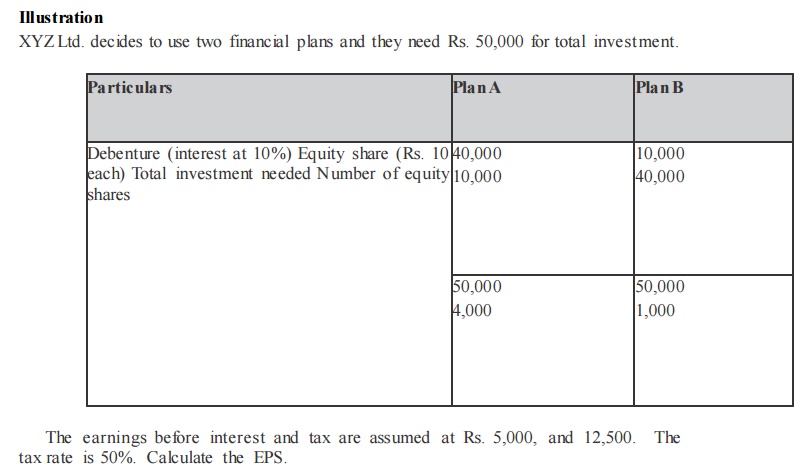
Calculate operating leverage and financial leverage under situatio ns A, B and C and financial plans 1, 2 and 3 respectively from the following infor mation relating to the operating and financial leverage which give the highest value and the least value.

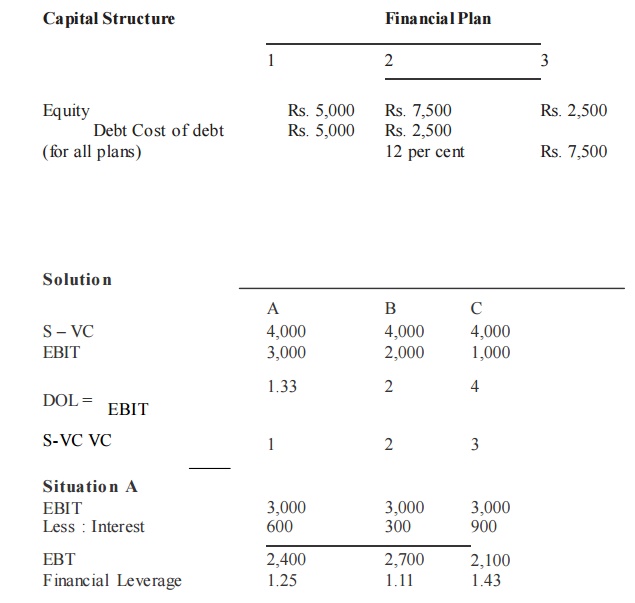
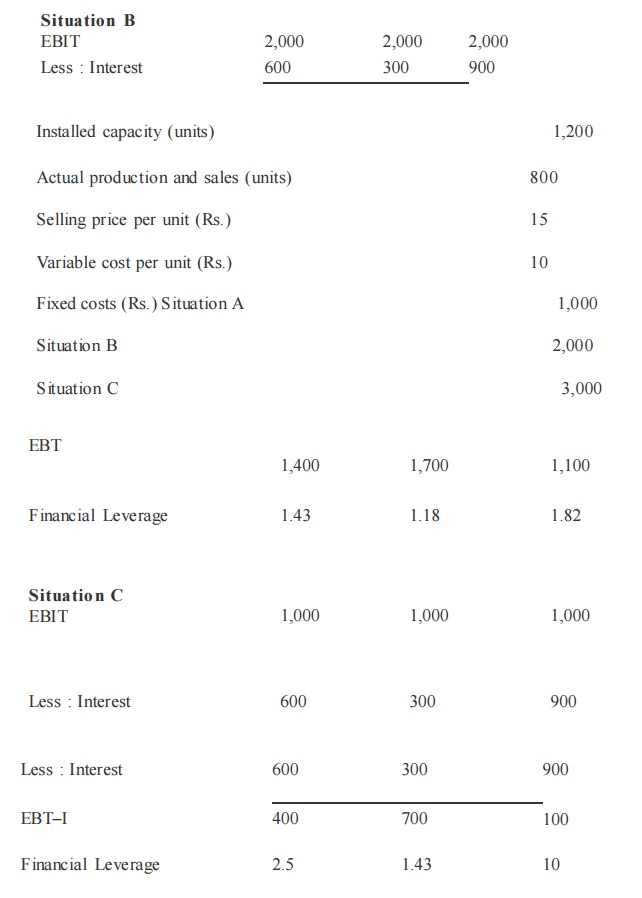
FINANCIAL STRUCTURE 

Example  

From  the  following  information,  calculate the  capitalization,  capital  structure  and financial structures.  

Balance Sheet  

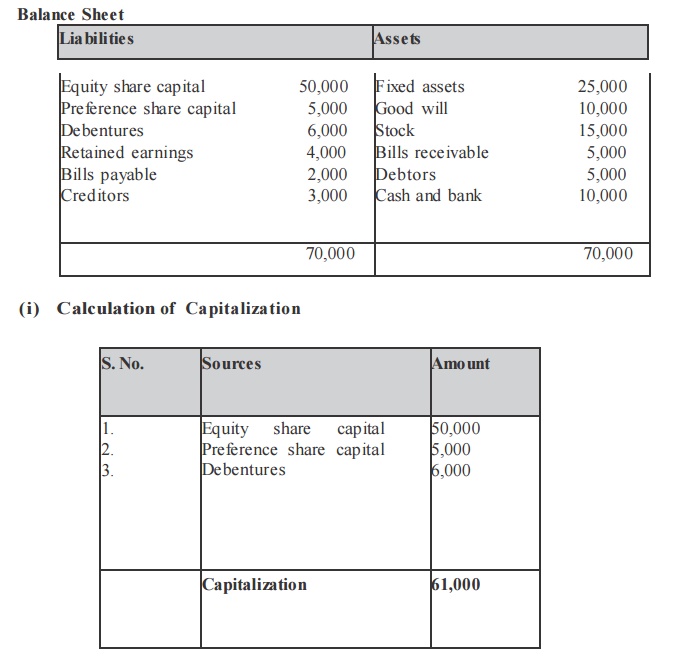
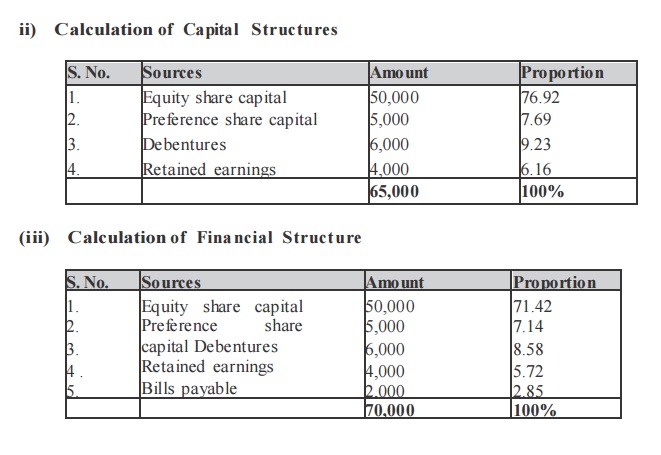
illustration 

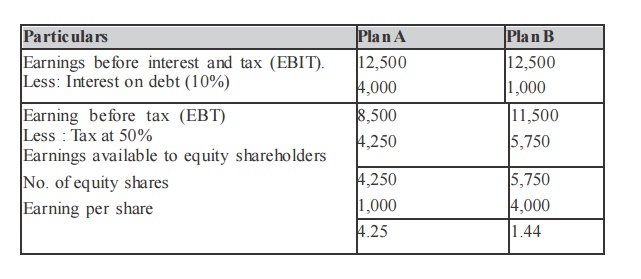
ABC Ltd., needs Rs. 30,00,000 for the installation of a new factory. The new factory expects to yield annual earnings before interest and tax (EBIT) of Rs.5,00,000. In choosing a financial plan, ABC Ltd., has an objective of maximizing earnings per share (EPS). The company proposes to issuing ordinary shares and raising debit of Rs. 3,00,000 and Rs. 10,00,000 of Rs. 15,00,000. The current market price per share is Rs. 250 and is expected to drop to Rs. 200 if the funds are borrowed in excess of Rs. 12,00,000.

Funds can be raised at the following rates. 

–up to Rs. 3,00,000 at 8%

–over Rs. 3,00,000 to Rs. 15,000,00 at 10%

–over Rs. 15,00,000 at 15%

Assuming a tax rate of 50% advise the company.

Solutio n

Earnings Before Interest and Tax (BIT) less Interest Earnings Before Tax less: Tax@50%.

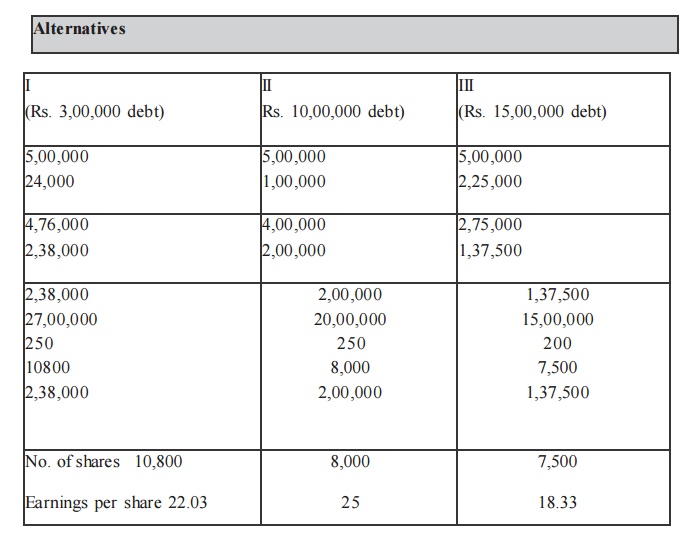
The secure alter native which gives the highest earnings per share is the best. Therefore the company is advised to revise Rs. 10,00,000 through debt a mo unt Rs. 20,00,000 through ordinary shares.

illustration

Compute     the     market        value of the firm,  value  of shares  and  the         average  cost of capital from the    following  information.                               

Net operating income                                 Rs.    1,00,000               

Total investment                               Rs.    5,00,000               

Equity  capitalization     Rate:                                        

(a)   If the firm  uses no debt  10%                                 

(b)     If the firm uses     Rs. 25,000 debentures 11% (c)         

If the firm uses     Rs. 4,00,000  debentures 13%          

Assume       that Rs. 5,00,000 debentures can  be raised  at 6%  rate         of interest whereas

Rs. 4,00,000 debentures can be raised at 7% rate of interest.

 Solutio n                                                             

Computation of market value of firm value of shares  and the average cost of capital.

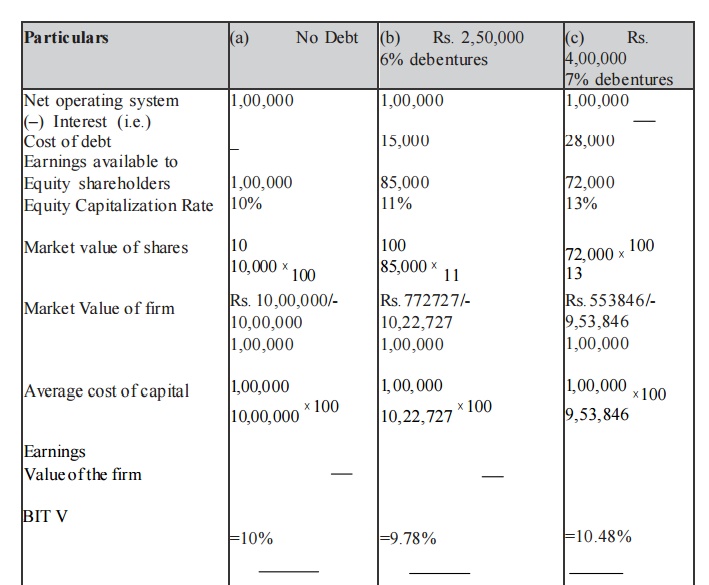
Comments

From the above data,  if debt of Rs. 2,50,000 is used, the value of the firm increases  and the overall cost of capital decreases. But, if more  debt is used  to  finance in place of equity  i.e., Rs. 4,00,000  debentures, the  value  of the firm  decreases  and the  overall  cost of capital increases.                  

illustration                   

(a) A Company expects a net income of Rs. 1,00,000. It has Rs. 2,50,000, 8% debentures. 

The equality capitalization rate  of the  company is  10%. Calculate  the value  of the  firm and  overall  capitalization rate  according  to the net income approach (ignoring income tax).                   

(b)   If the debenture debts  are  increased to  Rs. 4,00,000. What  shall be the value  of the  firm and  the overall  capitalizatio n rate?         

Solution                   

(a) Capitalization of the value of the  fir m         Rs.                     

Net  income            1,00,000     

Less: Interest on 8% Debentures of Rs. 2,50,000 20,000     

Earnings available  to equality shareholders   80,000    

Equity capitalizatio n rate             10%    

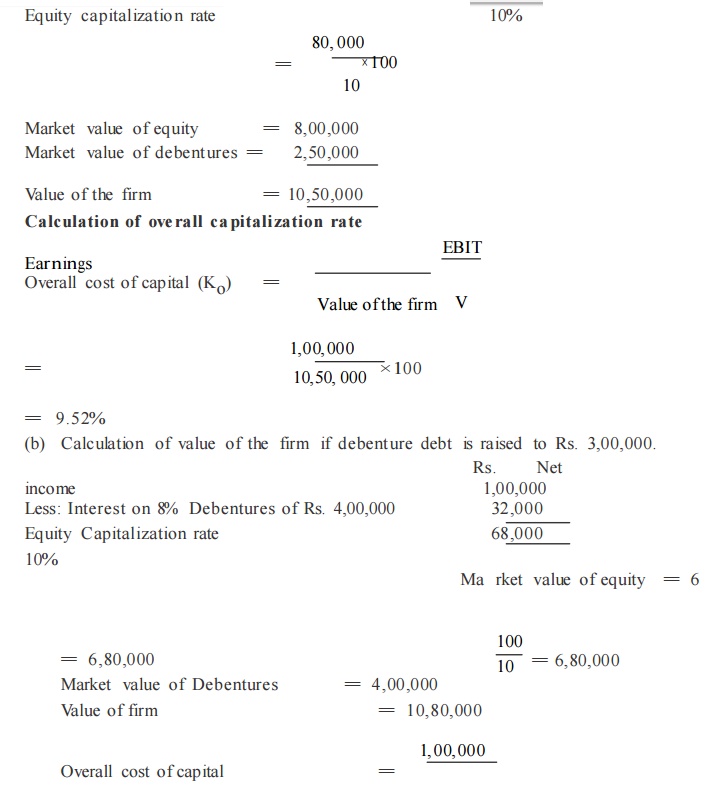
XYZ expects a net operating income of Rs. 2,00,000. It has 8,00,000, 6% debentures. The overall capitalization rate is 10%. Calculate the value of the firm and the equity capitalization rate (Cost of Equity) according to the net operating income approach. 

 If the debentures debt is increased to Rs. 10,00,000. What will be the effect on volume of the firm and the equity capitalization rate? 

Solutio n

Net operating income = Rs. 2,00,000 

Overall cost of capital = 10% 

Market value of the firm (V)

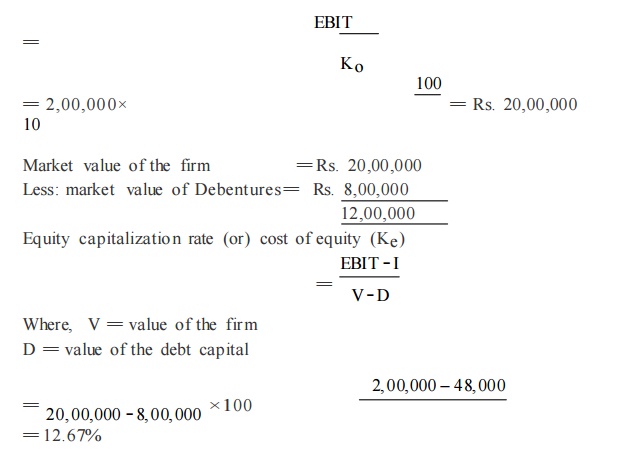
If the debentures debt is increased to Rs. 10,00,000, the value of the firm shall rema in changed to Rs. 20,00,000. The equity capitalization rate will increase as follows:

illustration

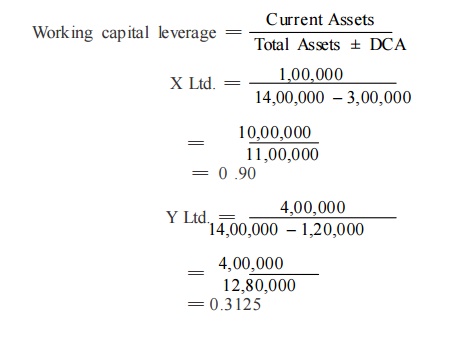
Financing Decision and working capital management

-         1Capitalization rate is 10%, Earning per share Rs. 50. Assume rate of return on investment 12%, 8%,

-         10 % show the effect of dividend policy on market price per share applying watter‘s formula when

-         dividend payout ratio is

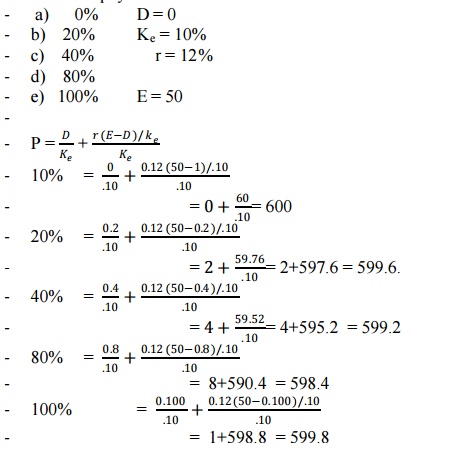
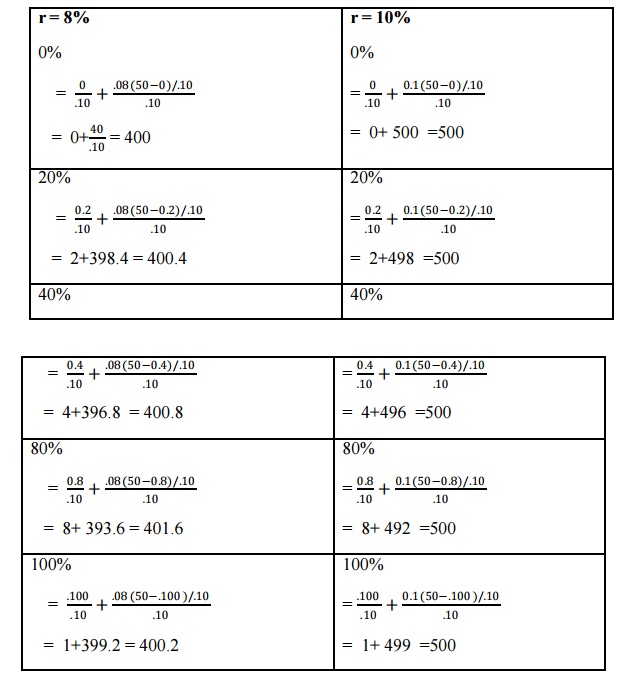
2. The following information in available irrespective of r, k, e of ABC Ltd. Rate of reture R = 15%, r = 12% r = 10% cost of capital = 12%, Earning per share 10 determine the value of its shares using Gordons model assuming the following

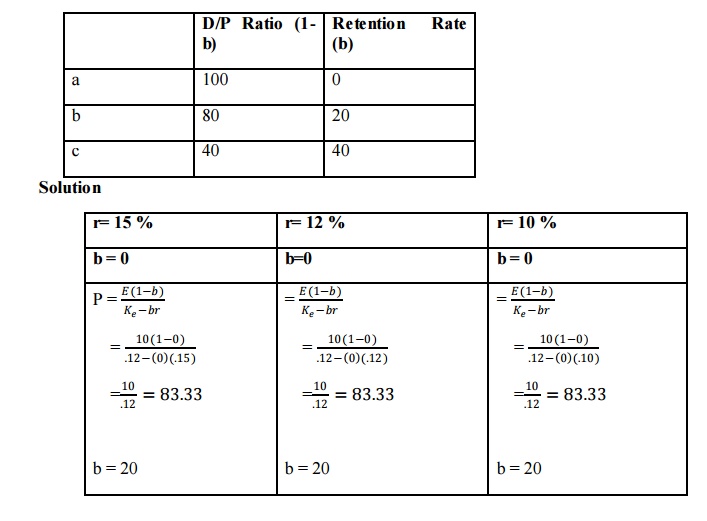
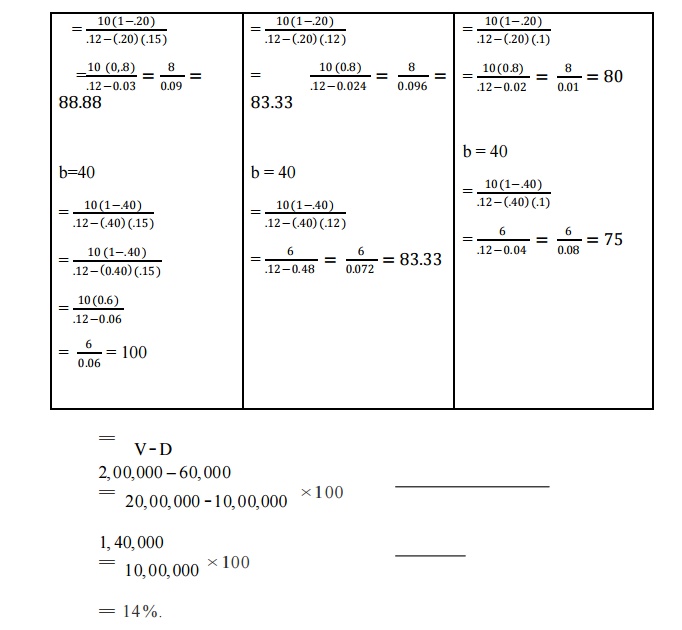
= 9.26 % 

Thus, it is evident that with the increase in debt financing, the value of the firm has increased and the overall cost of capital has increased.

Problem

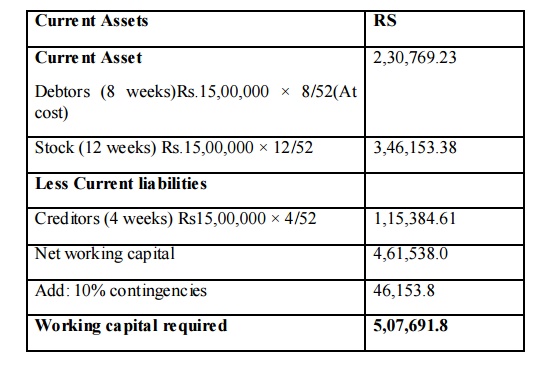
1. Prepare an estimate of working capital requirement from the following data of the XYZ Ltd.

a) Projected annual sales volume 2,00,000 units

b) Selling price Rs.10 per unit

c) % of net profit on sales 25% 

d) Average credit period allowed to customers 8 weeks

e) Average credit period allowed by suppliers 4 weeks

f) Average holding period of the inventories 12 weeks

g) Allow 10% for contingencies 

Solution 

Statement of working capital requirements

Average Credit Purchase Per Day 

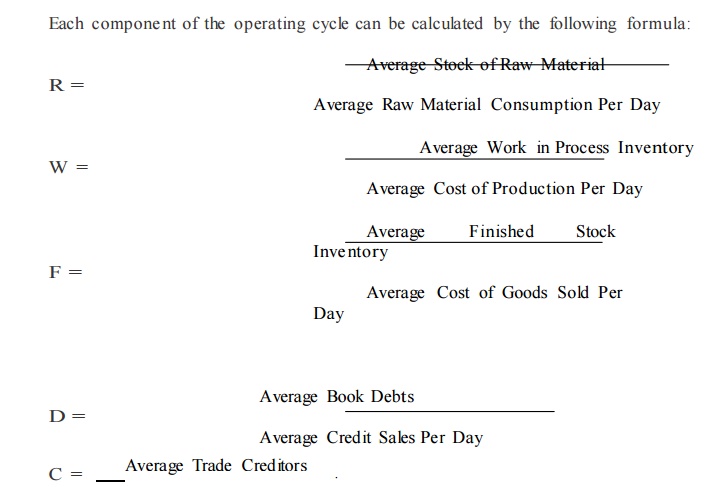
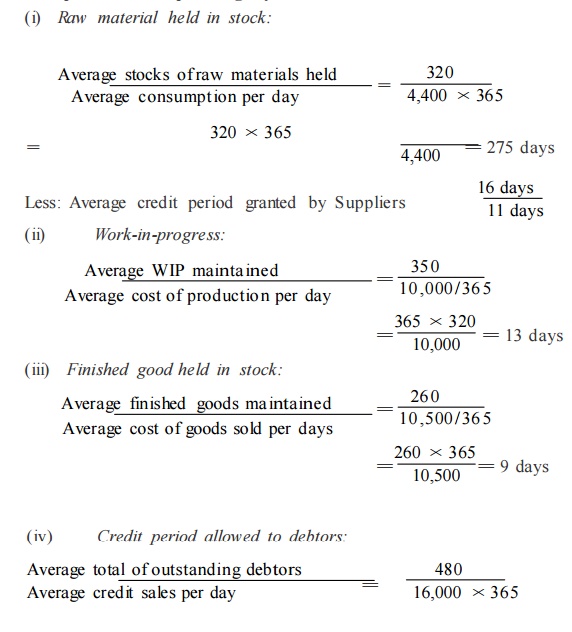
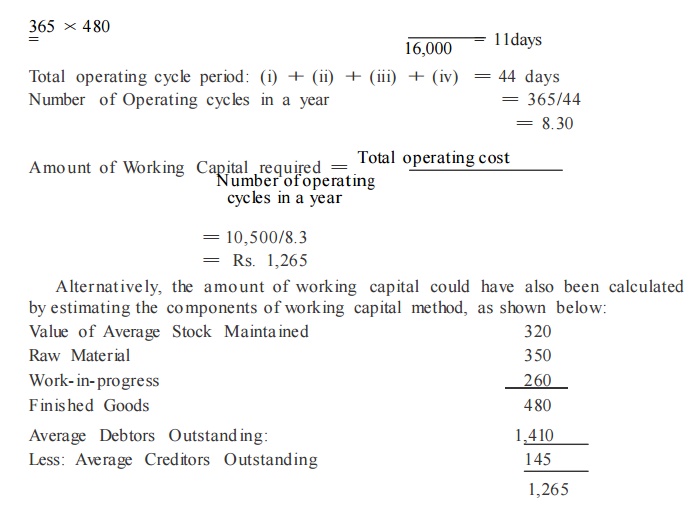
From the following information extracted from the books of a ma nufacturing company, compute the operating cycle in days and the a mount of working capital required:  

Period Covered 365 days

Average period of credit allowed by suppliers 16 days

Average Total of Debtors Outstand ing 480 00 

Raw Material Consumption 4,400 00

Total Production Cost 10,000 00

Total Cost of Sales 10,500 00

Sales for the year 16,000 00

Value of Average Stock maintained : 

Raw Material 320 00 

Work- in- progress 350 00 

Finished Goods 260 00

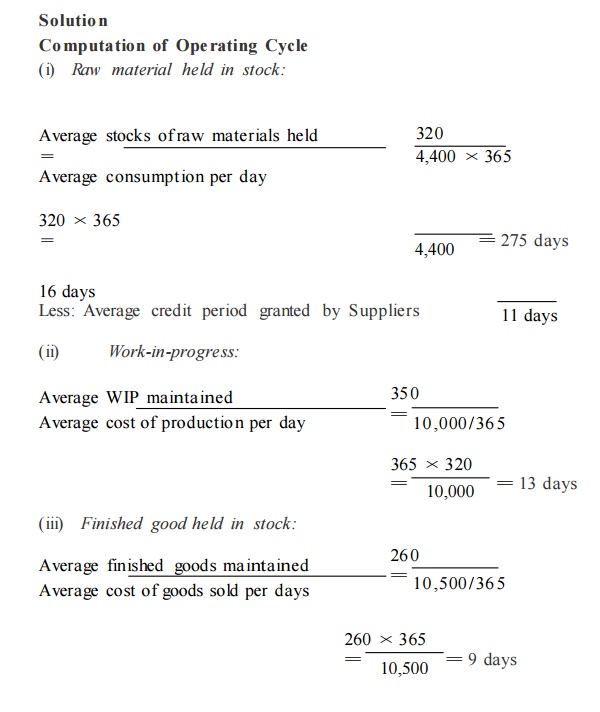
illustration

From the following information extracted from the books of a manufacturing company, compute the operating cycle in days and the a mount of working capital required:

Period Covered 365 days

Average period of credit allowed by suppliers 16 days

Average Total of Debtors Outstanding 480 00 

Raw Material Consumption 4,400 00

Total Production Cost 10,000 00

Total Cost of Sales 10,500 00

Sales for the year 16,000 00

Value of Average Stock maintained : 

Raw Material 320 00 

Work- in- progress 350 00 

Finis hed Goods 260 00 

Solution 

Computation of Ope rating Cycle 

(i) Raw material held in stock:

Exercise 1 

(a) Find out the economic order quantity and the number of orders per year from the following information: 

Annual consumption: 36,000 units

Purchase price per units: Rs. 54

Ordering            cost  per        order:          Rs.  150

Inventory  carrying                 cost  is  20%  of the        average       inventor y.

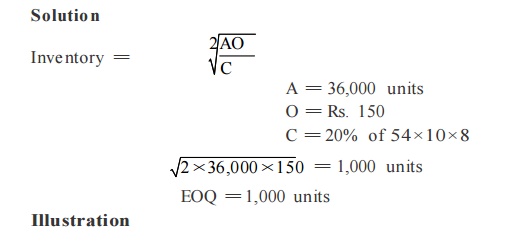
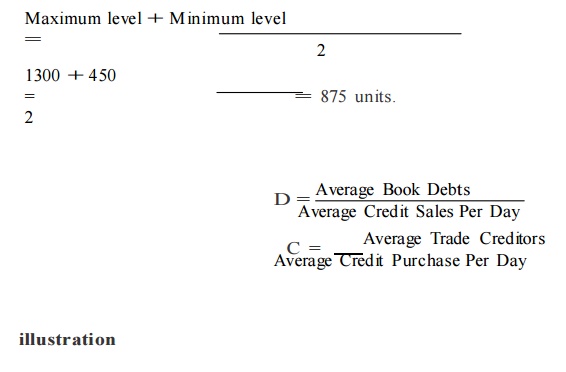
From  the              following                   information calculate,               (1) Re- order               level (2)   Maximum                 level

(3) Minimum level (4) Average level Normal usage: 100 units per week Maximum usage: 150 units per week Minimum usage: 50 units per week Re-order quantity (EOQ) 500: units Log in time: 5 to 7 weeks

Solutio n

(1)  Re-order  Level

=      Maximum  consumption  × Maximum  Re-order  period

=     150×7=1050  units

(2)  Maximum         Level

= Re-order le ve l+ Re- order qua ntity

–( Minimum consumption× Minimum delivery period) = 1050 + 500 – (50 × 5) = 1300 units

(3)  Minimum Level

= Re-order level – (Nor mal co nsumption×Normal delivery period) = 1050 – (100 × 6 ) = 450 units

Illustration

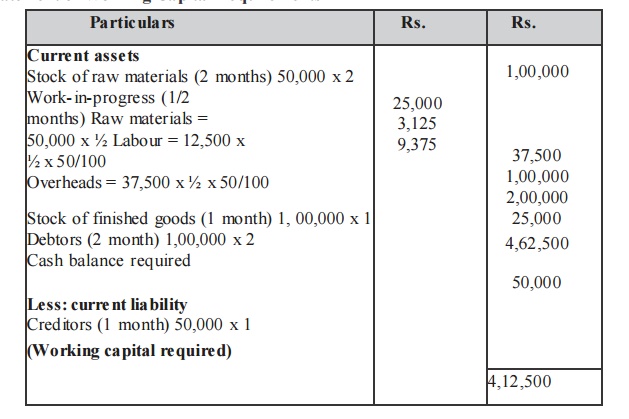
The board of directors of Aravind mills limited request you to prepare a statement showing the working capital requirements for a level of activity of 30,000 units of output for the year. The cost structure for the company‘s product for the above mentioned activity level is given below.

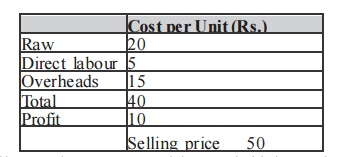
(a)Past experience indicates that raw materials are held in stock, on an average for 2 months.

(b) Work in progress (100% complete in regard to materials and 50% for labour and overheads) will be half a month‘s production.

(c) Finished goods are in stock on an average for 1 month. 

(d) Credit allowed to suppliers: 1 month.

(e) Credit allowed to debtors: 2 months.

(f)   A minimum cash  balance of Rs 25,000  is expected  to  be maintained. Prepare a state me nt of working capital requirements. 

Solution       

Output per  annum  = 30,000  units     

Output per  annum  = 12%  of 30,000  =2,500 units 

Raw materials  p. m. Rs. 20×2500 = 50,000   

Labour  p. m. Rs. 5×2,500  =  12,500   

Overheads p. m. Rs. 15×2,500 = 37,500   

 Total:   1,00,000      

Statement of Working Capital Require ments

illustration

Prepare an estimate  of working  capital requirement from the         following  information of a trading concern.

Projected  annual sales            10,000        units

Selling price                                                Rs. 10 per unit

Percentage of net profit on sales                                                                 20% Average

credit  period  allowed  to  customers                                                                  8 Weeks

Average  credit  period  allowed  by suppliers                        4 Weeks

Average  stock  holding  in terms  of sales require me nts12 Weeks

Allow 10% for contingencies

Solution

Statement of Working Capital Requirements

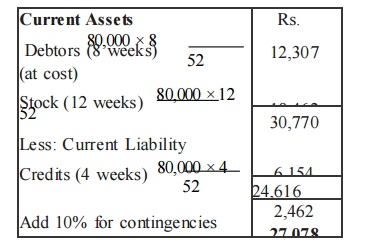
Working  Notes                                 

Sales = 10000×10 = Rs. 1,00,000                                                     

Profit  20% of Rs. 1,00,000  = Rs. 20,000           

Cost of Sales=Rs.1,00,000 – 20,000          = Rs. 80,000

As it is a trading  concern, cost of sales is assumed       to be the      purchases.

Illustration

Prepare       an estimate  of working  capital require me nt from the following informations of a trading        concern.

Projected  annual sales                                                                               Rs.  6,50,000

Percentage of net profit on sales                                                                                    25% Average

credit  period  allowed  to debtors                                                                                 10 Weeks

Average  credit  period  allowed  by creditors                                            4 Weeks

Average  stock  holding  in terms  of sales requirements                  8 Weeks

Allow 20% for contingencies

Working    Notes                    

Sales = Rs. 6,50,000                         

Profit 25 /125 of Rs. 6,50,000 = Rs. 1,30,000               

Cost of Sales= Rs. 6,50,000 –1,30,000=Rs.        5,20,000

As it is a trading   concern, cost of sales is assumed  to be the purchases.

Illustration

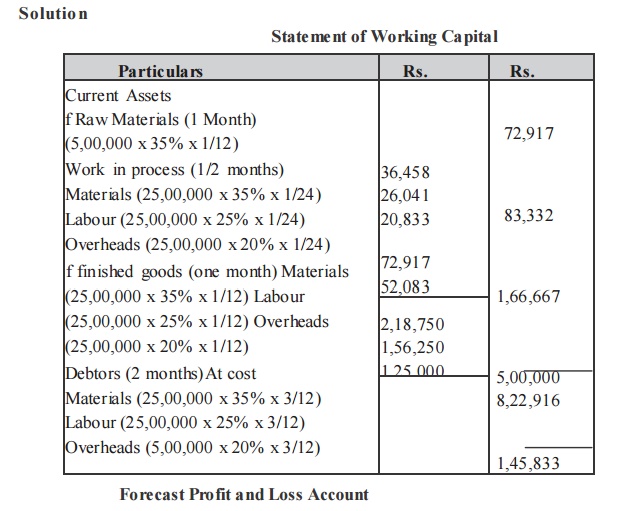
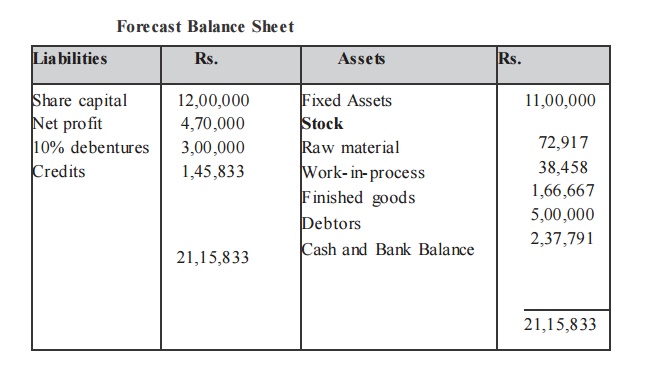
A Performa cost sheet of a company  provides the  following  particulars :

Elements of  cost

Material                                                                35%                                         

Direct  Labours                                                    25%                                         

Overheads                                                            20%                                         

Further particulars available  are:                                                                        

(i)      It  is proposed to  maintain a level of activity of 2,50,000      units.

(ii)     Selling         price is Rs.  10/-  per  unit                                                                

(iii)    Raw  materials are  to  remain in stores  for an average  period  of one    mo nth.

(iv)    Finished  foods     are  required  to be in stock  for an average         period  of one mo nth. 

( v)    Credit         allowed  to  debtors       is 3  months.        

(vi)              Credit         allowed       by suppliers is 2  months.

 You are      required      to prepare a statement of working    capital         requirements, a forecost profit  and        loss account and balance  sheet of the company  assuring that

Share Capital                                              Rs.    12,00,000                                

10%            Debentures                                        Rs.    3,00,000                                  

Fixed Assets                                               Rs.    11,00,000