Exercise 1.1: Cartesian Product

Exercise 1.1

1. Find A ×B , A ×A and B ×A

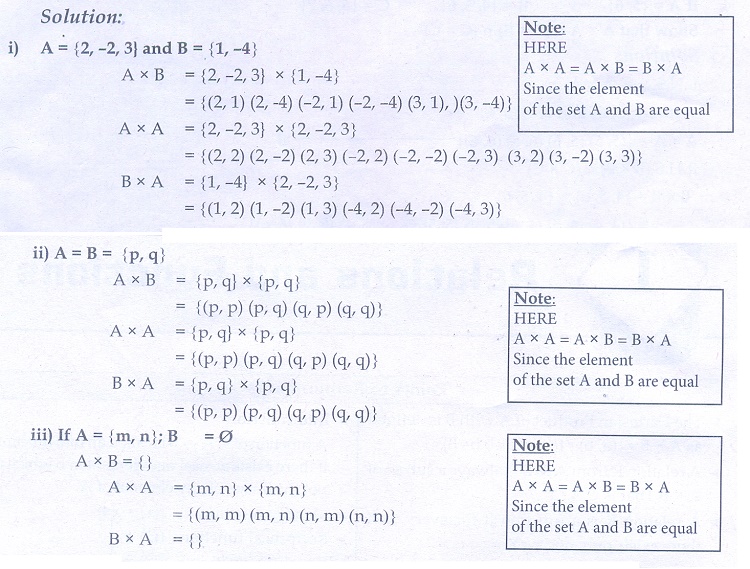
(i) A= {2, −2, 3} and B = {1, −4} (ii) A = B ={p , q} (iii) A ={m,n} ; B =ɸ

2. Let A = {1,2,3} and B = {x | x is a prime number less than 10}. Find A ×B and B ×A.

3. If B ×A = {(− 2, 3),(−2, 4),(0, 3),(0, 4),(3, 3),(3, 4)} find A and B.

4. If A = {5, 6} , B = {4, 5, 6} , C = {5, 6, 7} , Show that A ×A = (B ×B ) ∩(C ×C) .

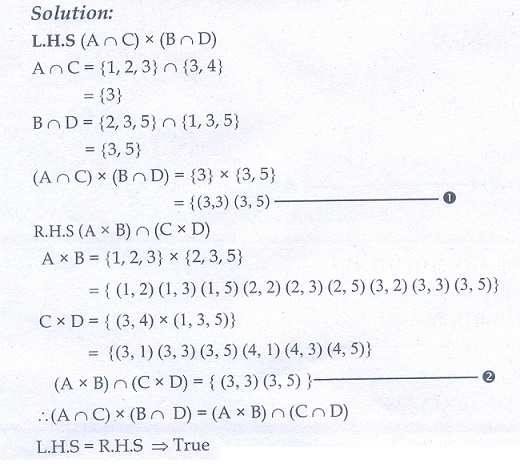
5. Given A={1,2,3}, B = {2,3,5}, C = {3,4} and D = {1,3,5}, check if

(A ∩ C)×(B ∩ D) = (A ×B ) ∩(C ×D) is true?

6. Let A = {x ∈ W | x < 2} , B = {x ∈ N | 1 < x ≤ 4} and C = {3, 5} . Verify that

(i) A × ( B  ∪  C)  = (A × B) ∪ (A × C )

(ii) A × (B ∩ C) = (A × B) ∩ (A × C)

(iii) (A ∪ B)×C = (A×C ) ∪ (B ×C)

7. Let A = The set of all natural numbers less than 8, B = The set of all prime numbers less than 8, C = The set of even prime number. Verify that

(i) (A ∩ B)×C = (A×C ) ∩ (B ×C)

(ii) A × (B −C ) = (A×B ) − (A×C)

Answers:

1.(i)

A × B = {(2, 1),(2, − 4),(−2, 1),(− 2, −4),(3, 1),(3, −4)}

A × A = {(2, 2),(2, − 2),(2, 3),(−2, 2),(− 2, −2),(− 2, 3),(3, 2),(3, −2),(3, 3)}

B × A = {(1, 2),(1, − 2),(1, 3),(−4, 2),(− 4,−2),(−4, 3)}

(ii) A × B = {( p, p)(p, q)(q , p)(q , q)}; A × A = {( p, p),( p, q),(q , p),(q , q)} ;

B × A = {( p, p),( p, q),(q , p),(q , q)}

(iii) A × B = { }; A × A = {(m, m ),(m, n),(n, m ),(n, n)}; B × A = { }

2. A × B = {(1, 2),(1, 3),(1, 5),(1, 7),(2, 2),(2, 3),(2, 5),(2, 7),(3, 2),(3, 3),(3, 5),(3, 7)}

B × A = {(2, 1),(2, 2),(2, 3),(3, 1),(3, 2),(3, 3),(5, 1),(5, 2),(5, 3),(7, 1),(7, 2),(7, 3)}

3. A = {3, 4} B = {−2, 0, 3}

5. true