Core type transformer - Design of Transformers

Core type transformer

Note:

1. D-distance between the two core or leg central lines

2. Width Ww is measured from one edge of the leg to the other of the adjacent leg in case of square  or rectangular core with square or rectangular coil and between the two circumscribing circles of adjacent  legs in case of stepped legs.

3. Depth or width of the core type transformer = b in case of rectangular core

 = a in case of square or stepped core

Overall length = (Ww + 2a) or (D+a) in case of single phase core type transformer with square or   rectangular core

 = (Ww+d+a) or (D+a) in case of single phase core type transformer with a   Stepped leg

 = (2Ww + 2d + a) or (2D+a) in case of three phase core type transformer with   a stepped leg. No square or rectangular leg is used for high capacity three   phase transformers.

 Overall height = (Hw + 2Hy) for all core type transformers.

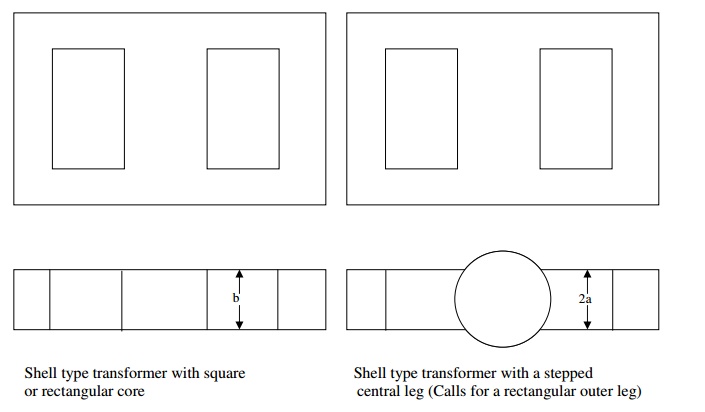
 Depth or width of the Shell type transformer = b in case of rectangular central leg

 = 2a in case of stepped central leg

 Overall length = (2Ww + 4a) in case of a shell type transformer with rectangular or square central  leg

  = (d + 2Ww + 2a) in case of shell type transformer with central stepped leg

 Overall height = (Hw + 2Hy) in case of a single phase shell type transformer

 = 3(Hw+2Hy) in case of a three phase shell type transformer

Winding details:

 Since the applied voltage V1 is approximately equal to the voltage induced E1 = 4.44 φm f T1 = Et T1

 Number of primary turns (or turns / phase) T1 = V1 / Et in case of single phase transformers

 = V1ph / Et in case of 3 phase transformers

Number of secondary turns (or turns / phase) T2 = V2/ Et in case of single phase transformers

 = V2ph / Et in case of 3 phase transformers

Primary current (or current/phase) I1 = kVA x 103 / V1 in case of single phase transformers

 = kVA x 103 / 3V1ph in case of 3 phase transformers

Cross-sectional area of primary winding conductor a1 = I1/ δ mm2

Secondary current (or current / phase) I2 = kVA x 103 / V2 in case of single phase transformers

 = kVA x 103 / 3V2ph in case of 3 phase transformers

Cross-sectional area of secondary winding conductor a2 = I2/ δ mm2

 Knowing the number of turns and cross-sectional area of the primary and secondary winding  conductors, number of turns/layer in a window height of Hw and number of layers in a window width of  Ww can be found out.