Chapter: 11th Mathematics : UNIT 11 : Integral Calculus
BernoulliŌĆÖs formula for Integration by Parts
BernoulliŌĆÖs formula for Integration by Parts
If u and v are functions of x, then the BernoulliŌĆÖs rule is
Ōł½udv = uv ŌłÆ u ŌĆ▓v1 + u ŌĆ▓ŌĆ▓v2 - ......
where u ŌĆ▓, u ŌĆ▓ŌĆ▓, uŌĆ▓ŌĆ▓ŌĆ▓,... are successive derivatives of u
and v, v1 , v2 , v3 , are successive integrals of dv
BernoulliŌĆÖs formula is advantageously applied when u = xn ( n is a positive integer)
For the following problems we have to apply the integration by parts two or more times to find the solution. In this case BernoulliŌĆÖs formula helps to find the solution easily.
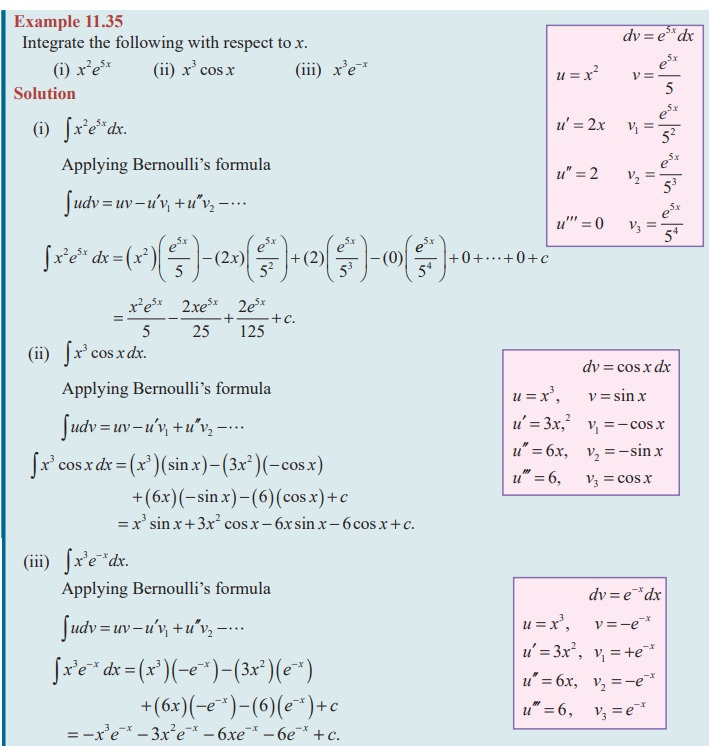
Example 11.35
Integrate the following with respect to x.
(i) x2 e5x (ii) x3 cos x (iii) x3eŌłÆ x
EXERCISE 11.7
Integrate the following with respect to x:
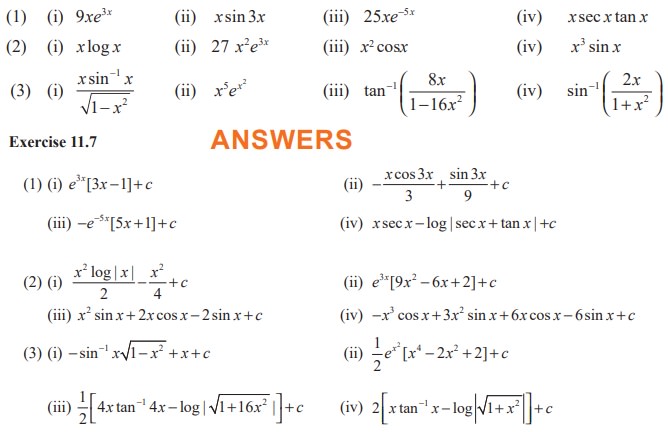
Related Topics