Wave Theory and Parrtical Theory

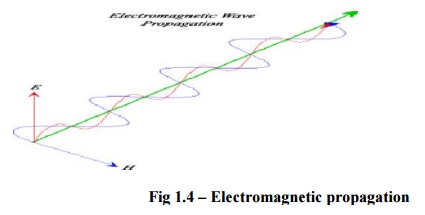
WAVE THEORY AND PARRTICAL THEORY

Light can exhibit both a wave theory, and a particle theory at the same time. Much of the time, light behaves like a wave. Light waves are also called electromagnetic waves because they are made up of both electric (E) and magnetic (H) fields. Electromagnetic fields oscillate perpendicular to the direction of wave travel, and perpendicular to each other. Light waves are known as transverse waves as they oscillate in the direction traverse to the direction of wave travel.

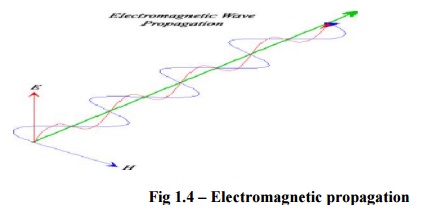
Fig 1.4 - Electromagnetic propagation

Waves have two important characteristics - wavelength and frequency.

The sine wave is the fundamental waveform in nature. When dealing with light waves, we refer to the sine wave. The period (T) of the waveform is one full 0 to 360 degree sweep. The relationship of frequency and the period is given by the equation:

f  = 1 / T

T  = 1 / f

The waveforms are always in the time domain and go on for infinity.

The speed of a wave can be found by multiplying the two units together. The wave's speed is measured in units of length (distance) per second:

Wavelength x Frequency = Speed

As proposed by Einstein, light is composed of photons, a very small packets of energy. The reason that photons are able to travel at light speeds is due to the fact that they have no mass and therefore, Einstein's infamous equation - E=MC² cannot be used. Another formula devised by Planck, is used to describe the relation between photon energy and frequency  

Planck's Constant (h)  - 6.63x10^-34 Joule-Second.

E = hf(or)E = hc / /?

E is the photonic energy in Joules, h is Planks constant  and f is the frequency in Hz.

PARTICAL THEORY

The basic idea of qua ntum theory is that radiant energy is trans mitted inindivisible packets whose energy is given in integral parts, of size hv, where h is Planck's constant = 6.6252 x 10-34 J - s, and v is the frequency of the radiation. These ar e called quanta or photons.

The dilemma of the si multaneous wave and particle waves of elec tromagneticenergy may be conceptually resolved by considering that energy is not supplied continuously throughout a wave, but rather that it is carried by photons. The classical w ave theory does not give the intensity of energy at a point in space, but gives the probability of finding a photon at that point. Thus the classica l concept of a wave yields to the idea th at a wave simply describes the probability path for the motion of the individual photons.

The particular impor tance of the quantum approach for remote sensing is thatit provides the concept of discrete energy levels in materials. The values a nd arrangement of these levels are different for different materials. Information about a given material is thus available in electromagnetic radiation as a consequence of transitions bettween these energy levels. A transition to a highe r energy level is caused by the absorption of energy, or from a higher to a lower energy leve l is caused by the' emission of energy. The amounts of energy either absorbed or emitted c orrespond precisely to the energy difference between the two levels involved in the transitio n. Because the energy levels are different for each material, the amount of energy a particular substance can absorb or emit is different for that material from any other materials. Conseque ntly, the position and intensities of the band s in the spectrum of a given material are characteriistic to that material.