Summary of Operations Research

Summary

Linear programming problem(LPP) is a mathematical modeling technique which is used to allocate limited available resources in order to optimize (maximize or minimize) the objective function.

Short form of LPP

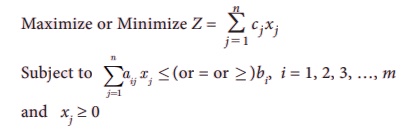
Objective function: A function Z = c₁x ₁ +c₂x₂ +…. +c_nx_n which is to be optimized (maximized or minimized) is called objective function.

Decision variable: The decision variables are the variables we seek to determine to optimize the objective function. x_j , j = 1,2,3,…,n , are the decision variables.

Solution: A set of values of decision variables x_j,j =1,2,3,…,n satisfying all the constraints of the problem is called a solution to that problem.

Feasible solution: A set of values of the decision variables that satisfies all the constraints of the problem and non-negativity restrictions is called a feasible solution of the problem.

Optimal solution: Any feasible solution which maximizes or minimizes the objective function is called an optimal solution.

Feasible region: The common region determined by all the constraints including non-negative constraints x_j≥0 of a linear programming problem is called the feasible region ( or solution region) for the problem.

Linear programming problems which involve only two variables can be solved by graphical method.

It should be noted that the optimal value of LPP occurs at the corner points of the feasible region

Network is a diagrammatic representation of various activities concerning a project arranged in a logical manner.

An activity is a task or item of work to be done, that consumes time, effort, money or other resources

The beginning and end points of an activity are called events (or nodes).

Critical path: The longest path connected by the activities in the network is called the critical path. A path along which it takes the longest duration.