Study of Relation Between Length of the Given Wire and Tension for a Constant Frequency Using Sonometer

STUDY OF RELATION BETWEEN LENGTH OF THE GIVEN WIRE AND TENSION FOR A CONSTANT FREQUENCY USING SONOMETER

AIM

To study the relationship between the length of a given wire and tension for constant frequency using a sonometer.

APPARATUS REQUIRED

Sonometer, tuning fork of known frequency, meter scale, rubber pad, paper rider, hanger with half – kilogram masses, wooden bridges.

FORMULA

 The frequency of the fundamental mode of vibration of a string is given by,

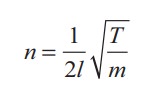
 If n is a constant, for a given wire (m is constant)

√T/l is constant.

 Where

 n → Frequency of the fundamental mode of vibration of a string (Hz)

 m → Mass per unit length of string (kg m^–1)

 T → Tension in the string (including the weight of the hanger) = Mg (N)

 l → Length of the string between the wedges ( metre )

 M → Mass suspended, including the mass of the hanger (kg)

DIAGRAM

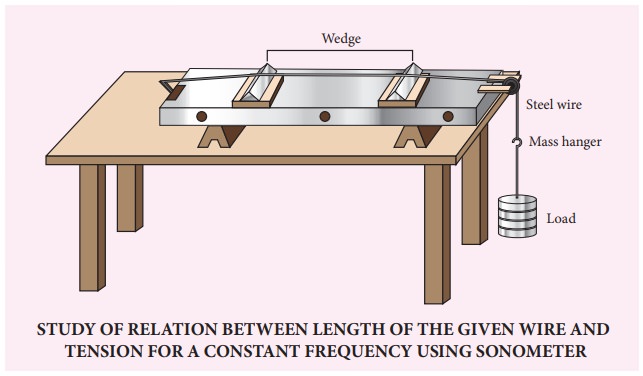
PROCEDURE

·         Set up the sonometer on the table and clean the groove on the pulley to ensure that it has minimum friction.

·         Set a tuning fork of known frequency into vibration by striking it against the rubber pad. Plug the sonometer wire and compare the sound due to the vibration of tuning fork and the plugged wire.

·         Adjust the vibrating length of the wire by the adjusting the bridge B till the two sounds ap-pear alike.

·         Place a mass of 1 kg for initial reading in the load hanger.

·         For final adjustment place a small paper rider R in the middle of the wire AB.

·         Now, strike the tuning fork and place its shank stem on the bridge A and then slowly adjust the position of the bridge B till the paper rider is agitated violently (might eventually falls) indicating resonance.

·         Measure the length of the wire between wedges at A and B which is the fundamental mode corresponding to the frequency of the tuning fork.

·         Increase the load on the hanger in steps of 0.5 kg and each time find the resonating length as done before with the same tuning fork.

·         Record the observations in the tabular column.

MODEL GRAPH

OBSERVATIONS

Frequency of the tuning fork = ________ Hz

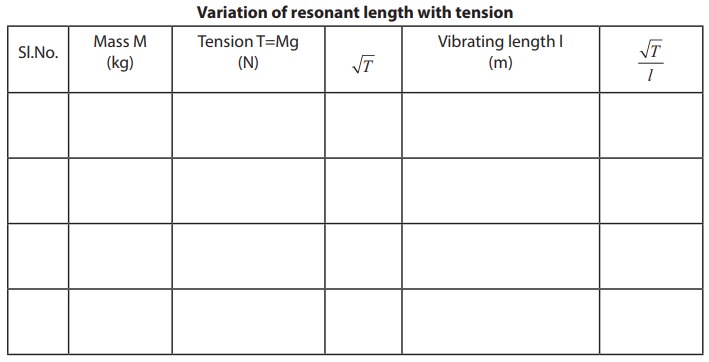
CALCULATION

Calculate the value √T/l for the tension applied in each case.

RESULT

·           The resonating length varies as square root of tension for a given frequency of vibration of a stretched string.

·           √T/l is found to be a constant.