Solved Problems: Thermodynamics Second Law

1. Two kg of air at 500kPa, 80°C expands adiabatically in a closed system until its volume is doubled and its temperature becomes equal to that of the surroundings which is at 100kPa and 5°C.

For this process, determine

1) the maximum work

2) the change in a availability and

3) the irreversibility.

Take, C_v = 0.718 KJ/kg K, R = 0.287 KJ/kg K.

2. A reversible heat engine receives 3000 KJ of heat from a constant temperature source at 650 K . If the surroundings is at 295 K,

determine

i) the availability of heat energy

ii)  Unavailable heat.

A = Q₁ –T₀ (ΔS)

= 3000 –295 (3.17)

= 2064.85 KJ.

Unavailable heat (U.A) = T₀ (ΔS)

= 295 (3.17)

= 935.15 KJ.

Result:

1) The availability of heat energy (A) = 2064.85 KJ.

2) Unavailable heat (U.A) = 935.15 KJ.

3. Air in a closed vessel of fixed volume 0.15 m³ exerts pressure of 12 bar at 250 °C. If the vessel is cooled so that the pressure falls to 3.5 bar , determine the final pressure, heat transfer and change of entropy.

Given Data:

V₁ = 0.15 m³

p₁ = 12 bar = 1200 KN/m² p₂ = 3.5 bar = 350 KN/m²

T₁ = 250°C = 273+250 = 523 K

To find:

1)    The final pressure,

2)    Heat transfer

3)    Change of entropy.

Solution:

Result:

1)    The final pressure, T₂ = 152.54 K.

2)    Heat transfer, Q = - 446.78 KJ.

3)  Change   of   entropy,-1.06KJ/K.   ΔS   =

4. A domestic food freezer maintains a temperature of - 15°C. The ambient air is at 30°C. If the heat leaks into the freezer at a continuous rate of 1.75 KJ/s, what is the least power necessary to pump the heat out continuously?

Given Data:

T_L = - 15°C = 273 –15 = 258 K

T_H = 30°C  = 273 + 30 = 303 K

Q_S = 1.75 KW

To find:

Least power, (W)

Solution:

Result:

Least power necessary to pump heat, W = 0.305 KW.

5. A refrigerator working on reversed Carnot cycle requires 0.5 KW per KW of cooling to maintain a temperature of -15°C. Determine the following:

a) COP of the refrigerator

b) Temperature at which heat is rejected and

Amount of heat rejected to the surroundings per KW of cooling.

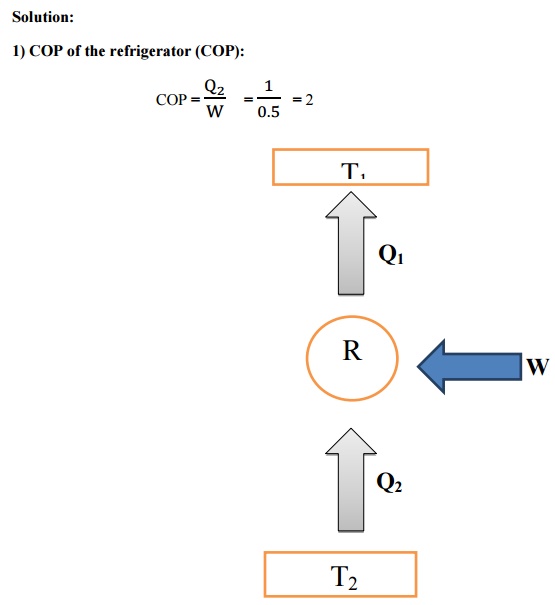
Given Data:

W = 0.5 KW

Q₂ = 1 KW

T₂ = -15°C = 273 –15 = 258 K.

To find:

1) COP of the refrigerator (COP)

2) Temperature at which heat is rejected (T₁)

3) Amount of heat rejected to the surroundings per KW of cooling (Q₁)

Solution:

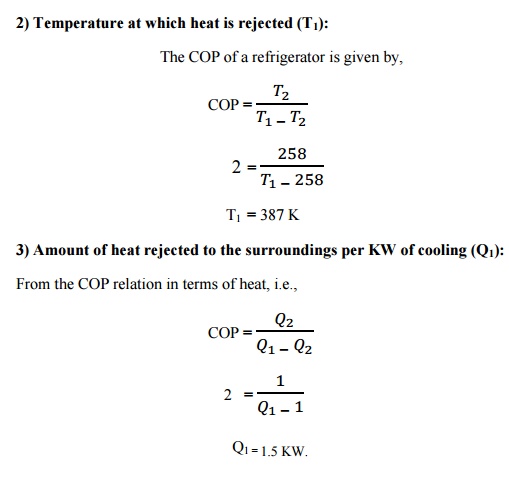
Result:

1) COP of the refrigerator (COP) = 2

2) Temperature at which heat is rejected (T₁) = 387 K

Amount of heat rejected to the surroundings per KW of cooling (Q₁) = 1.5 KW.