Chapter: Mechanical : Strength of Materials : Deflection of Beams

Solved Problems: Deflection of Beams

Mechanical - Strength of Materials - Deflection of Beams

Problem –1:

Determine the deflection of a given beam at the point loads. Take I = 64x10^-4 mm⁴ & its Young’s modulusN/mm(E).

Problem –2:

A steel cantilever beam of 6m long carries 2 point loads 15KN at the free end and 25KN at the distance of 2.5m from the free end. To determine the slope at free end & also deflection at free end I = 1.3x10⁸mm⁴. E = 2x10⁵ N/mm²

Solution:

Given:

Length (l) = 6m

loads (w1) = 25KN

(w2) = 15KN

I = 1.3x108 mm⁴

= 1.3x10-4m⁴

E = 2x105 N/mm²

= 2x108 KN/m²

Bending moment calculation:

Bending moment at C = 0

Bending moment at B = -(15x2.5) = - 37.5 KNm

Bending moment at A = -(15x6) –(25x3.5) = -177.5KNm

To find Bending moment Area:

Area of section (1) = ½ (bh)

a1 = ½ (2.5x37.5) = 46.875 m²

Area of section (2) = lb

a2 = 3.5x37.5 = 131.25m²

Area of section (3) = ½(bh)

a3 = ½(3.5x140) = 245m²

Total bending moment area

A = a1 + a2 + a3

= 46.8 + 131.25 + 245 = 423.125m²

To find the slope at free end:

According to moment area

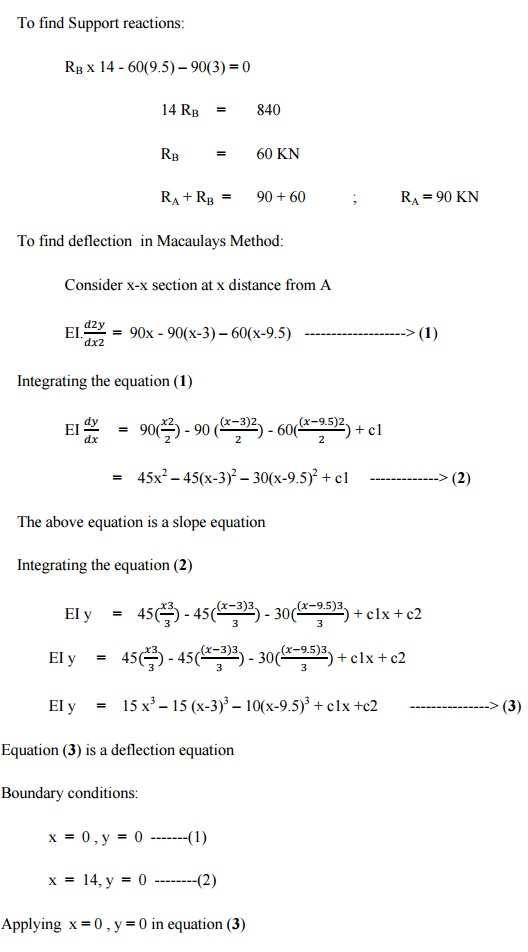
Problem –3:

Determine the deflection under point load.

E = 2x10⁵ KN/m²

I = 1x10^-4 m⁴ . Using moment area method.

Solution:

To find support reactions:

Taking moment about A,

R_B x 4 –(10x 3) –10 = 0

4R_B = 40

R_B = 4

R_A + R_B = 20

R_A = 10

R_A = 10KN

R_B = 10KN

Bending moment calculation:

Bending moment at B = 0

Bending moment at D = (10x1) = 10KNm

Bending moment at C = (10x3) - (10x2) = 10KNm

Bending moment at A = (10x4) - (10x3) –(10x1) = 0

To find area of bending moment:

A₁ = ½(bh)

= ½(1)x10 = 5m²

A₂ = 1/2(bh) = 5m²

A₃ = lxb = 10x2 = 20m²

To find slope at point load:

Study Material, Lecturing Notes, Assignment, Reference, Wiki description explanation, brief detail

Mechanical : Strength of Materials : Deflection of Beams : Solved Problems: Deflection of Beams |