Deflection of Beams

DEFLECTION OF BEAMS

Elastic curve of neutral axis

Assuming that the I-beam is symmetric, the neutral axis will be situated at the midsection of the beam. The neutral axis is defined as the point in a beam where there is neither tension nor compression forces. So if the beam is loaded uniformly from above, any point above the neutral axis will be in compression, whereas any point below it will be in tension

.

However, if the beam is NOT symmetric, then you will have to use the following methodology to calculate the position of the neutral axis. .

1. Calculate the total cross-sectional area of the beam (we shall call this A). Let x denote the position of the neutral axis from the topmost edge of the top flange of the beam . .

2. Divide the I-beam into rectangles and find the area of these rectangles (we shall denote these areas as A1, A2, and A3 for the top flange, web and bottom flange respectively). Additionally, find the distance from the edge of the top flange to the midsection of these 3 rectangles (these distances will be denoted as x1, x2 and x3) .

.

3. Now, to find the position of the neutral axis, the following general formula must be used:

A*x=  A1*x1       + A2*x2 + A3*x3

We know all the variables in the above formula, except for x (the position of the neutral axis from the top edge of the top flange). So it is just a case of rearranging the formula to find x.

Evaluation of beam deflection and slope

Beam deflection

Static beam equation

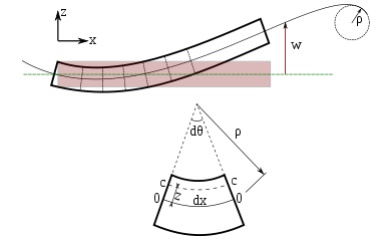
Bending of an Euler-Bernoulli beam. Each cross-section of the beam is at 90 degrees to the neutral axis.

The Euler-Bernoulli equation describes the relationship between the beam's  deflection and the applied load

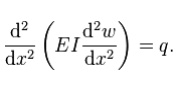
The curve w(x) describes the deflection w of the beam at some position x (recall that the beam is modeled as a one-dimensional object). q is a distributed load, in other words a force per unit length (analogous to  pressure being a force per area); it may be a function of x, w, or other variables.

Note that E is the  elastic modulus and that I is the  second moment of area. I must be calculated with respect to the centroidal axis perpendicular to the applied loading. For an Euler-Bernoulli beam not under any axial loading this axis is called the  neutral axis.

Often, w = w(x), q = q(x), and  EI is a constant, so that:

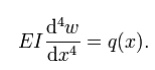
This equation, describing the deflection of a uniform, static beam, is used widely in engineering practice. Tabulated expressions for the deflection wfor common beam configurations can be found in engineering handbooks. For more complicated situations the deflection can be determined by solving the Euler-Bernoulli equation using techniques such as the  "slope deflection method", "moment distribution method", "moment area method,  "conjugate beam method", "the principle of virtual work", "direct integration",  "Castigliano's method", "Macaulay's method" or the  "direct stiffness method".

Successive derivatives of w have important meanings:

The stresses in a beam can be calculated from the above expressions after the deflection due to a given load has been determined.

A number of different sign conventions can be found in the literature on the bending of beams and care should be taken to maintain consistency. In this article, the sign convention has been chosen so the coordinate system is right handed. Forces acting in the positive x and z directions are assumed positive. The sign of the bending moment is chosen so that a positive value leads to a tensile stress at the bottom cords. The sign of the shear force has been chosen such that it matches the sign of the bending moment.

Double integration method

The double integration method is a powerful tool in solving deflection and slope of a beam at any point because we will be able to get the equation of the elastic curve.

Thus, EI / M = 1 / y''

.

Macaulay Method

The starting point for Maucaulay's method is the relation between  bending  moment and  curvature from  Euler-Bernoulli beam theory

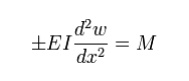
This equation is simpler than the fourth-order beam equation and can be integrated twice to find w if the value of M as a function of x is known. For general loadings, M can be expressed in the form

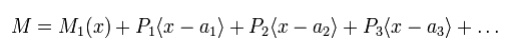
where the quantities P_i(x-a_i) represent the bending moments due to point loads and the quantity (x-a_i) is a  Macaulay bracket defined as

Ordinarily, when integrating P(x - a) we get

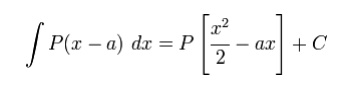
However, when integrating expressions containing Macaulay brackets, we have

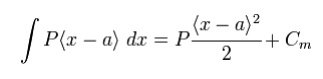
with the difference between the two expressions being contained in the constant C_m. Using these integration rules makes the calculation of the deflection of Euler-Bernoulli beams simple in situations where there are multiple point loads and point moments. The Macaulay method predates more sophisticated concepts such as  Dirac delta functions and  step  functions but achieves the same outcomes for beam problems.

Example: Simply supported beam with point load

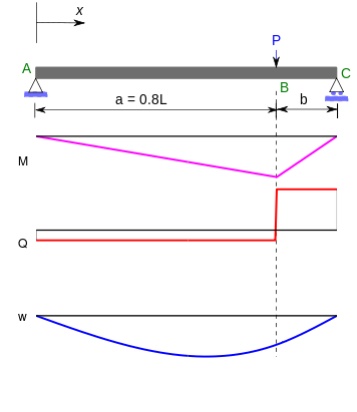
Simply supported beam with a single eccentric concentrated load.

An illustration of the Macaulay method considers a simply supported beam with a single eccentric concentrated load as shown in the adjacent figure. The first step is to find M. The reactions at the supports A and C are determined from the balance of forces and moments as

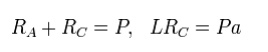
Therefore R_A = Pb / L and the bending moment at a point D between A and B (0 < x < a) is given by

M = R_Ax = Pbx / L

Using the moment-curvature relation and the Euler-Bernoulli expression for the bending moment, we have

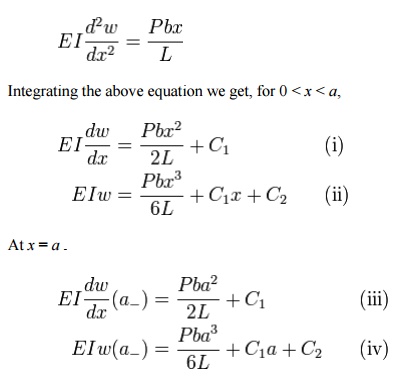
For a point D in the region BC (a < x < L), the bending moment is

M = R_Ax - P(x - a) = Pbx / L - P(x - a)

In Macaulay's approach we use the  Macaulay bracket form of the above expression to represent the fact that a point load has been applied at location B, i.e.,

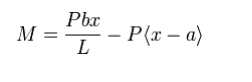
Therefore the Euler-Bernoulli beam equation for this region has the form

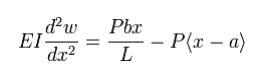
Comparing equations (iii) & (vii) and (iv) & (viii) we notice that due to continuity at point B, C₁ = D₁ and C₂ = D₂. The above observation implies that for the two regions considered, though the equation for  bending moment and hence for the  curvature are different, the constants of integration got during successive integration of the equation for curvature for the two regions are the same.

The above argument holds true for any number/type of discontinuities in the equations for curvature, provided that in each case the equation retains the term for the subsequent region

in the form ( x-a)ⁿ, ( x-b)ⁿ, ( x-c)ⁿ  etc. It should be remembered that for any x, giving the quantities within the brackets, as in the above case, -ve should be neglected, and the calculations should be made considering only the quantities which give +ve sign for the terms within the brackets.

Reverting back to the problem, we have

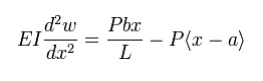
It is obvious that the first term only is to be considered for x < a and both the terms for x > a and the solution is

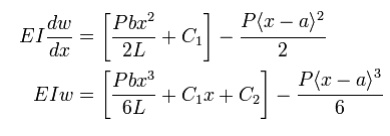
Note that the constants are placed immediately after the first term to indicate that they go with the first term when x < a and with both the terms when x > a. The Macaulay brackets help as a reminder that the quantity on the right is zero when considering points with x < a.

Moment area method

Theorems of Area-Moment Method

Theorem I

The change in slope between the tangents drawn to the elastic curve at any two points A and B is equal to the product of 1/EI multiplied by the area of the moment diagram between these two points.

Theorem II

The deviation of any point B relative to the tangent drawn to the elastic curve at any other point A, in a direction perpendicular to the original position of the beam, is equal to the product of 1/EI multiplied by the moment of an area about B of that part of the moment diagram between points A and B.

Rules of Sign

1.     The deviation at any point is positive if the point lies above the tangent, negative if the point is below the tangent.

2.     Measured from left tangent, if ? is counterclockwise, the change of slope is positive, negative if ? is clockwise.

Columns –End conditions

Columns -end conditions

What is a Column or Strut?

Any machine member, subjected to the axial compressive loading is called a strut and the vertical strut is called column

The columns are generally categorized in two types: short columns and long columns. The one with length less than eight times the diameter (or approximate diameter) is called short column and the one with length more than thirty times the diameter (or approximate diameter) is called long column.

Ideally, the columns should fail by crushing or compressive  stress and it normally happens for the short columns, however, the long columns, most of the times,  failure occurs by buckling.

Euler’s                       Buckling   Formula

To get the correct results, this formula should only be applied for the long columns. The buckling load calculated by the Euler formula is given by:

Fbe = (C*?²*E*I)/

Equivalent length of a column

Strength Of Columns

A stick of timber, a bar of  iron, etc., when used to sustain end loads which act lengthwise of the pieces, are called  columns, posts, or struts if they are so long that they would bend before breaking. When they are so short that they would not bend before breaking, they are called short blocks, and their compressive  strengths are computed by means of equation 1. The strengths of columns cannot, however, be so simply determined, and we now proceed to explain the  method of computing them.

77. End  Conditions. The strength of a column depends in part on the way in which its ends bear, or are joined to other parts of a  structure, that is, on its " end conditions." There are practically but three kinds of end conditions, namely:

1.  "Hinge" or "pin" ends,

2. " Flat" or " square " ends, and

3. "Fixed" ends.

(1)             When a column is fastened to its  support at one end by means of a pin about which the column could rotate if the other end were free, it is said to be "hinged" or "pinned" at the former end. Bridge posts or columns are often hinged at the ends.

(2)             A column either end of which is flat and perpendicular to its axis and bears on other parts of the structure at that  surface, is said to be "flat" or " square" at that end.

(3)             Columns are sometimes riveted near their ends directly to other parts of the structure and do not bear directly on their ends; such are called " fixed ended." A column which bears on its flat ends is often fastened near the ends to other parts of the structure, and such an end is also said to be " fixed." The fixing of an end of a column stiffens and therefore strengthens it more or less, but the strength of a column with fixed ends is computed as though its ends were flat. Accordingly we have, so far as strength is concerned, the following classes of columns:

78. Classes of Columns. (1) Both ends hinged or pinned; (2) one end hinged and one flat; (3) both ends flat.

Other things being the same, columns of these three classes are unequal in strength. Columns of the  first class are the weakest, and those of the third class are the strongest.

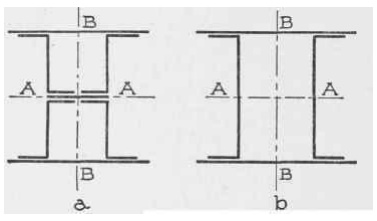
Fig. 46.

70. Cross=sections of Columns. Wooden columns are usually  solid, square, rectangular, or round in section; but sometimes they are "built up" hollow.  Cast-iron columns are practically always made hollow, and rectangular or round in section. Steel columns are made of single rolled shapes - angles, zees,  channels, etc.; but the larger ones are usually "built up" of several shapes. Fig. 46, a, for  example, represents a cross-section of a "Z-bar" column; and Fig. 46, b, that of a "channel" column.

80. Radius of Gyration. There is a quantity appearing in almost all formulas for the strength of columns, which is called "radius of gyration." It depends on the form and extent of the cross-section of the column, and may be defined as follows:

The radius of gyration of any  plane figure (as the section of a column) with respect to any line, is such a length that the square of this length multiplied by the area of the figure equals the  moment of inertia of the figure with respect to the given line.

Thus, if A denotes the area of a figure; I, its moment of inertia with respect to some line; and r, the radius: of gyration with respect to that line; then

(9)

In the column formulas, the radius of gyration always refers to an axis through the center of gravity of the cross-section, and usually to that axis with respect to which the radius of gyration (and moment of inertia) is least. (For an exception, see example 3. Art. 83.) Hence the radius of gyration in this  connection is often called for brevity the "least radius of gyration," or simply the "least radius."

The moment of inertia of the square with respect to the axis is 1/12 a4- Since A = a2, then, by formula 9 above,

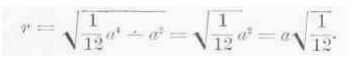
2. Prove that the value of the radius of gyration given for the hollow square in Table A, page 54, is correct.

The value of the moment of inertia of the square with respect to the axis is 1/12 (a4 - a1 4). Since A = a2 - a12,

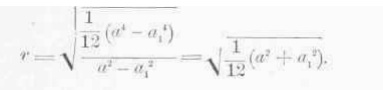
Euler equation

A column under a concentric axial load exhibiting the characteristic deformation of buckling

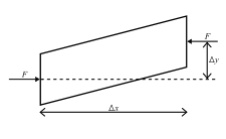
The eccentricity of the axial force results in a bending moment acting on the beam element.

The ratio of the effective length of a  column to the least  radius of gyration of its cross section is called the slenderness ratio (sometimes expressed with the Greek letter lambda, ?). This ratio affords a means of classifying columns. Slenderness ratio is important for design considerations. All the following are approximate values used for convenience.

·        A short  steel column is one whose slenderness ratio does not exceed 50; an intermediate length steel column has a slenderness ratio ranging from about 50 to 200, and are dominated by the strength limit of the material, while a long steel column may be assumed to have a slenderness ratio greater than 200.

·        A short  concrete column is one having a ratio of unsupported length to least dimension of the cross section not greater than 10. If the ratio is greater than 10, it is a long column (sometimes referred to as a slender column).

·         Timber columns may be classified as short columns if the ratio of the length to least dimension of the cross section is equal to or less than 10. The dividing line between intermediate and long timber columns cannot be readily evaluated. One way of defining the lower limit of long timber columns would be to set it as the smallest value of the ratio of length to least cross sectional area that would just exceed a certain constant K of the material. Since K depends on the  modulus of elasticity and the allowable compressive  stress parallel to the grain, it can be seen that this arbitrary limit would vary with the  species of the timber. The value of K is given in most structural handbooks.

If the load on a column is applied through the  center of gravity of its cross section, it is called an  axial load. A load at any other point in the cross section is known as an  eccentric load. A short column under the action of an axial load will fail by direct compression before it buckles, but a long column loaded in the same manner will fail by buckling  (bending), the buckling effect being so large that the effect of the direct load may be neglected. The intermediate-length column will fail by a combination of direct compressive stress and bending.

In 1757,  mathematician Leonhard Euler derived a formula that gives the maximum axial load that a long, slender, ideal column can carry without buckling. An ideal column is one that is perfectly straight, homogeneous, and free from initial stress. The maximum load, sometimes called the critical load, causes the column to be in a state of unstable  equilibrium; that is, the introduction of the slightest lateral force will cause the column to fail by buckling. The formula derived by Euler for columns with no consideration for lateral forces is given below. However, if lateral forces are taken into consideration the value of critical load remains approximately the same.

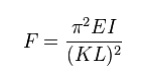
where

F = maximum or critical  force (vertical load on column), E =  modulus of elasticity,

I =  area moment of inertia,

L = unsupported length of column,

K = column effective length factor, whose value depends on the conditions of end support of the column, as follows.

For both ends pinned (hinged, free to rotate), K = 1.0. For both ends fixed, K = 0.50.

For one end fixed and the other end pinned, K = 0.699....

For one end fixed and the other end free to move laterally, K = 2.0. KL is the effective length of the column.

Examination of this formula reveals the following interesting facts with regard to the load-bearing ability of slender columns.

1.      Elasticity and not compressive  strength of the materials of the column determines the critical load.

2.     The critical load is directly  proportional to the  second moment of area of the cross section.

The boundary conditions have a considerable effect on the critical load of slender columns. The boundary conditions determine the mode of bending and the distance between inflection points on the deflected column. The closer together the inflection points are, the higher the resulting capacity of the column.

A demonstration model illustrating the different "Euler" buckling modes. The model shows how the boundary conditions affect the critical load of a slender column. Notice that each of the columns are identical, apart from the boundary conditions.

The strength of a column may therefore be increased by distributing the material so as to increase the moment of inertia. This can be done without increasing the weight of the column by distributing the material as far from the principal axis of the cross section as possible, while keeping the material thick enough to prevent local buckling. This bears out the well-known fact that a tubular section is much more efficient than a solid section for column service.

Another bit of information that may be gleaned from this equation is the effect of length on critical load. For a given size column, doubling the unsupported length quarters the allowable load. The restraint offered by the end connections of a column also affects the critical load. If the connections are perfectly rigid, the critical load will be four times that for a similar column where there is no resistance to rotation (hinged at the ends).

Since the moment of inertia of a surface is its area multiplied by the square of a length called the radius of gyration, the above formula may be rearranged as follows. Using the Euler formula for hinged ends, and substituting A·r² for I, the following formula results.

where F / A is the allowable stress of the column, and l / r is the slenderness ratio.

Since structural columns are commonly of intermediate length, and it is impossible to obtain an ideal column, the Euler formula on its own has little practical application for ordinary design. Issues that cause deviation from the pure Euler strut behaviour include imperfections in geometry in combination with plasticity/non-linear stress strain behaviour of the column's material. Consequently, a number of empirical column formulae have been developed to agree with test data, all of which embody the slenderness ratio. For design, appropriate  safety factors are introduced into these formulae. One such formular is the  Perry  Robertson formula which estimates of the critical buckling load based on an initial (small) curvature. The Rankine Gordon fomular is also based on eperimental results and surgests that a strut will buckle at a load Fmax given by:

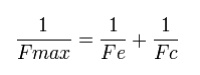
where Fe is the euler maximum load and Fc is the maximum compresive load. This formular typically produces a conservative estimate of Fmax.

Self-buckling

A free-standing, vertical column, with density ?, Young's modulus E, and radius r, will buckle under its own weight if its height exceeds a certain critical height:

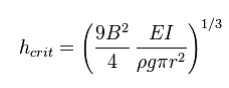
where g is the acceleration due to gravity, I is the  second moment of area of the beam cross section, and B is the first zero of the  Bessel function of the first kind of order -1/3, which is equal to 1.86635...

Slenderness ratio

Euler's Theory : The struts which fail by buckling can be analyzed by Euler's theory. In the following sections, different cases of the struts have been analyzed.

Case A: Strut with pinned ends:

Consider an axially loaded strut, shown below, and is subjected to an axial load „P „P' produces a deflectionAssume„y'that theatends are eitherdi pin jointed or rounded so that there is no moment at either end.

Assumption:

The strut is assumed to be initially straight, the end load being applied axially through centroid.

In this equation „M' is not a functi directly as has been done in the case of deflection of beams by integration method.

Though this equation is in „y' but we maximum or minimum.

So the above differential equation can be arranged in the following form

Let us define a operator

D = d/dx

(D2 + n2) y =0 where n2 = P/EI

This is a second order differential equation which has a solution of the form consisting of complimentary function and particular integral but for the time being we are interested in the complementary solution only[in this P.I = 0; since the R.H.S of Diff. equation = 0]

Thus y = A cos (nx) + B sin (nx)

Where A and B are some constants.

Therefore

In order to evaluate the constants A and B let us apply the boundary conditions,

(i) at x = 0; y = 0

(ii) at x = L ; y = 0

Applying the first boundary condition yields A = 0.

Applying the second boundary condition gives

From the above relationship the least value of P which will cause the strut to buckle, and it is called the " Euler Crippling Load " Pe from which w obtain.

The interpretation of the above analysis is that for all the values of the load P, other than those which make sin nL = 0; the strut will remain perfectly straight since

y = B sin nL = 0

For the particular value of

Then we say that the strut is in a state of neutral equilibrium, and theoretically any deflection which it suffers will be maintained. sensibly constant and in practice slight increase in load at the critical value will cause the deflection to increase appreciably until the material fails by yielding.

Further it should be noted that the deflection is not proportional to load, and this applies to all strut problems; like wise it will be found that the maximum stress is not proportional to load.

The solution chosen of nL = p is just one particular solution; the solutions nL= 2p, 3p, 5p etc are equally valid mathematically and equally valid for modes of buckling of strut different from that of a simple bow. Theoretically therefore, there are an infinite number of values of Pe , each corresponding with a different mode of buckling.

The value selected above is so called the fundamental mode value and is the lowest critical load producing the single bow buckling condition.

The solution nL = 2p produces buckling in two half –waves, 3p in three half-waves etc.

If load is applied sufficiently quickly to the strut, then it is possible to pass through the fundamental mode and to achieve at least one of the other modes which are theoretically possible. In practical loading situations, however, this is rarely achieved since the high stress associated with the first critical condition generally ensures immediate collapse.

Rankine formula for columns

Rankine's formula

One of the most widely known formulae for the design and investigation of  columns employed in engineering practice:

where

=  allowable unit stress for the column

= allowable unit stress for short columns

= a constant

= length

=  radius of gyration in reference to an axis normal to a plane in which  flexure takes

place