Simple Interest

Simple Interest

Selvam said that her sister took a loan from the bank to do her higher studies. The loan money that she borrowed from the bank is known as Sum or Principal. The borrower takes some time period to return the money to the bank. To use the money during a particular period of time, the borrower has to pay an additional amount to the bank. This is known as Interest. So to repay the money borrowed, the borrower has to add the principal and the interest.

That is, Amount = Principal + Interest

Interest is generally given in per cent for a period of 1 year per annum. Suppose the bank gives an amount of ₹ 100 at an interest rate of  ₹ 8, it is written as 8% per year or per annum or in short 8% p.a. (per annum).

It means on every ₹ 100 borrowed, ₹ 8 is the required interest, to be paid for every one year. To understand this let us consider an example.

Selvam takes a loan of ₹ 10000 at 15% per year as rate of interest. Let us find the interest he has to pay at the end of 1 year.

Sum borrowed = ₹ 10000

Rate of interest = 15 % per year

This means if ₹ 100 is borrowed he has to pay ₹ 15 as interest.

So, for the borrowed amount of ₹ 10000, the interest for one year would be

15/100 ×10000 = ₹ 1500

So at the end of 1 year, he has to give an amount of = 10000 + 1500 = ₹ 11500.

Now we can write a general relation to find interest for one year. Take P as the principal or sum and r % as Rate per cent per annum. On every ₹ 100 borrowed the interest paid is ₹ r. Therefore, on ₹ P borrowed the interest paid for 1 year would be [P×r]/100 . If the amount is borrowed for more than 1 year then the interest is calculated for the total period during which the money is kept. This way of calculating interest for the total time period for the same Principal is known as simple interest.

We know that on a Principal of ₹ P at r % rate of interest per year, the interest paid for 1 year is [P×r]/100 . Hence the interest ‘I’ paid for ‘n’ years would be [P × n × r ] /100 or Pnr/100 .

 The amount we have to pay at the end of ‘n’ years is A = P + I .

Example 2.24

Find the simple interest on ₹ 25,000 at 8% per annum for 3 years?

Solution:

Here, the Principal (P) = ₹ 25,000

Rate of interest (r) = 8% per annum

                Time (n) = 3 years

Simple Interest (I) = Pnr/100

                             = [ 25000 × 3 × 8 ] / 100 = 6000

Hence, Simple Interest (I) is ₹ 6,000.

Try these

1. Arjun borrowed a sum of ₹ 5,000 from a bank at 5% per annum. Find the interest and amount to be paid at the end of three year.

Solution:

Here Principal (P) = ₹5000

Rate of interest (r) = 5% per annum

Time (n) = 3 years

Simple Interest I = Pnr/100 = [ 5000 × 3 × 5 ] / 100 = ₹750

Amount to be paid A = P + I = ₹ 5000 + ₹750 = ₹5,750

I = ₹750;

A = ₹5,750 

2. Shanti borrowed ₹ 6,000 from a Bank for 7 years at 12% per annum. What amount will clear off her debt?

Solution:

Here principal (P) = ₹ 6.000

Rate of Interest (r) = 12% per annum

Time (n) = 7 years

Simple Interest (I) = Pnr / 100 = [ 6000 × 7 × 12 ] / 100

I = ₹ 5,040

Amount to be paid A = P + I = 6,000 + 5,040 =  ₹ 11,040 

Example 2.25

Kumaravel has paid simple interest on a certain sum for 2 years at 10% per annum is ₹ 750. Find the sum.

Solution:

Given the rate of interest (r) = 10% per annum

                               Time (n) = 2 years

We know that Simple Interest (I) = Pnr/100

                                             750 = P×2×10 /100

Therefore, Principal (P) = (750 × 100) / (2×10) = 3750

Therefore, Kumaravel has borrowed a sum of ₹ 3,750.

Example 2.26

In what time will ₹ 5,600 amount to ₹ 6,720 at 6% per annum?

Solution:

Principal (P) = ₹ 5,600

Rate (r) = 6% per annum

Amount = ₹ 6,720

Amount = principal + interest

Interest = Amount – Principal

             = 6720 − 5600 = 1120

We know that Simple Interest (I) = Pnr/100

1120 = [6720 × 6 × n] /100

  Therefore, n = [1120 ×100] / [5600 × 6] = 3 (1/3)  = 3 years.

Example 2.27

Sathish kumar borrowed ₹ 52,000 from a money lender at a particular rate of simple interest. After 4 years, he paid ₹ 79,040 to settle his debt. At what rate of interest he borrowed the money?

Solution:

Principal (P) = ₹ 52,000

Time (n) = 4 years

Interest = Amount – Principal

            =79,040 − 52,000 = 27,040

We find the Simple Interest (I) = Pnr/100

Therefore, 27040 =  [52000 × r × 4] / [100]

Rate of interest he borrowed (r) = [27040 ×100] / [52000 × 4] =13%.

Example 2.28

 A sum of ₹ 46,000 was lent out at simple interest and at the end of 1 year and 9 months, the total amount was ₹ 52,440. Find the rate of interest per year.

Solution:

A =P+I

I =A–P

= 52440 – 46000

= ₹ 6,440

r = ?

Example 2.29

A principal becomes ₹ 10,050 at the rate of 10% in 5 years. Find the principal.

Solution:

A = ₹ 10,050

n = 5 years

r =10% 

P = ?

For calculating principal with the given data, we proceed as follows. We know that,

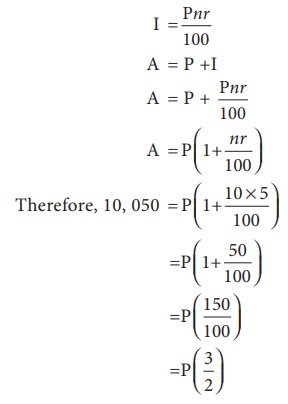
Therefore, P = 10,050 × (2/3) =3350

Hence, Principal = ₹ 3,350.

Think

In simple interest, a sum of money doubles itself in 10 years. In how many years it will get triple itself.

Solution:

Let the Principal be P and Rate of interest be r'% per annum.

Here the number of years n = 10 years

Given in 10 years P becomes 2 P

A = P + I

After 2 years A = 2P

i.e. 2P = P +1

2P - P = I

P = P×n×r  / 100

P = P×10×r  / 100

 r = P×100 / P×10

 r = 10%

Now if the amount becomes triple then A = P + I = 3P

3P = P + I

3P - P = I

2P = I

 2P = [ P×n×10 ] / 100

[2P × 100] / [ P × 10 ] = n

 n = 20 years

After 20 years the amount get tripled.