Definition, Theorem, Solved Example Problems | Mathematics - Resolution of Vectors | 11th Mathematics : UNIT 8 : Vector Algebra I
Chapter: 11th Mathematics : UNIT 8 : Vector Algebra I
Resolution of Vectors
Resolution of Vectors
Resolution of a vector can be done for any finite dimension. But we will discuss only in two and three dimensions. Let us start with two dimension.
Resolution of a vector in two dimension
Theorem 8.5
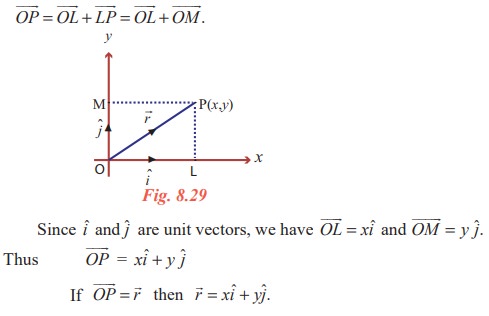
Let iˆ and ˆj be the unit vectors along the positive x-axis and the y-axis having initial point at the origin O. Now ![]() is the position vector of any point P in the plane. Then
is the position vector of any point P in the plane. Then ![]() can be uniquely written as
can be uniquely written as
![]() = xiˆ + y jˆ for some real numbers x and y. Further
= xiˆ + y jˆ for some real numbers x and y. Further 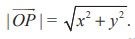
Proof
Let (x, y) be the coordinates of the point P. Let L and M be the foots of the perpendiculars drawn from P to the x and y axes. Then
To prove the uniqueness, let x1iˆ + y1 ˆj and x2 iˆ + y2 ˆj be two representations of the same point P. Then
x1iˆ + y1jˆ = x2iˆ + y2jˆ .
This implies that
(x1 − x2 )iˆ − ( y2− y1 ) ˆj = ![]() ⇒ x1 − x2 = 0, y2 − y1 = 0.
⇒ x1 − x2 = 0, y2 − y1 = 0.
In other words x1 = x2 and y1 = y2 and hence the uniqueness follows.
In the triangle OLP, OP2 = OL2 + LP2 ;
hence | ![]() |= √[ x2 + y2 ].
|= √[ x2 + y2 ].
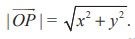
That is, 
Observe that if iˆ + jˆ are the unit vectors in the postive directions of x and y axes, then the iˆ and jˆ position vector of the point (6,4) can be written as 6iˆ + 4 jˆ and this is the only way of writing it.
Result 8.8
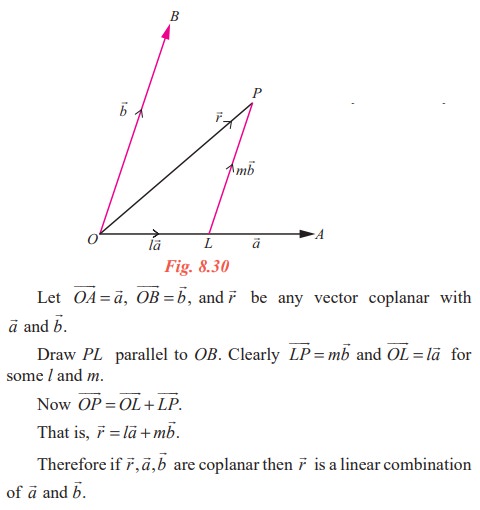
If ![]() and
and ![]() are two non-collinear vectors in a plane, then any vector in the plane can be written as the linear combination of
are two non-collinear vectors in a plane, then any vector in the plane can be written as the linear combination of ![]() and
and ![]() in a unique way. That is, any vector in the plane is of the form l
in a unique way. That is, any vector in the plane is of the form l![]() + m
+ m![]() for some scalars l and m.
for some scalars l and m.
Proof
Note 8.2
Further if three non collinear vectors are coplanar then any one of the vector can be written as a linear combination of other two. Note that the converse is also true.
Result 8.9
If ![]() ,
, ![]() and
and ![]() are three non-coplanar vectors in the space, then any vector in the space can be written as l
are three non-coplanar vectors in the space, then any vector in the space can be written as l ![]() + m
+ m![]() + n
+ n![]() in a unique way for some scalars l, m and n.
in a unique way for some scalars l, m and n.
Definition 8.15
Let iˆ and ˆj be the unit vectors in the positive directions of x and y axes respectively. Let ![]() be any vector in the plane. Then
be any vector in the plane. Then ![]() = xiˆ + y ˆj for some real numbers x and y. Here xiˆ and y ˆj are called the rectangular components of
= xiˆ + y ˆj for some real numbers x and y. Here xiˆ and y ˆj are called the rectangular components of ![]() along the x and y axes respectively in two dimension.
along the x and y axes respectively in two dimension.
What we discussed so far can be discussed in the three dimensional space also.
Resolution of a vector in three dimension
Theorem 8.6
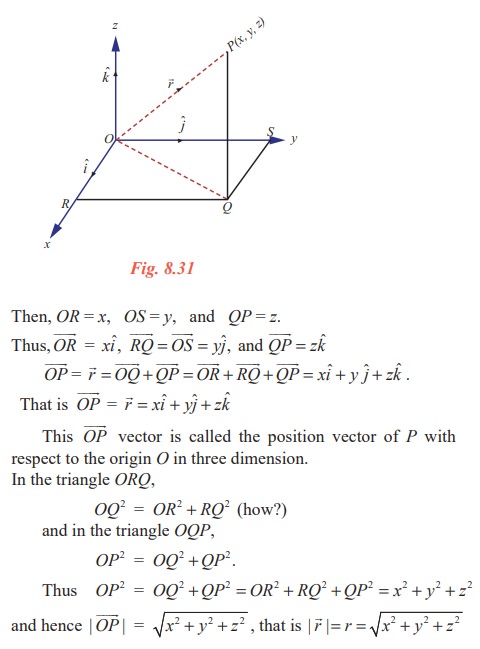
Let iˆ, ˆj and kˆ be the unit vectors in the direction of postive x, y and z axes respectively having initial point at the origin O. Let ![]() be the position vector of any point P in the space. Then
be the position vector of any point P in the space. Then ![]() can be uniquely written as
can be uniquely written as ![]() = xiˆ + y ˆj + zkˆ for some real numbers x, y and z. Further |
= xiˆ + y ˆj + zkˆ for some real numbers x, y and z. Further | ![]() | = √ [ x 2+ y 2 + z2 ].
| = √ [ x 2+ y 2 + z2 ].
Proof
Let (x, y, z) be the coordinates of the point P. Let Q be the foot of the perpendicular drawn from P to the xy-plane. Let R and S be the foots of the perpendiculars drawn from Q to the x and y axes respectively. Let ![]() =
= ![]() .
.
Then, OR = x, OS = y, and QP = z. z
Components of vector joining two points
Let us find the components of the vector joining the point (x1 , y1 ) to (x2 , y2 ).
Let A and B be the points (x1 , y1 ) and (x2 , y2 ). Let P be the point (x2 − x1 , y2 − y1 ). Then ![]() =
=![]() .
.
The components of ![]() are (x2 − x1 )iˆ and ( y2 − y ) jˆ. Hence the components of
are (x2 − x1 )iˆ and ( y2 − y ) jˆ. Hence the components of ![]() in the directions of x and y axes are (x2 − x1 )iˆ ( y2 − y1 ) jˆ.
in the directions of x and y axes are (x2 − x1 )iˆ ( y2 − y1 ) jˆ.
Similarly if A and B are the points (x1 , y1 , z1 ) and (x2 , y2 , z2 ), then the components of ![]() in the directions of x, y and z axes are (x2 − x1)iˆ ( y2 − y1 ) jˆ (z2 − z1 )kˆ .
in the directions of x, y and z axes are (x2 − x1)iˆ ( y2 − y1 ) jˆ (z2 − z1 )kˆ .
Matrix representation of a vector
A vector with three components can be visualised as either a row or column matrix as [x, y, z] or ![]() respectively.
respectively.
Thus any vector ![]() = a1iˆ + a2 jˆ + a3kˆ can be obtained from
= a1iˆ + a2 jˆ + a3kˆ can be obtained from
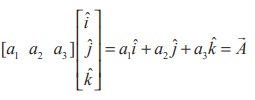
Hence addition of vectors and multiplication of a vector by a scalar can be defined as follows.
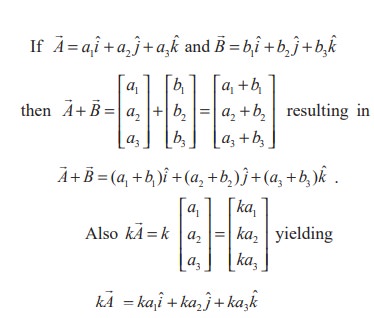
k![]() = ka1iˆ + ka2 ˆj + ka3 kˆ
= ka1iˆ + ka2 ˆj + ka3 kˆ
For k ∈ R, k > 1 yields magnification, 0 < k < 1 yields contraction of a vector and k = 0 yields a zero vector ![]() = 0iˆ + 0 ˆj + 0kˆ =
= 0iˆ + 0 ˆj + 0kˆ = ![]() .
.
Result 8.10
Using the commutative, associative properties of vector addition and the distributive property of the scalar multiplication we can prove the following.

Example 8.4
Find a unit vector along the direction of the vector 5iˆ − 3 ˆj + 4kˆ.
Note 8.3
Now we have another unit vector parallel to 5iˆ − 3 ˆj + 4kˆ in the opposite direction. That is,
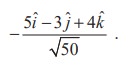
Related Topics