Odds - Probability Theory

ODDS

The word odds is frequently used in probability and statistics. Odds relate the chances in favour of an event A to the chances against it. Suppose a represents the number of ways that an event can occur and b represents the number of ways that the event can fail to occur.

The odds of an event A are a : b in favour of an event and

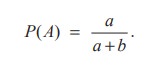
Further, it may be noted that the odds are a : b in favour of an event is the same as to say that the odds are b : a against the event.

If the probability of an event is p , then the odds in favour of its occurrence are p to (1 − p) and the odds against its occurrence are (1 − p) to p .

Illustration 12.7

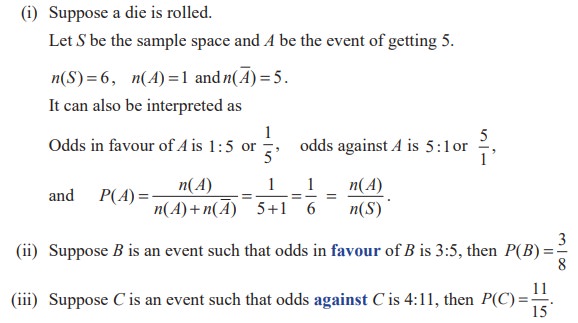
Example 12.11

A man has 2 ten rupee notes, 4 hundred rupee notes and 6 five hundred rupee notes in his pocket. If 2 notes are taken at random, what are the odds in favour of both notes being of hundred rupee denomination and also its probability?

Solution

Let S be the sample space and A be the event of taking 2 hundred rupee note.

Therefore, n(S) =12c2 =66, n(A) = 4c2=6 and n()=66-6=60

Therefore, odds in favour of A is 6: 60

That is, odds in favour of A is 1: 10, and P(A) 1/11.

EXERCISE 12.1

(1) An experiment has the four possible mutually exclusive and exhaustive outcomes A, B, C, and D. Check whether the following assignments of probability are permissible.

(i) P(A) = 0.15, P(B) = 0.30, P(C) = 0.43, P(D)= 0.12

(ii) P(A) = 0.22, P(B) = 0.38, P(C) = 0. 16, P (D) = 0.34

(iii) P(A) = 2/5 , P(B) = 3/5 , P(C) = − 1/5 , P(D) = 1/5

(2) If two coins are tossed simultaneously, then find the probability of getting

(i) one head and one tail (ii) at most two tails

(3) Five mangoes and 4 apples are in a box. If two fruits are chosen at random, find the probability that (i) one is a mango and the other is an apple (ii) both are of the same variety.

(4) What is the chance that (i) non-leap year (ii) leap year should have fifty three Sundays?

(5) Eight coins are tossed once, find the probability of getting

(i) exactly two tails (ii) at least two tails (iii) at most two tails

(6) An integer is chosen at random from the first 100 positive integers. What is the probability that the integer chosen is a prime or multiple of 8?

(7) A bag contains 7 red and 4 black balls, 3 balls are drawn at random. Find the probability that (i) all are red (ii) one red and 2 black.

(8) A single card is drawn from a pack of 52 cards. What is the probability that

(i) the card is an ace or a king

(ii) the card will be 6 or smaller

(iii) the card is either a queen or 9?

(9) A cricket club has 16 members, of whom only 5 can bowl. What is the probability that in a

team  of 11 members at least 3 bowlers are selected?

(10) (i) The odds that the event A occurs is 5 to 7, find P(A).

(ii) Suppose P(B) = 2/5 . Express the odds that the event B occurs.