Exponents and Radicals

Exponents and Radicals

First we shall consider exponents.

1. Exponents

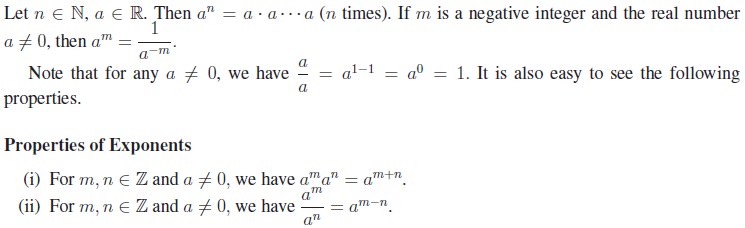
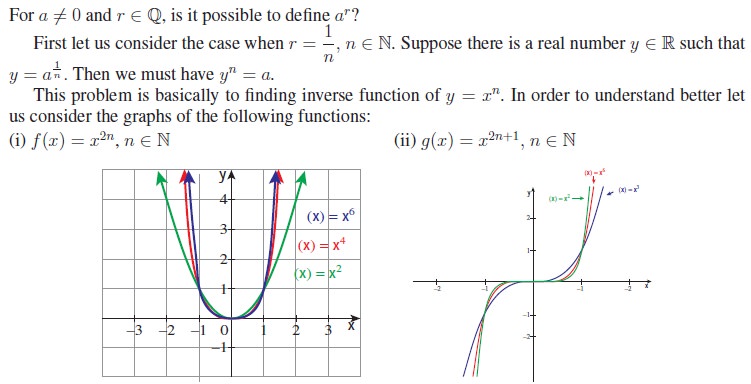
2. Radicals

Question:

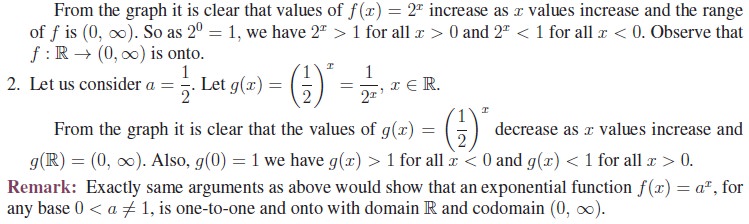
3. Exponential Function

Properties of Exponential Function

A Special Exponential Function

Among all exponential functions, f(x) = e^x, x ∈ R is the most important one as it has applications in many areas like mathematics, science and economics. Then what is this e? The following illustration from compounding interest problem leads to the constant e.

Illustration

Compound Interest

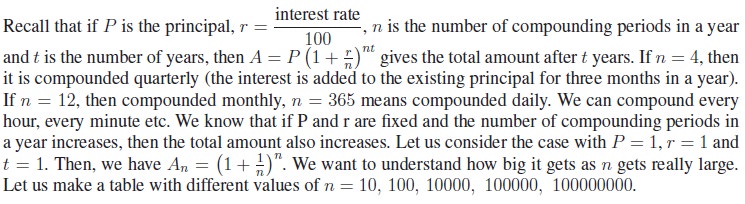
We notice that as n gets really large, A_n values seem to be getting closer to 2.718281815..... Actually A_n values approach a real number e, an irrational number. 2.718281815 is an approximation to e. So the compound interest formula becomes A = P e^rt, where r is the interest rate and P is the principal and t is the number of years. This is called Continuous Compounding.