Exercise 6.5: Multiple choice questions

Trigonometry

Multiple choice questions

1. If sin30º = x and cos60º = y , then x² + y² is

(1) 1/2

(2) 0

(3) sin90°

(4) cos90°

Solution:

x = sin 30° = 1/2

y = cos 60° = 1/2

x²+y² = (1/2)²+(1/2)² = ¼ + ¼ = 1/2

[Answer: (1) 1/2]

2. If tan θ cot 37º, then the value of θ is

(1) 37°

(2) 53°

(3) 90°

(4) 1°

Solution:

tan θ = cot 37°

tan (90° - 37°) = cot 37°

θ = 90°-37° = 53°

[Answer: (2) 53°]

3. The value of tan 72° tan18° is

(1) 0

(2) 1

(3) 18°

(4) 72°

Solution:

The value of tan 72° tan 18° = tan (90 - 18°). tan 18°

= cot 18° . tan 18 = [1/tan 18°] × [tan 18°] = 1

[Answer: (2) 1]

4. The value of [2 tan 30º ] / [1 - tan²30º ] is equal to

(1) cos60°

(2) sin60°

(3) tan60°

(4) sin30°

Solution:

2 tan 30° / 1 - tan² 30°

[Answer: (3) tan60°]

5. If 2sin 2θ = √3 , then the value of θ is

 (1) 90°

(2) 30°

(3) 45°

(4) 60°

Solution:

Solution:

2 sin 2θ = √3

sin 2θ = √3 / 2

= sin 60°

2θ = 60° ⇒ θ = 30°

[Answer: (2) 30°]

6. The value of 3sin 70° sec 20° + 2 sin 49° sec 51° is

(1) 2

(2) 3

(3) 5

(4) 6

Solution:

3 sin 70°. sec 20° + 2 sin 49° sec 51°

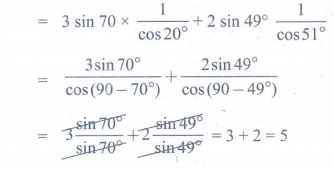
[Answer: (3) 5]

7. The value of [1 - tan²45°] / [1 + tan²45°] is

(1) 2

(2) 1

(3) 0

(4) 1/2

Solution:

[1 - tan²45°] / [1 + tan²45°] = 1-1² / 1+1² = 0/2 = 0

[Answer: (3) 0]

8. The value of cosec(70°+θ) - sec(20° - θ) + tan(65° + θ) - cot(25° - θ) is

(1) 0

(2) 1

(3) 2

(4) 3

Solution:

cosec (70° + 0) - sec (20° - 0) + tan (65° + 0) - cot (25° - 0)

[Answer: (1) 0]

9. The value of tan 1° tan 2° tan 3° ... tan 89° is

(1) 0

(2) 1

(3) 2

(4) √3/2

Solution:

tan (90° - 89°) tan (90° - 88°) ...tan (90° - 46°) tan 45° tan 46°... tan 88 tan 89°

= cot 89° cot 88°... cot 46° (1) tan 46°... tan 88° tan 89° = 1

[Answer: (2) 1]

10. Given that sin α = 1/2 and cos β = 1/2, then the value of α + β is

(1) 0°

(2) 90°

(3) 30°

(4) 60°

Solution:

sin α = 1/2, cos β = 1/2, α + β = 90°

[Answer: (2) 90°]