Differentiator

Differentiator:

One of the simplest of the op-amp circuits that contains capacitor in the differentiating amplifier.

Differentiator:

As the name implies, the circuit performs the mathematical operation of differentiation (i.e) the output waveform is the derivative of the input waveform. The differentiator may be constructed from a basic inverting amplifier if an input resistor R₁ is replaced by a capacitor C₁ .

The expression for the output voltage can be obtained KCL eqn written at node V₂ as follows,

Since the differentiator performs the reverse of the integrator function.

Thus the output V₀ is equal to R_F C₁ times the negative rate of change of the input voltage V_in with time.

The –sign => indicates a 180⁰ phase shift of the output waveform V₀ with respect to the input signal.

The below circuit will not do this because it has some practical problems.

The gain of the circuit (R_F /XC₁ )R with R in frequency at a rate of 20dB/decade. This makes the circuit unstable.

Also input impedance XC₁ S with R_in frequency which makes the circuit very susceptible to high frequency noise.

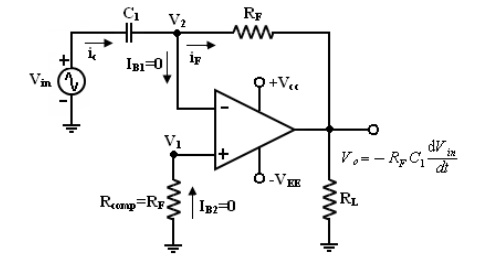
Basic Differetntiator

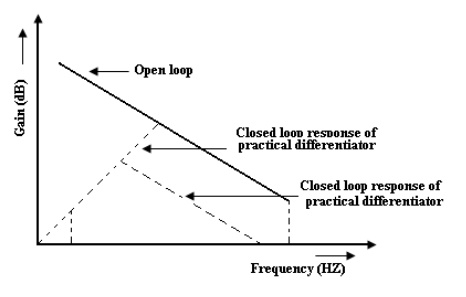
From the above fig, f_a = frequency at which the gain is 0dB and is given by, Both stability and high frequency noise problems can be corrected by the addition of 2 components. R₁ and C_F . This circuit is a practical differentiator.

From Frequency f to feedback the gain R_s at 20dB/decade after feedback the gain S at 20dB/decade. This 40dB/ decade change in gain is caused by the R₁ C₁ and R_F C_F combinations. The gain limiting frequency fb is given by,

Where R₁ C₁ = R_F C_F

R₁ C₁ and R_F C_F   => helps to reduce the effect of high frequency input, amplifier noise and offsets.

All R₁ C₁ and R_F C_F make the circuit more stable by preventing the R_in gain with frequency.

Generally, the value of Feedback and in turn R1 C1 and RF CF values should be selected such that

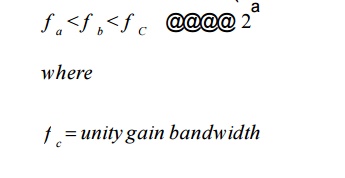
The input signal will be differentiated properly, if the time period T of the input signal is larger than or equal to R_F C₁ (i.e) T > R_F C₁

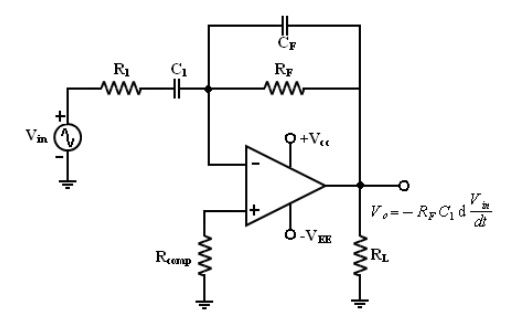
Practical Differentiator

A workable differentiator can be designed by implementing the following steps.

1. Select fa equal to the highest frequency of the input signal to be differentiated then assuming a value of C₁< 1μf. Calculate the value of R_F .

2. Choose fb = 20fa and calculate the values of R₁ and C_F so that R₁ C₁ = R_FC_F .

Uses:

Its used in waveshaping circuits to detect high frequency components in an input signal and also as a rate of change and detector in FM modulators.

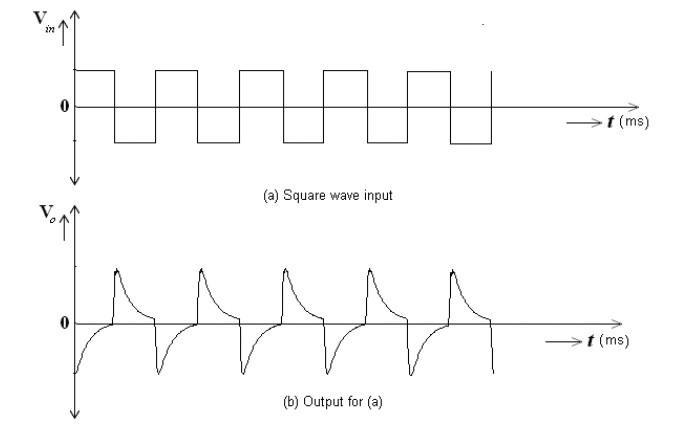
This o/p for practical differentiator