Chapter: 11th Statistics : Chapter 9 : Random Variables and Mathematical Expectation
Addition and Multiplication Theorem on Expectations
Addition
and Multiplication Theorem on Expectations
Addition Theorem on Expectations
1. Statement for Discrete random variable
If
X and Y are two discrete random variables
then
(X+Y)
= E(X) + E(Y)
Proof
Let the random variable X
assumes the values x1, x2 ... xn with corresponding probabilities p1, p2…..pn, and the random variable y
assume the values y1, y2 ……. ym with corresponding probabilities p1, p2…..pm

2. Statement for Continuous random variable
Let X and Y are two continuous random variables
with probability density functions f(x) and f(y) respectively. Then E (X
+ Y) = E(X) + E(Y)
Proof:
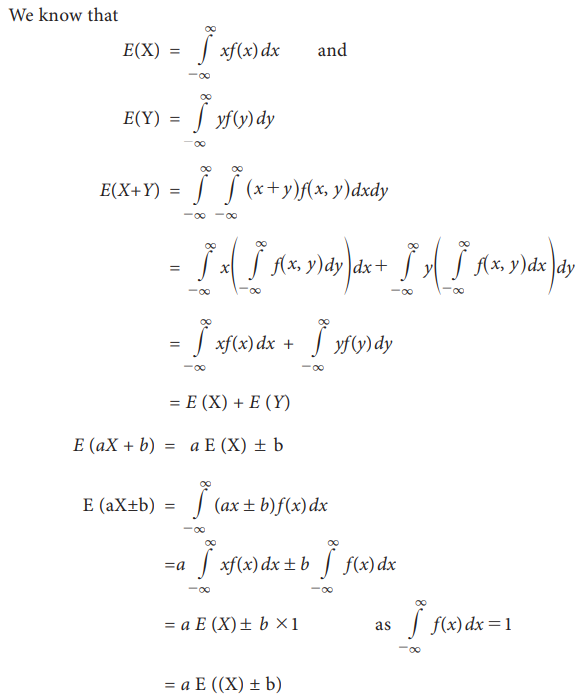
Remarks:
1. Statement: (aX+b) = aE(X)+b where a and b are constants.
Proof: E(aX+b)
= E(aX)+E(b) by property 3
=
aE(X)+b by property 2
(aX-b) = aE(X)-b
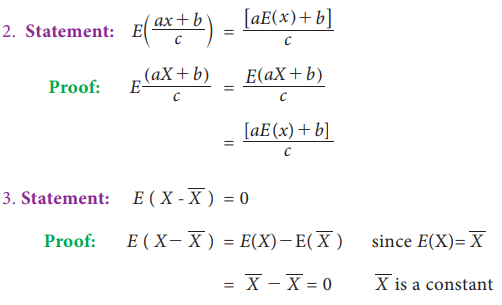
Example 9.23
Find
the expectation of the sum of the number obtained on throwing two dice.
Solution:
Let
X&Y denote the number obtained on the
I and II die respectively. Then each of them is a random variable which takes
the value 1,2,3,4,5 and 6 with equal probability 1/6.

Thus
the expectation of the numbers obtained on two dices.
X+Y takes the values 2, 3…12 with
their probability given by
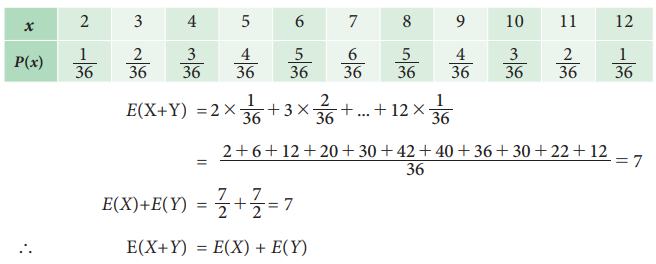
Example 9.24
Let
X and Y are two random variables with
p.d.f given by
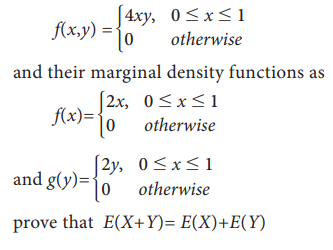
Solution:
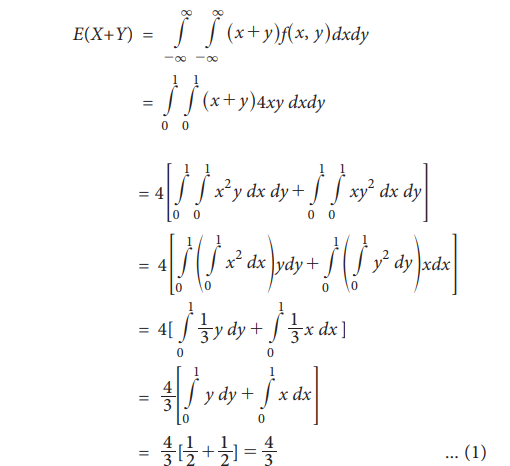
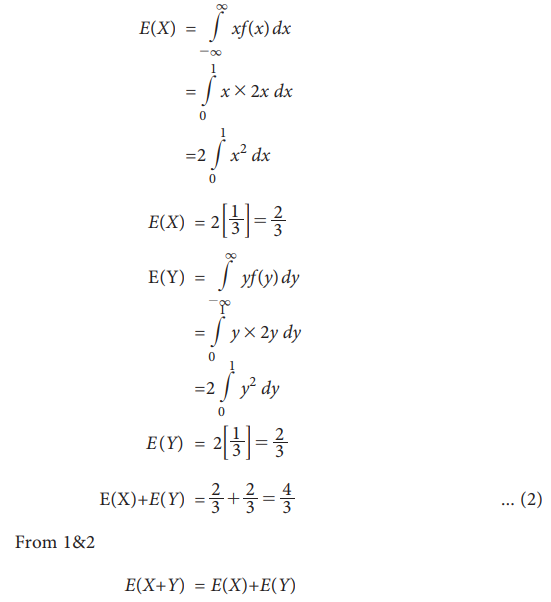
Multiplication Theorem on Expectation
Discrete random variable:
Statement:
If X and Y are two independent variables
then E(XY) = E (X) E(Y)
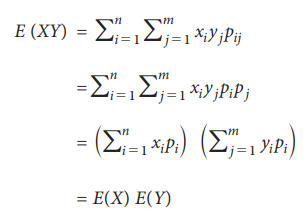
Continuous random variable:
Statement:
If X and Y are independent random variables
Then E (XY) = E(X) E(Y)
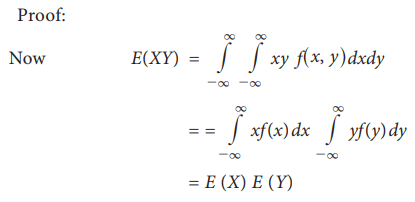
Example 9.25
Two
coins are tossed one by one. First throw is considered as X
and second throw is considered as Y following joint probability
distribution is given by,

[getting
Head is taken as 1 and Tail is taken as 0]
Verify
E(XY)= E(X) E(Y)
Solution:
A
random variable XY can take the values 0 and 1

[It is applicable only when X
and Y are independent]
Example 9.26
The independent random variables X and Y have the p.d.f
given by
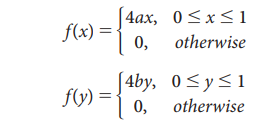
Prove that
E(XY) = E(X) E(Y)
Solution:
X and Y are independent
f(x,y) = f(x)
× f(y)
f(x,y) = 4ax×4by
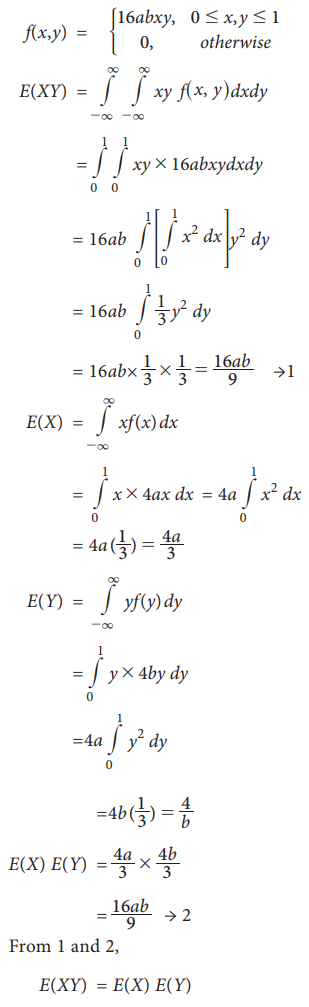
Related Topics