Systematic Sampling

Systematic Sampling

In systematic sampling, the population units are numbered from 1 to N in ascending order. A sampling interval, denoted by k, is determined as k=N/n, where n denotes the required sample size. Then n–1 such sampling intervals each consisting of k units will be formed. A number is selected at random from the first sampling interval. Let it be number i where i ≤ k. This number is the random starting point for the whole selection of the sample. The unit corresponding to i is the first unit in the sample. The subsequent sampling units are the units in the following positions:

i, k +i, 2k +i, 3k + i , …, nk

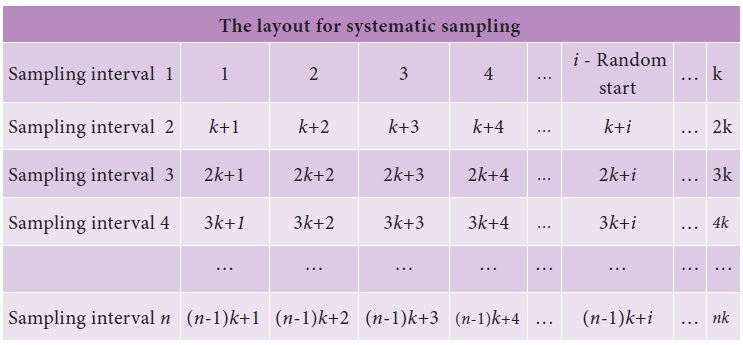
Thus, with selection of the first unit, the whole sample is selected automatically. As the first unit could have been any of the k units, the technique will generate k systematic samples with equal probability. If N is not an integral multiple of n, then sizes of a few possible systematic samples may vary by one unit.

Merits:

·           This method is simple and convenient.

·           Less time consuming.

·           It can be used in infinite population.

Limitation:

·           Since it is a quasi random sampling, the sample may not be a representative sample.

Example 2.3

Suppose a systematic random sample of size n = 10 is needed from a population of size N = 200, the sampling interval k=N/n=200/10=20.

The first sampling interval consists of numbers 1 to 20. If the randomly selected number (random starter) is 7, the systematic sample will consist units corresponding to positions 7, 27,47,67,87,107,127,14 7,167,187.

Applications:

·           Systematic sampling is preferably used when the information is to be collected from trees in a highway, houses in blocks, etc,.

·           This method is often used in industry, where an item is selected for testing from a production line (say, every fifteenth item in the order of production) to ensure that equipments are working satisfactorily.

·           This technique could also be used in a sample survey for interviewing people. A market researcher might select every 10th person who enters a particular store, after selecting a person at random as a random start.