SET - Game

SET - Game

Any game that uses features can be used to stimulate logical thinking and it provides an interesting and challenging context for exploring ideas in discrete mathematics.

Now, let us learn about a SET game

A SET game proves to be an excellent extension for activities that involve organizing the objects by attributes. The SET game builds the cognitive, logical, spatial reasoning as well as visual perception skills.

The SET game is a puzzle that uses cards which have four features on them. They are shapes, colours, shades and the number of shapes.

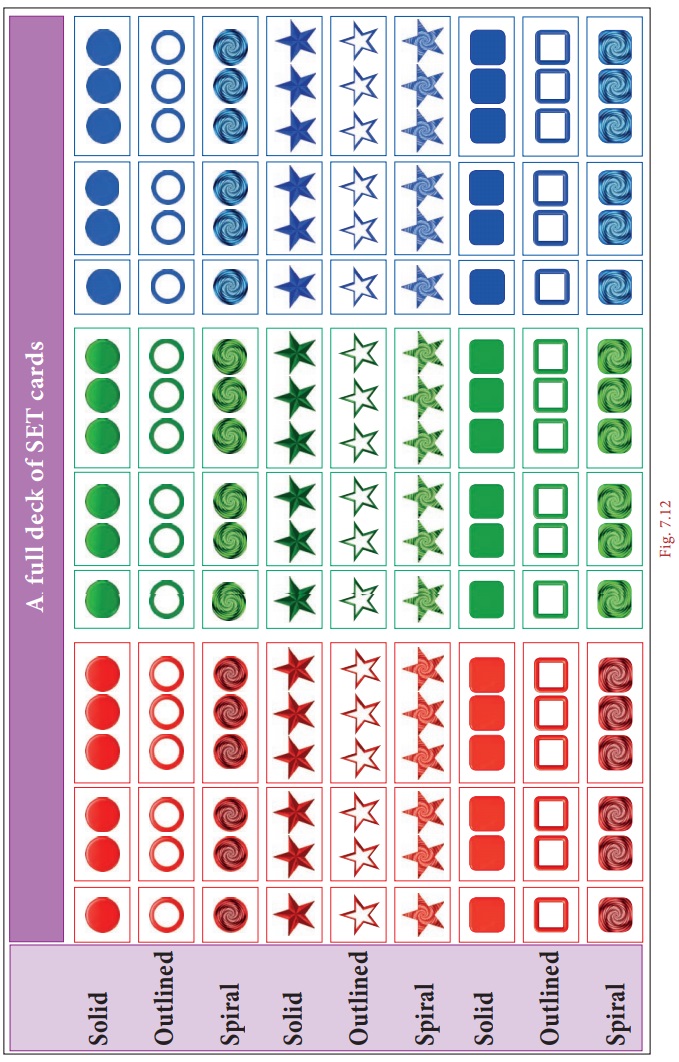
In one full deck of SET cards, there are 3 different shapes: circle, star, square and in 3 different colours: red, green, blue. 3 Each of these 9 cards (3 shapes × 3 colours) have 3 different shades: solid, outlined, spiral and also they can be paired 3 with different numbers: one, two and three. So there are totally 81 cards (3 shapes × 3 colours × 3 shades × 3numbers) in the deck as shown in Fig. 7.12.

A SET which consists of three cards should satisfy all the four following conditions:

(i) All the three cards have the same shape or have three different shapes.

(ii) All the three cards have the same colour or have three different colours.

(iii) All the three cards have the same shade or have three different shades.

(iv) All the three cards have the same number or have three different numbers.

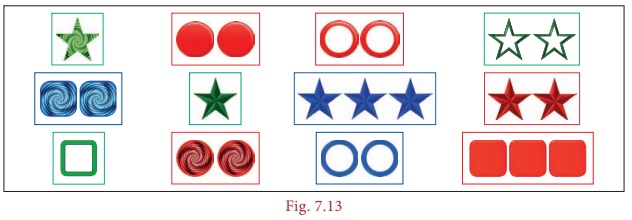
Situation:

The teacher displays 12 cards as shown in Fig. 7.13 and explains how to form a SET using these 2 cards  and  taken from them. Now, follow the step by step procedure to figure out the third card to complete this SET is as follows.

Remember, a SET consists of 3 set of cards.

STEP 1: If you look at the shape then, one is star  and the other one is also star.  These two cards have the same shape. So, the last card also should have the same shape. 

STEP 2: If you look at the colour then, one is green  and the other is red  These two cards have different colours. So, the last card also should have a different colour that is blue. 

STEP 3: If you look at the shade then, one is solid  and the other is also solid  These two cards have the same shade. So, the last card also should have the same shade. 

STEP 4: If you look at the number then, one star card is green solid  and the other has two star red solid cards  So, the last card also should have a different number three blue cards.  Therefore these three set of cards have different numbers and different colours with same shape and shade.

Finally, 

Shape : All same ✓ Colour : All different✓

Shade : All same ✓ Number : All different ✓

Now, this completes the rules for a SET.

Now, the teacher asks the students to find two more SETs from Fig.7.13. Let us check the SET again.

1. 

Shape : All same ✓ Colour : All same ✓

Shade : All different ✓ Number : All same ✓

Yes, This is a SET.

2. 

Shape : All different ✓ Colour : All different ✓

Shade : All different ✓ Number : All different ✓

Yes, This is a SET.

Again , the teacher makes an arrangement of cards and asks them to check whether, it forms a SET?

3. 

Shape : All same or all different × Colour : All same or all different ×

Shade : All same or all different × Number : All same or all different ×

No, this is not a SET.

Since, the above SET does not complete the SET rule.

Thus,we come to know a SET consists of 3 set of cards in which each individual feature is either all same on each card or all different on each card.

Activity

Choose the correct card to complete the perfect SET. One is done for you.

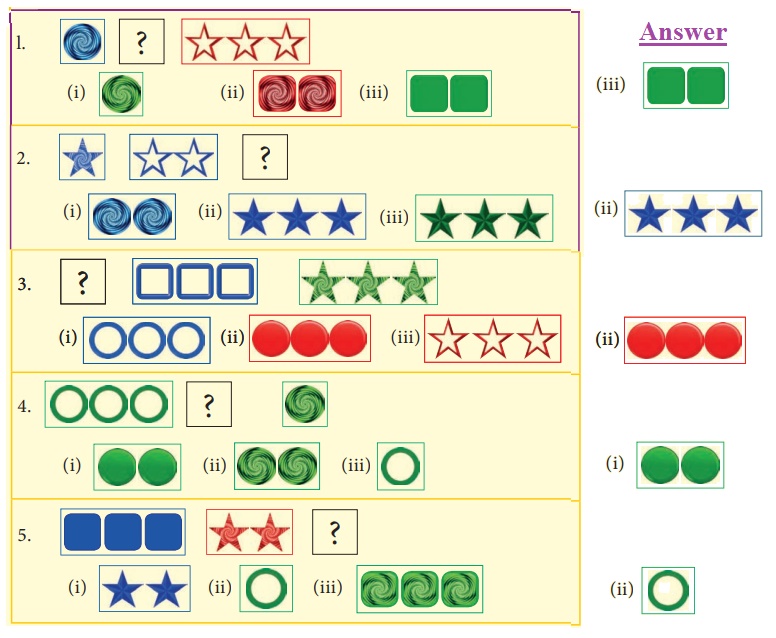
1. Answer: (iii) ; 2. Answer: (ii) ; 3. Answer: (ii) ; 4. Answer: (i) ; 5. Answer: (ii)

Try these

1. Find any five SETs among these set of cards (repetition of cards allowed).

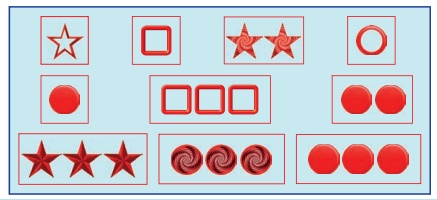
Solution:

(i) (a), (c), (h)

(ii) (e), (g), (j)

(iii) (a), (b), (d) 

(iv) (f), (i), (j) 

(v) (f), (i), (h) 

2. This is an example for a magic square in SETs. Can you make another two?

Solution:

Repeat one square with every thing 2 another square with everything 3.