Chapter: Medical Physiology: Physical Principles of Gas Exchange; Diffusion of Oxygen and Carbon Dioxide Through the Respiratory Membrane
Physics of Gas Diffusion and Gas Partial Pressures
Physics of Gas Diffusion and Gas Partial Pressures
Molecular Basis of Gas Diffusion
All the gases of concern in respiratory physiology are simple molecules that are free to move among one another, which is the process called “diffusion.” This is also true of gases dissolved in the fluids and tissues of the body.
For diffusion to occur, there must be a source of energy. This is provided by the kinetic motion of the molecules themselves. Except at absolute zero temperature, all molecules of all matter are continually undergoing motion. For free molecules that are not physically attached to others, this means linear movement at high veloc-ity until they strike other molecules. Then they bounce away in new directions and continue until striking other molecules again. In this way, the molecules move rapidly and randomly among one another.
Net Diffusion of a Gas in One Direction—Effect of a Concentration Gradient. If a gas chamberor a solution has a high concentration of a particular gas at one end of the chamber and a low concentration at the other end, as shown in Figure 39–1, net diffusion of the gas will occur from the high-concentration area toward the low-concentration area. The reason is obvious: There are far more molecules at end A of the chamber to diffuse toward end B than there are molecules to diffuse in the opposite direc-tion. Therefore, the rates of diffusion in each of the two directions are proportion-ately different, as demonstrated by the lengths of the arrows in the figure.

Gas Pressures in a Mixture of Gases—“Partial Pressures” of Individual Gases
Pressure is caused by multiple impacts of moving molecules against a surface. There-fore, the pressure of a gas acting on the surfaces of the respiratory passages and alveoli is proportional to the summated force of impact of all the molecules of that gas striking the surface at any given instant. This means that the pressure is directlyproportional to the concentration of the gas molecules.
In respiratory physiology, one deals with mixtures of gases, mainly of oxygen, nitrogen, and carbon dioxide.
The rate of diffusion of each of these gases is directly proportional to the pressure caused by that gas alone, which is called the partial pressure of that gas. The concept of partial pressure can be explained as follows.
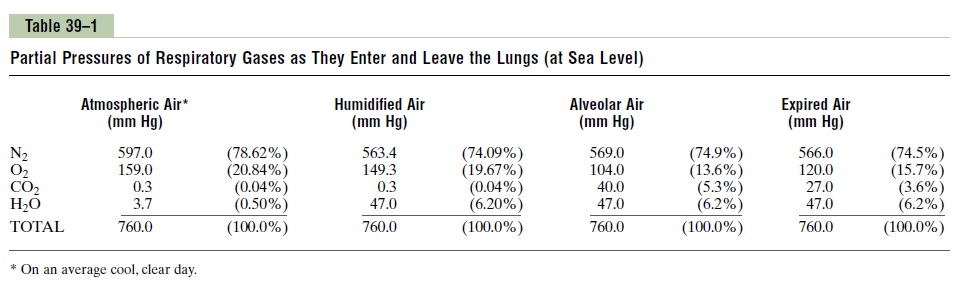
Consider air, which has an approximate composition of 79 per cent nitrogen and 21 per cent oxygen. The total pressure of this mixture at sea level averages 760 mm Hg. It is clear from the preceding description of the molecular basis of pressure that each gas contributes to the total pressure in direct proportion to its concen-tration. Therefore, 79 per cent of the 760 mm Hg is caused by nitrogen (600 mm Hg) and 21 per cent by oxygen (160 mm Hg). Thus, the “partial pressure” of nitrogen in the mixture is 600 mm Hg, and the “partial pressure” of oxygen is 160 mm Hg; the total pressure is 760 mm Hg, the sum of the individual partial pressures. The partial pressures of individual gases in a mixture are designated by the symbols PO2, PCO2, PN2, PH2O, PHe, and so forth.
Pressures of Gases Dissolved in Water and Tissues
Gases dissolved in water or in body tissues also exert pressure, because the dissolved gas molecules are moving randomly and have kinetic energy. Further, when the gas dissolved in fluid encounters a surface, such as the membrane of a cell, it exerts its own partial pressure in the same way that a gas in the gas phase does. The partial pressures of the separate dissolved gases are designated the same as the partial pressures in the gas state, that is, PO2, PCO2, PN2, PHe, and so forth.
Factors That Determine the Partial Pressure of a Gas Dissolved in a Fluid. The partial pressure of a gas in a solution isdetermined not only by its concentration but also by the solubility coefficient of the gas. That is, some types ofmolecules, especially carbon dioxide, are physically or chemically attracted to water molecules, whereas others are repelled. When molecules are attracted, far more of them can be dissolved without building up excess partial pressure within the solution. Conversely, in the case of those that are repelled, high partial pressure will develop with fewer dissolved molecules. These relations are expressed by the following formula, which isHenry’s law:

When partial pressure is expressed in atmospheres (1 atmosphere pressure equals 760 mm Hg) and con-centration is expressed in volume of gas dissolved in each volume of water, the solubility coefficients for important respiratory gases at body temperature are the following:

From this table, one can see that carbon dioxide is more than 20 times as soluble as oxygen. Therefore, the partial pressure of carbon dioxide (for a given concen-tration) is less than one twentieth that exerted by oxygen.
Diffusion of Gases Between the Gas Phase in the Alveoli and the Dissolved Phase in the Pulmonary Blood. The partial pressureof each gas in the alveolar respiratory gas mixture tends to force molecules of that gas into solution in the blood of the alveolar capillaries. Conversely, the molecules of the same gas that are already dissolved in the blood are bouncing randomly in the fluid of the blood, and some of these bouncing molecules escape back into the alveoli. The rate at which they escape is directly pro-portional to their partial pressure in the blood.
But in which direction will net diffusion of the gas occur? The answer is that net diffusion is determined by the difference between the two partial pressures. If the partial pressure is greater in the gas phase in the alveoli, as is normally true for oxygen, then more molecules will diffuse into the blood than in the other direction. Alter-natively, if the partial pressure of the gas is greater in the dissolved state in the blood, which is normally true for carbon dioxide, then net diffusion will occur toward the gas phase in the alveoli.
Vapor Pressure of Water
When nonhumidified air is breathed into the respiratory passageways, water immediately evaporates from the surfaces of these passages and humidifies the air. This results from the fact that water molecules, like the dif-ferent dissolved gas molecules, are continually escaping from the water surface into the gas phase. The partial pressure that the water molecules exert to escape through the surface is called the vapor pressure of the water. At normal body temperature, 37°C, this vapor pressure is 47 mm Hg. Therefore, once the gas mixture has become fully humidified—that is, once it is in “equi-librium” with the water—the partial pressure of the water vapor in the gas mixture is 47 mm Hg. This partial pressure, like the other partial pressures, is designated PH2O.
The vapor pressure of water depends entirely on the temperature of the water. The greater the temperature, the greater the kinetic activity of the molecules and, therefore, the greater the likelihood that the water mol-ecules will escape from the surface of the water into the gas phase. For instance, the water vapor pressure at 0°C is 5 mm Hg, and at 100°C it is 760 mm Hg. But the most important value to remember is the vapor pres-sure at body temperature, 47 mm Hg; this value appears in many of our subsequent discussions.
Diffusion of Gases Through Fluids—Pressure Difference Causes Net Diffusion
Now, let us return to the problem of diffusion. From the preceding discussion, it is clear that when the partial pressure of a gas is greater in one area than in another area, there will be net diffusion from the high-pressure area toward the low-pressure area. For instance, re-turning to Figure 39–1, one can readily see that the mol-ecules in the area of high pressure, because of their greater number, have a greater statistical chance of moving randomly into the area of low pressure than do molecules attempting to go in the other direction. However, some molecules do bounce randomly from the area of low pressure toward the area of high pres-sure. Therefore, the net diffusion of gas from the area of high pressure to the area of low pressure is equal to the number of molecules bouncing in this forward direction minus the number bouncing in the opposite direction;this is proportional to the gas partial pressure difference between the two areas, called simply the pressure dif-ference for causing diffusion.
Quantifying the Net Rate of Diffusion in Fluids. In addition tothe pressure difference, several other factors affect the rate of gas diffusion in a fluid. They are (1) the solubil-ity of the gas in the fluid, (2) the cross-sectional area of the fluid, (3) the distance through which the gas must diffuse, (4) the molecular weight of the gas, and (5) the temperature of the fluid. In the body, the last of these factors, the temperature, remains reasonably constant and usually need not be considered.
The greater the solubility of the gas, the greater the number of molecules available to diffuse for any given partial pressure difference. The greater the cross-sectional area of the diffusion pathway, the greater the total number of molecules that diffuse. Conversely, the greater the distance the molecules must diffuse, the longer it will take the molecules to diffuse the entire dis-tance. Finally, the greater the velocity of kinetic move-ment of the molecules, which is inversely proportional to the square root of the molecular weight, the greater the rate of diffusion of the gas. All these factors can be expressed in a single formula, as follows:
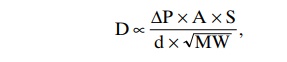
in which D is the diffusion rate, DP is the partial pres-sure difference between the two ends of the diffusion pathway, A is the cross-sectional area of the pathway, S is the solubility of the gas, d is the distance of diffusion, and MW is the molecular weight of the gas.
It is obvious from this formula that the characteristics of the gas itself determine two factors of the formula: solubility and molecular weight. Together, these two factors determine the diffusion coefficient of the gas, which is proportional to S/ MW . That is, the relative rates at which different gases at the same partial pres-sure levels will diffuse are proportional to their diffu-sion coefficients. Assuming that the diffusion coefficient for oxygen is 1, the relative diffusion coefficients for dif-ferent gases of respiratory importance in the body fluids are as follows:

Diffusion of Gases Through Tissues
The gases that are of respiratory importance are all highly soluble in lipids and, consequently, are highly soluble in cell membranes. Because of this, the major limitation to the movement of gases in tissues is the rate at which the gases can diffuse through the tissue water instead of through the cell membranes. Therefore, dif-fusion of gases through the tissues, including through the respiratory membrane, is almost equal to the diffu-sion of gases in water, as given in the preceding list.
Related Topics