Diffusion

Diffusion

All molecules and ions in the body fluids, including water molecules and dissolved substances, are in constant motion, each particle moving its own separate way. Motion of these particles is what physicists call “heat”—the greater the motion, the higher the tem-perature—and the motion never ceases under any condition except at absolute zero temperature. When a moving molecule, A, approaches a stationary mole-cule, B, the electrostatic and other nuclear forces of molecule A repel molecule B, transferring some of the energy of motion of molecule A to molecule B. Con-sequently, molecule B gains kinetic energy of motion, while molecule A slows down, losing some of its kinetic energy. Thus, as shown in Figure 4–3, a single molecule in a solution bounces among the other molecules first in one direction, then another, then another, and so forth, randomly bouncing thousands of times each second. This continual movement of mole-cules among one another in liquids or in gases is called diffusion.

Ions diffuse in the same manner as whole molecules, and even suspended colloid particles diffuse in a similar manner, except that the colloids diffuse far less rapidly than molecular substances because of their large size.

Diffusion Through the Cell Membrane

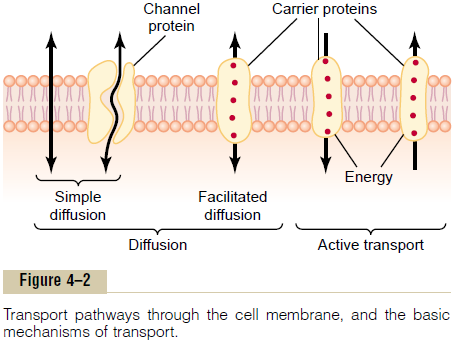
Diffusion through the cell membrane is divided into two subtypes called simple diffusion and facilitateddiffusion. Simple diffusion means that kinetic move-ment of molecules or ions occurs through a membrane opening or through intermolecular spaces without any interaction with carrier proteins in the membrane. The rate of diffusion is determined by the amount of substance available, the velocity of kinetic motion, and the number and sizes of openings in the membrane through which the molecules or ions can move.

Facilitated diffusion requires interaction of a carrier protein. The carrier protein aids passage of the mole-cules or ions through the membrane by binding chemically with them and shuttling them through the membrane in this form.

Simple diffusion can occur through the cell mem-brane by two pathways: (1) through the interstices of the lipid bilayer if the diffusing substance is lipid soluble, and (2) through watery channels that pene-trate all the way through some of the large transport proteins, as shown to the left in Figure 4–2.

Diffusion of Lipid-Soluble Substances Through the Lipid Bilayer.

One of the most important factors that determines how rapidly a substance diffuses through the lipid bilayer is the lipid solubility of the substance. For instance, the lipid solubilities of oxygen, nitrogen, carbon dioxide, and alcohols are high, so that all these can dissolve directly in the lipid bilayer and diffuse through the cell membrane in the same manner that diffusion of water solutes occurs in a watery solution. For obvious reasons, the rate of diffusion of each of these substances through the membrane is directly proportional to its lipid solubility. Especially large amounts of oxygen can be transported in this way; therefore, oxygen can be delivered to the interior of the cell almost as though the cell membrane did not exist.

Diffusion of Water and Other Lipid-Insoluble Molecules Through Protein Channels. Even though water is highly insolublein the membrane lipids, it readily passes through chan-nels in protein molecules that penetrate all the way through the membrane. The rapidity with which water molecules can move through most cell membranes is astounding. As an example, the total amount of water that diffuses in each direction through the red cell membrane during each second is about 100 times as great as the volume of the red cell itself.

Other lipid-insoluble molecules can pass through the protein pore channels in the same way as water molecules if they are water soluble and small enough. However, as they become larger, their penetration falls off rapidly. For instance, the diameter of the urea molecule is only 20 per cent greater than that of water, yet its penetration through the cell membrane pores is about 1000 times less than that of water. Even so, given the astonishing rate of water penetration, this amount of urea penetration still allows rapid transport of urea through the membrane within minutes.

Diffusion Through Protein Channels, and “Gating” of These Channels

Computerized three-dimensional reconstructions of protein channels have demonstrated tubular pathways all the way from the extracellular to the intracellu-lar fluid. Therefore, substances can move by simple diffusion directly along these channels from one side of the membrane to the other. The protein channels are distinguished by two important characteristics: (1) they are often selectively permeable to certain sub-stances, and (2) many of the channels can be opened or closed by gates.

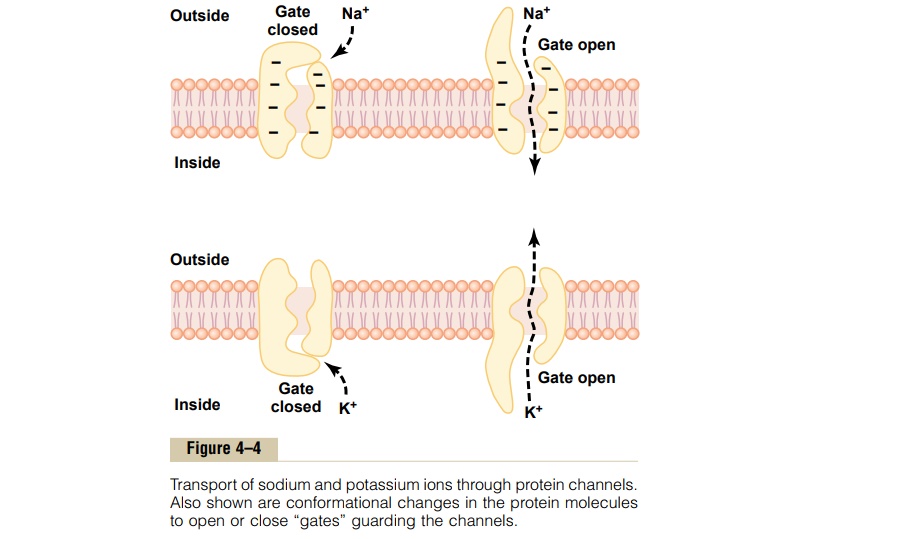
Selective Permeability of Protein Channels. Many of theprotein channels are highly selective for transport of one or more specific ions or molecules. This results from the characteristics of the channel itself, such as its diameter, its shape, and the nature of the electrical charges and chemical bonds along its inside surfaces. To give an example, one of the most important of the protein channels, the so-called sodium channel, is only 0.3 by 0.5 nanometer in diameter, but more important, the inner surfaces of this channel are strongly nega-tively charged, as shown by the negative signs insidethe channel proteins in the top panel of Figure 4–4.

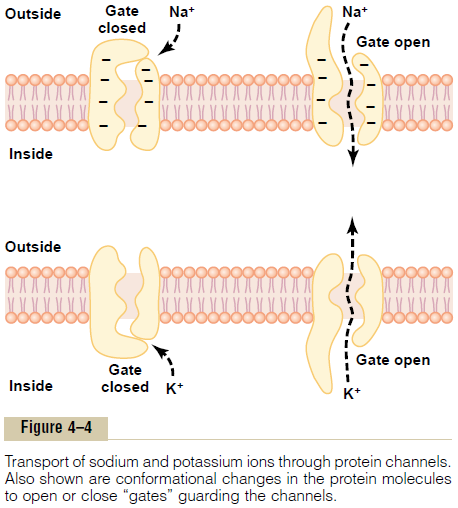
These strong negative charges can pull small dehy-drated sodium ions into these channels, actually pullingthe sodium ions away from their hydrating water molecules. Once in the channel, the sodium ions diffuse in either direction according to the usual laws of diffusion. Thus, the sodium channel is specifically selective for passage of sodium ions.

Conversely, another set of protein channels is selec-tive for potassium transport, shown in the lower panel of Figure 4–4. These channels are slightly smaller than the sodium channels, only 0.3 by 0.3 nanometer, but they are not negatively charged, and their chemicalbonds are different. Therefore, no strong attractive force is pulling ions into the channels, and the potassium ions are not pulled away from the water molecules that hydrate them. 

The hydrated form of the potassium ion is considerably smaller than the hydrated form of sodium because the sodium ion attracts far more water molecules than does potassium. Therefore, the smaller hydrated potassium ions can pass easily through this small channel, whereas the larger hydrated sodium ions are rejected, thus providing selective permeability for a specific ion.

Gating of Protein Channels. Gating of protein channelsprovides a means of controlling ion permeability of the channels. This is shown in both panels of Figure 4–4 for selective gating of sodium and potassium ions. It is believed that some of the gates are actual gatelike extensions of the transport protein molecule, which can close the opening of the channel or can be lifted away from the opening by a conformational change in the shape of the protein molecule itself.

The opening and closing of gates are controlled in two principal ways:

1.     Voltage gating. In this instance, the molecularconformation of the gate or of its chemical bonds responds to the electrical potential across the cell membrane. For instance, in the top panel of Figure 4–4, when there is a strong negative charge on the inside of the cell membrane, this presumably could cause the outside sodium gates to remain tightly closed; conversely, when the inside of the membrane loses its negative charge, these gates would open suddenly and allow tremendous quantities of sodium to pass inward through the sodium pores. This is the basic mechanism for eliciting action potentials in nerves that are responsible for nerve signals. In the bottom panel of Figure 4–4, the potassium gates are on the intracellular ends of the potassium channels, and they open when the inside of the cell membrane becomes positively charged. The opening of these gates is partly responsible for terminating the action potential.

2.     Chemical (ligand) gating. Some protein channelgates are opened by the binding of a chemical substance (a ligand) with the protein; this causes a conformational or chemical bonding change in the protein molecule that opens or closes the gate. This is called chemical gating or ligand gating. One of the most important instances of chemical gating is the effect of acetylcholine on the so-called acetylcholine channel. Acetylcholine opens the gateof this channel, providing a negatively charged pore about 0.65 nanometer in diameter that allows uncharged molecules or positive ions smaller than this diameter to pass through. This gate is exceedingly important for the transmission of nerve signals from one nerve cell to another  and from nerve cells to muscle cells to cause muscle contraction.

Open-State  Versus  Closed-State  of  Gated  Channels.

Figure 4–5A shows an especially interesting charac-teristic of most voltage-gated channels. This figure shows two recordings of electrical current flowing through a single sodium channel when there was an approximate 25-millivolt potential gradient across the

membrane. Note that the channel conducts current either “all or none.” That is, the gate of the channel snaps open and then snaps closed, each open state lasting for only a fraction of a millisecond up to several milliseconds. This demonstrates the rapidity with which changes can occur during the opening and closing of the protein molecular gates. At one voltage potential, the channel may remain closed all the time or almost all the time, whereas at another voltage level, it may remain open either all or most of the time. At in-between voltages, as shown in the figure, the gates tend to snap open and closed intermittently, giving an average current flow somewhere between the minimum and the maximum.

Patch-Clamp Method for Recording Ion Current Flow Through Single Channels.

One might wonder how it is technicallypossible to record ion current flow through single protein channels as shown in Figure 4–5A. This has been achieved by using the “patch-clamp” method illustrated in Figure 4–5B. Very simply, a micropipette, having a tip diameter of only 1 or 2 micrometers, is abutted against the outside of a cell membrane. Then suction is applied inside the pipette to pull the membrane against the tip of the pipette. This creates a seal where the edges of the pipette touch the cell membrane. The result is a minute membrane “patch” at the tip of the pipette through which electrical current flow can be recorded.

Alternatively, as shown to the right in Figure 4–5B, the small cell membrane patch at the end of the pipette can be torn away from the cell. The pipette with its sealed patch is then inserted into a free solution. This allows the concentrations of ions both inside the micropipette and in the outside solution to be altered as desired. Also, the voltage between the two sides of the membrane can be set at will—that is, “clamped” to a given voltage.

It has been possible to make such patches small enough so that only a single channel protein is found in the membrane patch being studied. By varying the concentrations of different ions, as well as the voltage across the membrane, one can determine the transport characteristics of the single channel and also its gating properties.

Facilitated Diffusion

Facilitated diffusion is also called carrier-mediated dif-fusion because a substance transported in this mannerdiffuses through the membrane using a specific carrier protein to help. That is, the carrier facilitates diffusion of the substance to the other side.

Facilitated diffusion differs from simple diffusion in the following important way: Although the rate of simple diffusion through an open channel increases proportionately with the concentration of the diffus-ing substance, in facilitated diffusion the rate of diffusion approaches a maximum, called Vmax, as the concentration of the diffusing substance increases.This difference between simple diffusion and facilitated dif-fusion is demonstrated in Figure 4–6. The figure shows that as the concentration of the diffusing substance increases, the rate of simple diffusion continues to increase proportionately, but in the case of facilitated diffusion, the rate of diffusion cannot rise greater than the Vmax level.

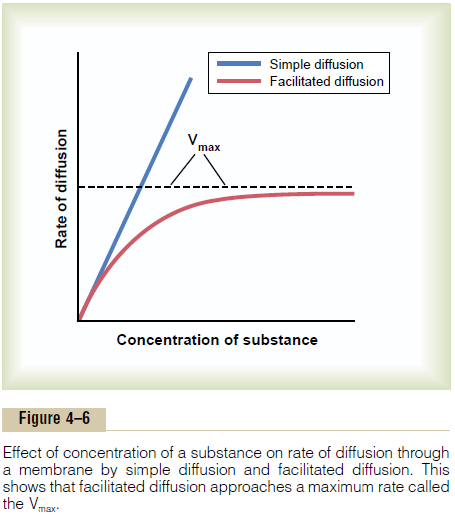
What is it that limits the rate of facilitated diffusion?

A probable answer is the mechanism illustrated in Figure 4–7. This figure shows a carrier protein with a pore large enough to transport a specific molecule partway through. It also shows a binding “receptor” on the inside of the protein carrier. The molecule to be transported enters the pore and becomes bound. Then, in a fraction of a second, a conformational or chemi-cal change occurs in the carrier protein, so that the pore now opens to the opposite side of the membrane. Because the binding force of the receptor is weak, the thermal motion of the attached molecule causes it to break away and to be released on the opposite side of the membrane. The rate at which molecules can be transported by this mechanism can never be greater than the rate at which the carrier protein molecule can undergo change back and forth between its two states. Note specifically, though, that this mechanism allows the transported molecule to move—that is, to “diffuse”—in either direction through the membrane.

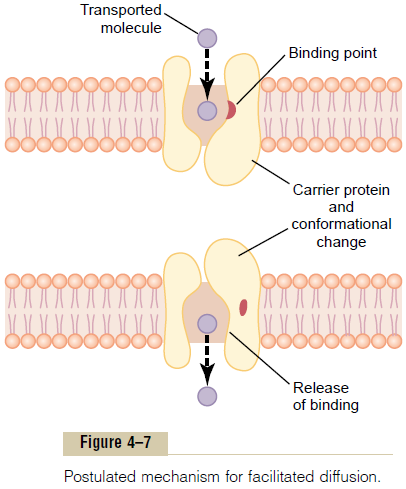
Among the most important substances that cross cell membranes by facilitated diffusion are glucose and most of the amino acids. In the case of glucose, the carrier molecule has been discovered, and it has a molecular weight of about 45,000; it can also transport several other monosaccharides that have structures similar to that of glucose, including galactose. Also, insulin can increase the rate of facilitated diffusion of glucose as much as 10-fold to 20-fold. This is the prin-cipal mechanism by which insulin controls glucose use in the body.

Factors That Affect Net Rate of Diffusion

By now it is evident that many substances can diffuse through the cell membrane. What is usually important is the net rate of diffusion of a substance in the desired direction. This net rate is determined by several factors.

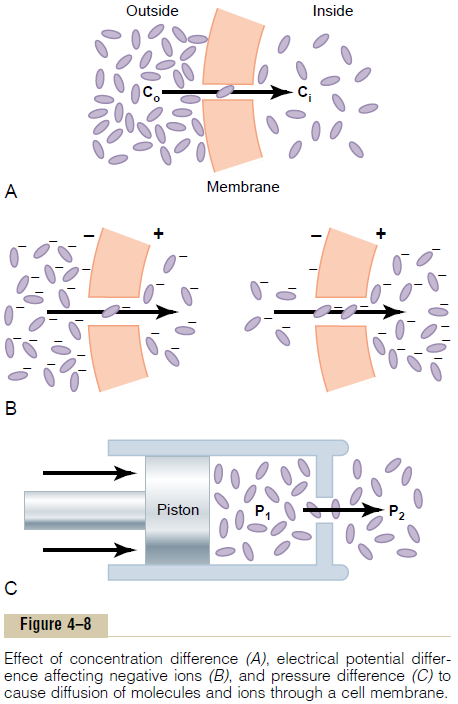
Effect of Concentration Difference on Net Diffusion Through a Membrane. Figure 4–8Ashows a cell membrane with asubstance in high concentration on the outside and low concentration on the inside. The rate at which the sub-stance diffuses inward is proportional to the con-centration of molecules on theoutside, because this concentration determines how many molecules strike the outside of the membrane each second. Conversely, the rate at which molecules diffuse outward is propor-tional to their concentration inside the membrane. Therefore, the rate of net diffusion into the cell is pro-portional to the concentration on the outside minus the concentration on the inside, or:

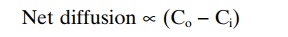
in which Co is concentration outside and Ci is concen-tration inside.

Effect of Membrane Electrical Potential on Diffusion of Ions— The “Nernst Potential.” If an electrical potential isapplied across the membrane, as shown in Figure 4–8B, the electrical charges of the ions cause them to move through the membrane even though no concen-tration difference exists to cause movement. Thus, in the left panel of Figure 4–8B, the concentration of negative ions is the same on both sides of the mem-brane, but a positive charge has been applied to the right side of the membrane and a negative charge to the left, creating an electrical gradient across the mem-brane. The positive charge attracts the negative ions, whereas the negative charge repels them. 

Therefore, net diffusion occurs from left to right.After much time, large quantities of negative ions have moved to the right, creating the condition shown in the right panel of Figure 4–8B, in which a concentration difference of the ions has developed in the direction opposite to the electrical potential difference. The concentration dif-ference now tends to move the ions to the left, while the electrical difference tends to move them to the right. When the concentration difference rises high enough, the two effects balance each other. At normal body temperature (37°C), the electrical difference that will balance a given concentration difference of univalent ions—such as sodium (Na+) ions—can bedetermined from the following formula, called the

Nernst equation:

EMF (in millivolts) = ± 61 log C1/ C2

in which EMF is the electromotive force (voltage) between side 1 and side 2 of the membrane, C1 is the concentration on side 1, and C2 is the concentration on side 2. This equation is extremely important in under-standing the transmission of nerve impulses.

Effect of a Pressure Difference Across the Membrane. Attimes, considerable pressure difference develops between the two sides of a diffusible membrane. This occurs, for instance, at the blood capillary mem-brane in all tissues of the body. The pressure is about 20 mm Hg greater inside the capillary than outside.

Pressure actually means the sum of all the forces of the different molecules striking a unit surface area at a given instant. Therefore, when the pressure is higher on one side of a membrane than on the other, this means that the sum of all the forces of the molecules striking the channels on that side of the membrane is greater than on the other side. In most instances, this is caused by greater numbers of molecules striking the membrane per second on one side than on the other side. The result is that increased amounts of energy are available to cause net movement of molecules from the high-pressure side toward the low-pressure side. This effect is demonstrated in Figure 4–8C, which shows a piston developing high pressure on one side of a “pore,” thereby causing more molecules to strike the pore on this side and, therefore, more molecules to “diffuse” to the other side.

Osmosis Across Selectively Permeable Membranes— “Net Diffusion” of Water

By far the most abundant substance that diffuses through the cell membrane is water. Enough water ordinarily diffuses in each direction through the red cell membrane per second to equal about 100 times thevolume of the cell itself. Yet, normally, the amount thatdiffuses in the two directions is balanced so precisely that zero net movement of water occurs. Therefore, the volume of the cell remains constant. However, under certain conditions, aconcentration difference for water can develop across a membrane, just as concentration differences for other substances can occur. When this happens, net movement of water does occur across the cell membrane, causing the cell either to swell or to shrink, depending on the direction of the water move-ment. This process of net movement of water caused by a concentration difference of water is called osmosis.

To give an example of osmosis, let us assume the conditions shown in Figure 4–9, with pure water on one side of the cell membrane and a solution of sodium chloride on the other side. Water molecules pass through the cell membrane with ease, whereas sodium and chloride ions pass through only with diffi-culty. Therefore, sodium chloride solution is actually a mixture of permeant water molecules and nonperme-ant sodium and chloride ions, and the membrane is said to be selectively permeable to water but much less so to sodium and chloride ions. Yet the presence of the sodium and chloride has displaced some of the water molecules on the side of the membrane where these ions are present and, therefore, has reduced the con-centration of water molecules to less than that of pure water. As a result, in the example of Figure 4–9, more water molecules strike the channels on the left side,

where there is pure water, than on the right side, where the water concentration has been reduced. Thus, net movement of water occurs from left to right—that is, osmosis occurs from the pure water into the sodiumchloride solution.

Osmotic Pressure

If in Figure 4–9 pressure were applied to the sodium chloride solution, osmosis of water into this solution would be slowed, stopped, or even reversed. The exact amount of pressure required to stop osmosis is called the osmotic pressure of the sodium chloride solution.

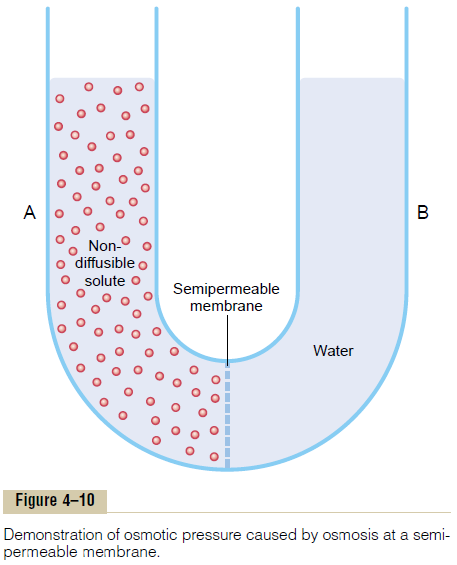
The principle of a pressure difference opposing osmosis is demonstrated in Figure 4–10, which shows a selectively permeable membrane separating two columns of fluid, one containing pure water and the other containing a solution of water and any solute that will not penetrate the membrane. Osmosis of water from chamber B into chamber A causes the levels of the fluid columns to become farther and farther apart, until eventually a pressure difference develops between the two sides of the membrane great enough to oppose the osmotic effect. The pressure dif-ference across the membrane at this point is equal to the osmotic pressure of the solution that contains the nondiffusible solute.

Importance of Number of Osmotic Particles (Molar Concentra-tion) in Determining Osmotic Pressure. The osmotic pres-sure exerted by particles in a solution, whether they are molecules or ions, is determined by the number of particles per unit volume of fluid, not by the mass of the particles. The reason for this is that each particle in a solution, regardless of its mass, exerts, on average, the same amount of pressure against the membrane. That is, large particles, which have greater mass (m) than small particles, move at slower velocities (v). The small particles move at higher velocities in such a way that their average kinetic energies (k), determined by the equation

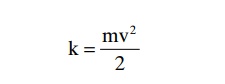
are the same for each small particle as for each large particle. Consequently, the factor that determines the osmotic pressure of a solution is the concentration of the solution in terms of number of particles (which is the same as its molar concentration if it is a nondisso-ciated molecule), not in terms of mass of the solute.

“Osmolality”—The Osmole. To express the concentrationof a solution in terms of numbers of particles, the unit called the osmole is used in place of grams.

One osmole is 1 gram molecular weight of osmoti-cally active solute. Thus, 180 grams of glucose, which is 1 gram molecular weight of glucose, is equal to 1 osmole of glucose because glucose does not dissociate into ions. Conversely, if a solute dissociates into two ions, 1 gram molecular weight of the solute will become 2 osmoles because the number of osmotically active particles is now twice as great as is the case for the nondissociated solute. Therefore, when fully disso-ciated, 1 gram molecular weight of sodium chloride, 58.5 grams, is equal to 2 osmoles.

Thus, a solution that has 1 osmole of solute dissolvedin each kilogram of water is said to have an osmolality of 1 osmole per kilogram, and a solution that has1/1000 osmole dissolved per kilogram has an osmo-lality of 1 milliosmole per kilogram. The normal osmolality of the extracellular and intracellular fluids is about 300 milliosmoles per kilogram of water.

Relation of Osmolality to Osmotic Pressure. At normal bodytemperature, 37°C, a concentration of 1 osmole per liter will cause 19,300 mm Hg osmotic pressure in the solution. Likewise, 1 milliosmole per liter concentra-tion is equivalent to 19.3 mm Hg osmotic pressure. Multiplying this value by the 300 milliosmolar con-centration of the body fluids gives a total calculated osmotic pressure of the body fluids of 5790 mm Hg. The measured value for this, however, averages only about 5500 mm Hg. The reason for this difference is that many of the ions in the body fluids, such as sodium and chloride ions, are highly attracted to one another; consequently, they cannot move entirely unrestrained in the fluids and create their full osmotic pressure potential. Therefore, on average, the actual osmotic pressure of the body fluids is about 0.93 times the cal-culated value.

       The Term “Osmolarity.” Because of the difficulty of meas-uring kilograms of water in a solution, which is required to determine osmolality,osmolarity, which is the osmolar concentration expressed as osmoles per liter ofsolution rather than osmoles per kilogram of water, is used instead. Although, strictly speaking, it is osmoles per kilogram of water (osmolality) that determines osmotic pressure, for dilute solutions such as those in the body, the quantitative differences between osmo-larity and osmolality are less than 1 per cent. Because it is far more practical to measure osmolarity than osmo-lality, this is the usual practice in almost all physiologic studies.