Formula, Solved Example Problems | Statistics | Mathematics - Calculation of Standard Deviation | 10th Mathematics : UNIT 8 : Statistics And Probability
Chapter: 10th Mathematics : UNIT 8 : Statistics And Probability
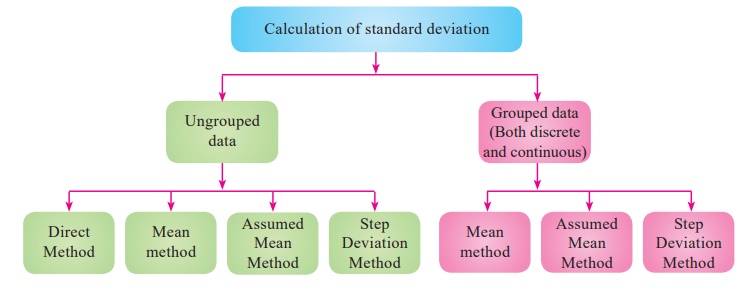
Calculation of Standard Deviation
1. Calculation of Standard Deviation for ungrouped data
(i) Direct Method
(ii) Mean method
(iii) Assumed Mean method
(iv) Step deviation method
2. Calculation of Standard deviation for continuous frequency distribution
(i) Mean method
(ii) Assumed Mean method
(iii) Step deviation method
1. Calculation of Standard Deviation for ungrouped data
(i) Direct Method
Note
· While computing standard deviation, arranging data in ascending order is not mandatory.
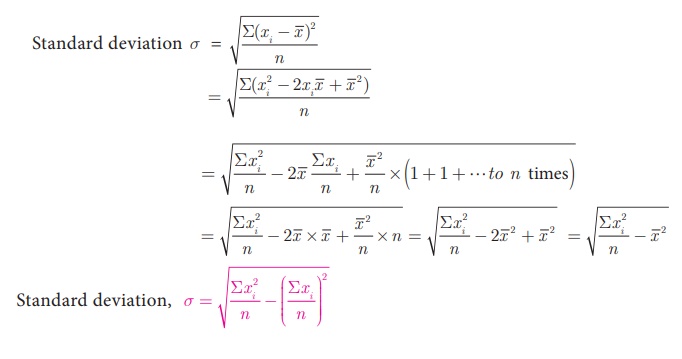
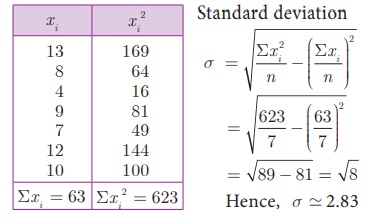
· If the data values are given directly then to find standard deviation we can use the formula σ = 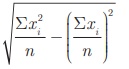
· If the data values are not given directly but the squares of the deviations from the mean of each observation is given then to find standard deviation we can use the formula σ = 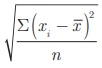
Example 8.4 The number of televisions sold in each day of a week are 13, 8, 4, 9, 7, 12, 10.
Find its standard deviation.
Solution
(ii) Mean method
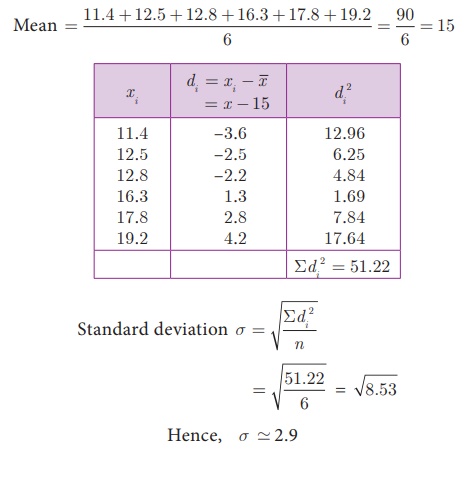
Another convenient way of finding standard deviation is to use the following formula.
Standard deviation (by mean method) σ = 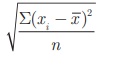
If di = xi – ![]() are the deviations, then
are the deviations, then 
Example 8.5 The amount of rainfall in a particular season for 6 days are given as 17.8 cm, 19.2 cm, 16.3 cm, 12.5 cm, 12.8 cm and 11.4 cm. Find its standard deviation.
Solution Arranging the numbers in ascending order we get, 11.4, 12.5, 12.8, 16.3, 17.8, 19.2.
Number of observations n = 6
(iii) Assumed Mean method
When the mean value is not an integer (since calculations are very tedious in decimal form) then it is better to use the assumed mean method to find the standard deviation.
Let x 1 , x2, x 3 , ..., xn be the given data values and let ![]() be their mean.
be their mean.
Let di be the deviation of xi from the assumed mean A, which is usually the middle value or near the middle value of the given data.
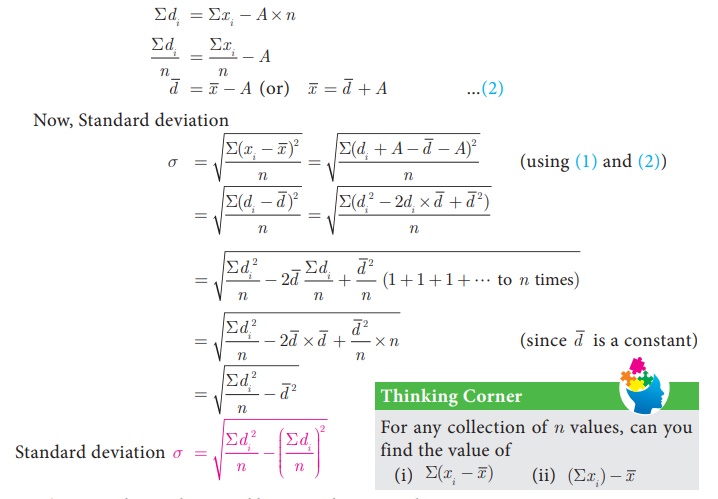
di= xi − A gives, xi = di + A ...(1)
Σdi= Σ(xi −A)
= Σxi −(A + A + A + . . . to n times)
Σdi = Σxi – A × n
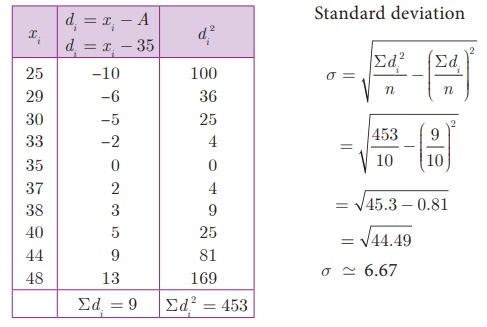
Example 8.6 The marks scored by 10 students in a class test are 25, 29, 30, 33, 35, 37, 38, 40, 44, 48. Find the standard deviation.
Solution The mean of marks is 35.9 which is not an integer. Hence we take assumed mean, A = 35, n = 10 .
(iv) Step deviation method
Let x 1 , x2, x 3 ,...xn be the given data. Let A be the assumed mean.
Let c be the common divisor of x i - A .
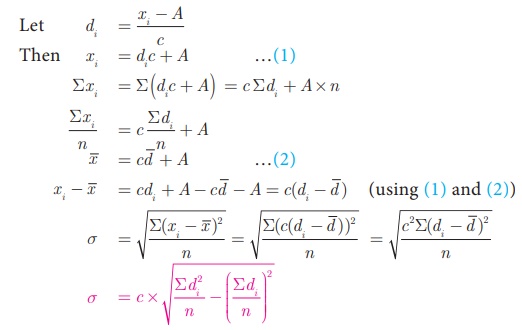
Note
We can use any of the above methods for finding the standard deviation
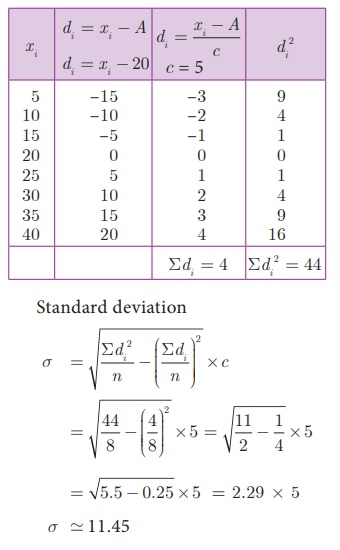
Example 8.7 The amount that the children have spent for purchasing some eatables in one day trip of a school are 5, 10, 15, 20, 25, 30, 35, 40. Using step deviation method, find the standard deviation of the amount they have spent.
Solution We note that all the observations are divisible by 5. Hence we can use the step deviation method. Let the Assumed mean A = 20, n = 8.
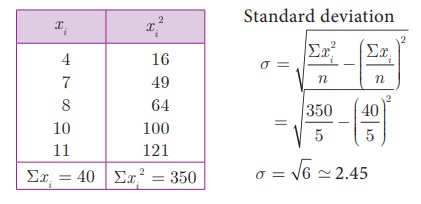
Example 8.8 Find the standard deviation of the following data 7, 4, 8, 10, 11. Add 3 to all the values then find the standard deviation for the new values.
Solution Arranging the values in ascending order we get, 4, 7, 8, 10, 11 and n = 5
When we add 3 to all the values, we get the new values as 7,10,11,13,14.
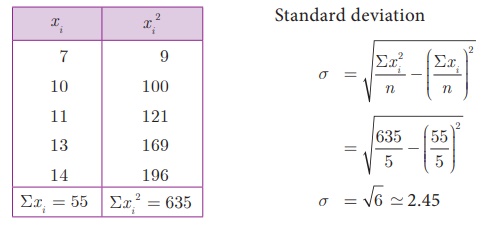
From the above, we see that the standard deviation will not change when we add some fixed constant to all the values.
Example 8.9 Find the standard deviation of the data 2, 3, 5, 7, 8. Multiply each data by 4. Find the standard deviation of the new values.
Solution Given, n = 5
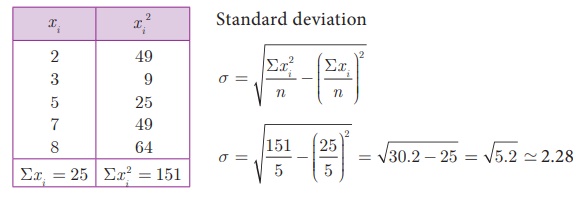
When we multiply each data by 4, we get the new values as 8, 12, 20, 28, 32.

From the above, we see that when we multiply each data by 4 the standard deviation also get multiplied by 4.
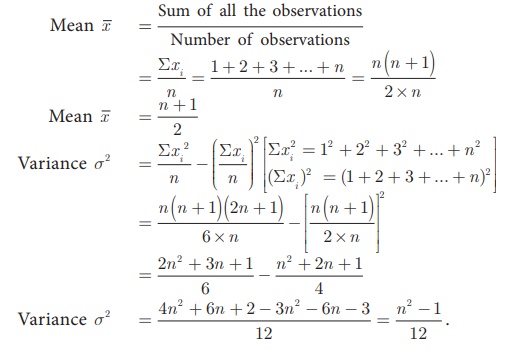
Example 8.10 Find the mean and variance of the first n natural numbers.
Solution
Calculation of Standard deviation for grouped data
(i) Mean method
Example 8.11
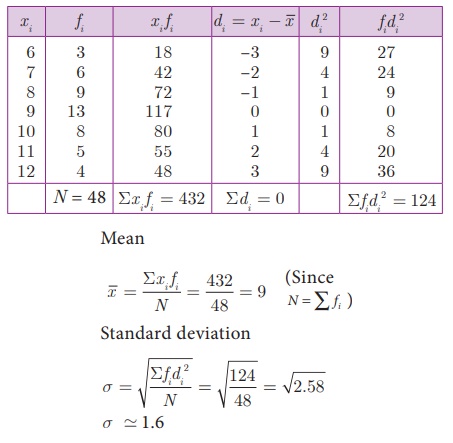
48 students were asked to write the total number of hours per week they spent on watching television. With this information find the standard deviation of hours spent for watching television.

Solution
(ii) Assumed Mean Medthod
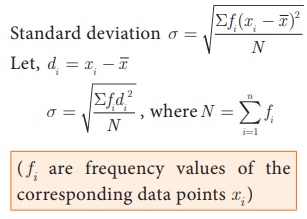
Let x 1 , x2, x 3 , ...xn be the given data with frequencies f1 , f2, f3 , ... fn respectively. Let x be their mean and A be the assumed mean..
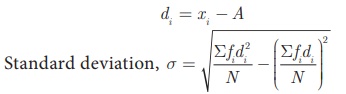
Example 8.12
The marks scored by the students in a slip test are given below.
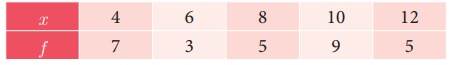
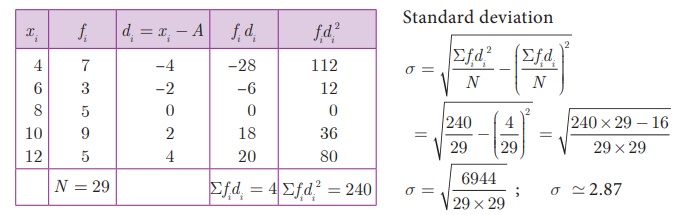
Find the standard deviation of their marks.
Solution
Let the assumed mean, A = 8
2. Calculation of Standard deviation for continuous frequency distribution
(i) Mean method
Standard deviation 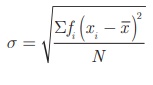 where xi = Middle value of the i th class.
where xi = Middle value of the i th class.
fi = Frequency of the i th class.
(ii) Shortcut method (or) Step deviation method
To make the calculation simple, we provide the following formula. Let A be the assumed mean, xi be the middle value of the ith class and c is the width of the class interval.
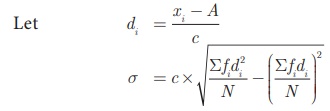
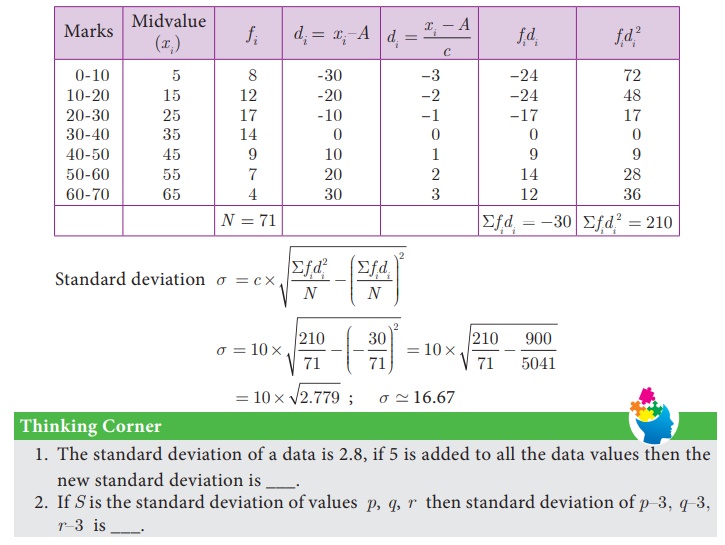
Example 8.13
Marks of the students in a particular subject of a class are given below.

Find its standard deviation.
Solution
Let the assumed mean, A = 35, c = 10
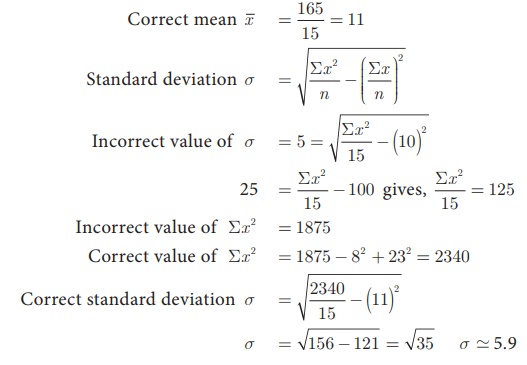
Example 8.14
The mean and standard deviation of 15 observations are found to be 10 and 5 respectively. On rechecking it was found that one of the observation with value 8 was incorrect. Calculate the correct mean and standard deviation if the correct observation value was 23?
Solution

Wrong observation value = 8, Correct observation value = 23.
Correct total = 150 − 8 + 23 = 165
Related Topics