Absolute Measures

Absolute Measures

It involves the units of measurements of the observations. For example, (i) the dispersion of salary of employees is expressed in rupees, and (ii) the variation of time required for workers is expressed in hours. Such measures are not suitable for comparing the variability of the two data sets which are expressed in different units of measurements.

1. Range

Raw Data:

Range is defined as difference between the largest and smallest observations in the data set. Range(R) = Largest value in the data set (L) –Smallest value in the data set(S)

R= L - S

Grouped Data:

For grouped frequency distribution of values in the data set, the range is the difference between the upper class limit of the last class interval and the loawer class limit of first class interval.

Coefficient of Range

The relative measure of range is called the coefficient of range.

Co efficient of Range = (L-S) / (L+S)

Example 6.2

The following data relates to the heights of 10 students (in cms) in a school.

Calculate the range and coefficient of range.

158, 164, 168, 170, 142, 160, 154, 174, 159, 146

Solution:

L=142 S=174

Range = L – S = 174 – 142 = 32

Coefficient of range = (L-S)( L+S)

=(174-142)/(174+142) = 32/316 = 0.101

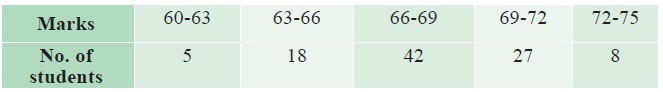
Example 6.3

Calculate the range and the co-efficient of range for the marks obtained by 100 students in a school.

Solution:

L = Upper limit of highest class  = 75

S = lower limit of lowest class = 60

Range  = L-S = 75-60 = 15

Coefficient of range = (L-S)( L+S)

=15/(75+60) = 0.111

Merits:

·           Range is the simplest measure of dispersion.

·           It is well defined, and easy to compute.

·           It is widely used in quality control, weather forecasting, stock market variations etc.

Limitations:

·           The calculations of range is based on only two values – largest value and smallest value.

·           It is largely influenced by two extreme value.

·           It cannot be computed in the case of open-ended frequency distributions.

·           It is not suitable for further mathematical treatment.

2. Inter Quartile Range and Quartile Deviation

The quartiles Q₁, Q₂ and Q₃ have been introduced and studied in Chapter 5.

Inter quartile range is defined as: Inter quartile Range (IQR) = Q₃-Q₁

Quartile Deviation is defined as, half of the distance between Q₁ and Q₃.

Quartile Deviation Q.D = Q₃-Q₁ / 2

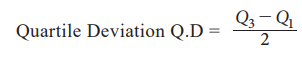
It is also called as semi-inter quartile range.

Coefficient of Quartile Deviation

The relative measure corresponding to QD is coefficient of QD and is defined as:

Coefficient of Quartile Deviation = Q₃-Q₁ / Q₃+Q₁

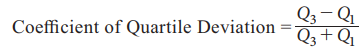
Merits:

·           It is not affected by the extreme (highest and lowest) values in the data set.

·           It is an appropriate measure of variation for a data set summarized in open-ended class intervals.

·           It is a positional measure of variation; therefore it is useful in the cases of erratic or highly skewed distributions.

Limitations:

·           The QD is based on the middle 50 per cent observed values only and is not based on all the observations in the data set, therefore it cannot be considered as a good measure of variation.

·           It is not suitable for mathematical treatment.

·           It is affected by sampling fluctuations.

·           The QD is a positional measure and has no relationship with any average in the data set.

3. Mean Deviation

The Mean Deviation (MD) is defined as the arithmetic mean of the absolute deviations of the individual values from a measure of central tendency of the data set. It is also known as the average deviation.

The measure of central tendency is either mean or median. If the measure of central tendency is mean (or median), then we get the mean deviation about the mean (or median).

The coefficient of mean deviation (CMD) is the relative measure of dispersion corresponding to mean deviation and it is given by

Example 6.4

The following are the weights of 10 children admitted in a hospital on a particular day.

Find the mean deviation about mean, median and their coefficients of mean deviation.

7,4,10,9,15,12,7,9,9,18

Solution:

4. Standard Deviation

Consider the following data sets.

It is obvious that the range for the three sets of data is 8. But a careful look at these sets clearly shows the numbers are different and there is a necessity for a new measure  to address the real variations among the numbers in the three data sets. This variation is measured by standard deviation. The idea of standard deviation was given by Karl Pearson in 1893.

Definition

 ‘Standard deviation is the positive square root of average of the deviations of all the observation taken from the mean.’ It is denoted by a greek letter v.

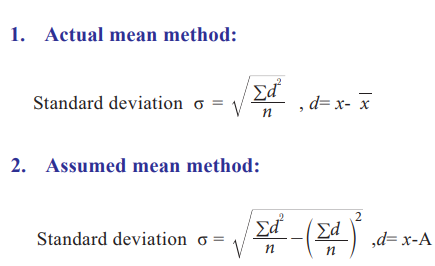
a. Ungrouped data

x₁ , x₂ , x₃  ... x_n are the ungrouped data then standard deviation is calculated by

b. Grouped Data (Discrete)

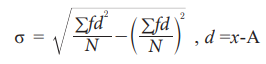
Where, f = frequency of each class interval

N = total number of observation (or elements) in the population

x = mid – value of each class interval

where A is an assumed A.M.

c. Grouped Data (continuous)

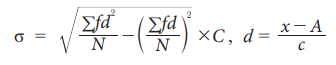
Where, f = frequency of each class interval

N = total number of observation (or elements) in the population

c = width of class interval

x = mid-value of each class interval where A is an assumed A.M.

Variance : Sum of the squares of the deviation from mean is known as Variance.

The square root of the variance is known as standard deviation. 

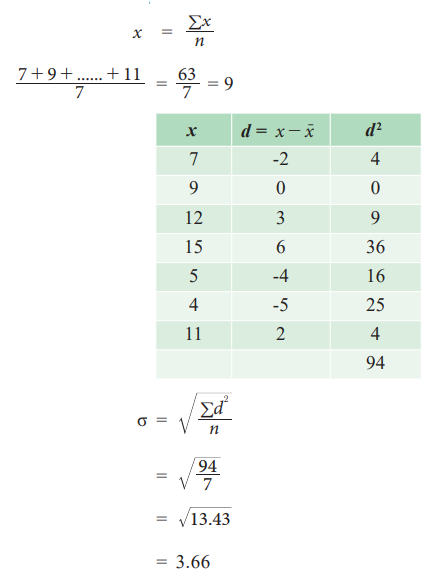
Example 6.5

The following data gives the number of books taken in a school library in 7 days find the standard deviation of the book taken

7, 9, 12, 15, 5, 4, 11

Solution:

Actual mean method

Merits:

·           The value of standard deviation is based on every observation in a set of data.

·           It is less affected by fluctuations of sampling.

·           It is the only measure of variation capable of algebraic treatment.

Limitations:

·           Compared to other measures of dispersion, calculations of standard deviation are difficult.

·           While calculating standard deviation, more weight is given to extreme values and less to those near mean.

·           It cannot be calculated in open intervals.

·           If two or more data set were given in different units, variation among those data set cannot be compared.

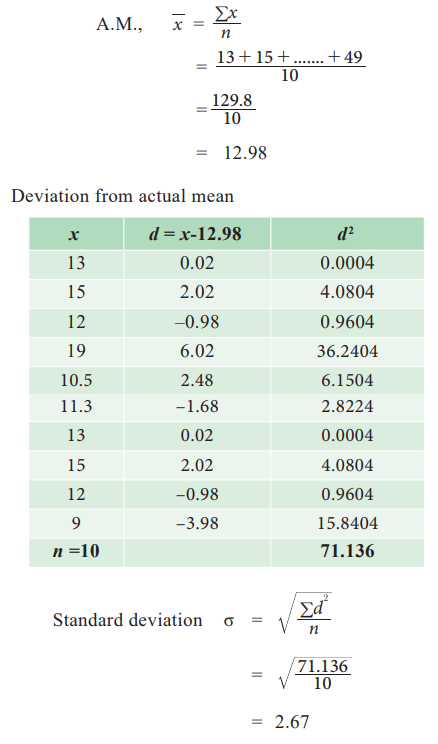
Example 6.6

Raw Data:

Weights of children admitted in a hospital is given below calculate the standard deviation of weights of children.

13, 15, 12, 19, 10.5, 11.3, 13, 15, 12, 9

Solution:

Example 6.7

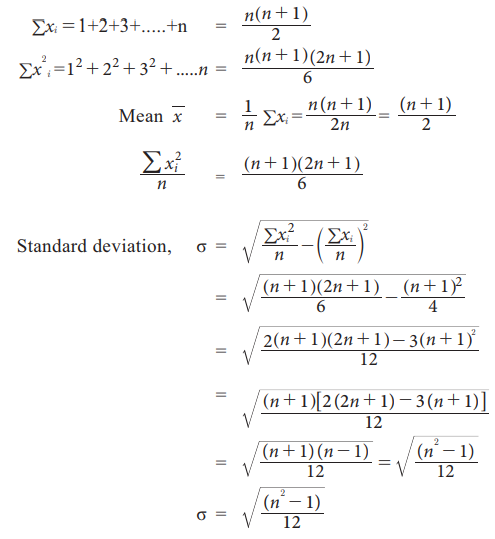
Find the standard deviation of the first ‘n’ natural numbers.

Solution:

The first n natural numbers are 1, 2, 3,…, n. The sum and the sum of squares of these n numbers are

Example 6.8

The wholesale price of a commodity for seven consecutive days in a month is as follows:

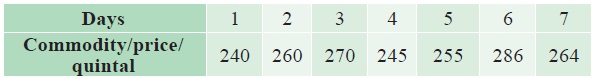
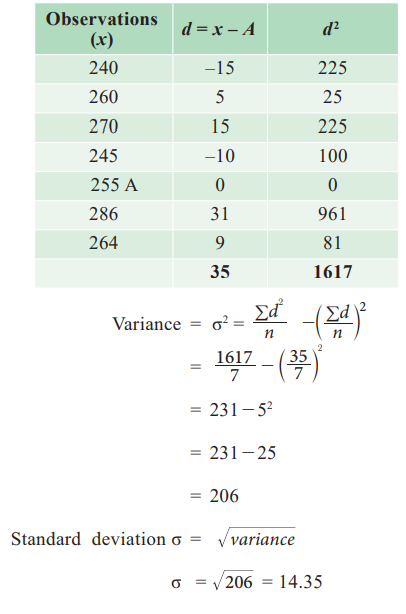
Calculate the variance and standard deviation.

Solution:

The computations for variance and standard deviation is cumbersome when x values are large. So, another method is used, which will reduce the calculation time. Here we take the deviations from an assumed mean or arbitrary value A such that d = x – A

In this question, if we take deviation from an assumed A.M. =255. The calculations then for standard deviation will be as shown in below Table;

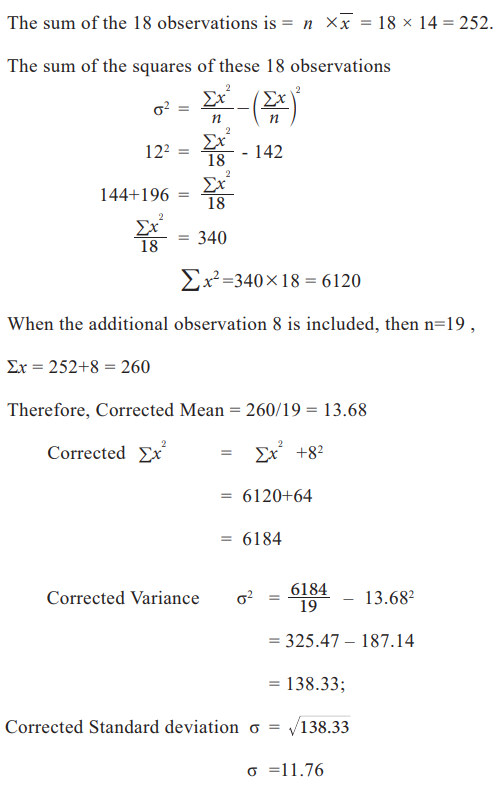
Example 6.9

The mean and standard deviation from 18 observations are 14 and 12 respectively. If an additional observation 8 is to be included, find the corrected mean and standard deviation.

Solution:

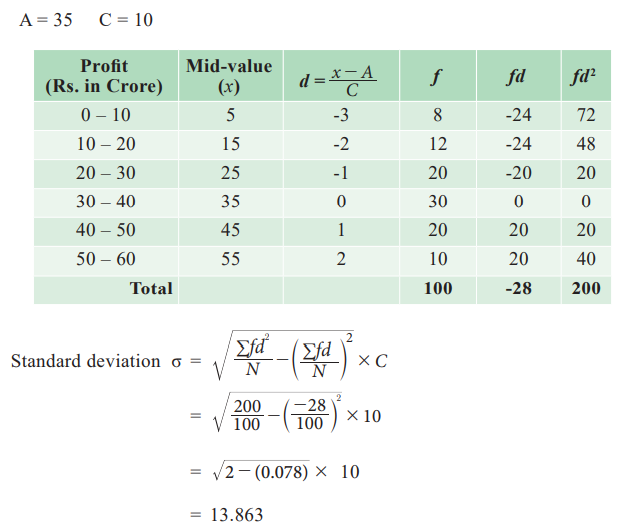
Example 6.10

A study of 100 engineering companies gives the following information

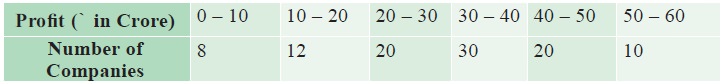
Calculate the standard of the profit earned.

Solution: