Vernier Motors

VERNIER MOTORS

A Vernier motor is an unexcited (or reluctance Type) inductor synchronous motor. It is also named because it operates on the principle of a vernier. The peculiar feature of this kind of motor is that a small displacement of the rotor produces a large displacement of the axes of maximum and minimum permeance. When a rotating magnetic field is introduced in the air gap of the machine, rotor will rotate slowly and at a definite fraction of the speed of the rotating field.

This rotating field can be produced either by feeding poly phase current to the stator winding or by exciting the stator coil groups in sequence. AS the rotor speed steps down from the speed of the rotating field, the motor torque steps up. A vernier motor works as an electric gearing. This kind of motor is attractive in applications which require low speed and high torque and where mechanical gearing is undesirable.

1. Principle of operation

The stator of a vernier motor has slots and a distributed winding just like the stator of an ordinary poly phase induction motor. The rotor is a slotted iron core without winding. A 2 – pole machine with 12 stator slots and 10 rotor slots.

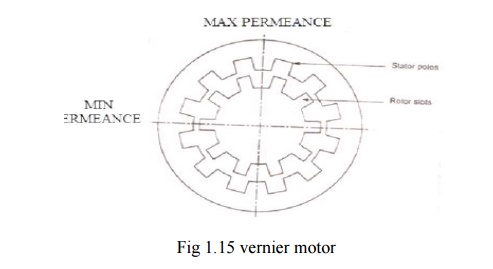
The stator and rotor teeth are facing each other in the vertical axis. The stator teeth are facing rotor slots in the horizontal axis. At this position therefore, the maximum permeance is along the vertical axis and the minimum permeance is along the horizontal axis. When then rotor is rotated one half of its slot pitch, the rotor slots will face stator teeth in the vertical axis. The rotor and stator teeth will face each other in the horizontal axis. The axis of maximum permeance is now horizontal and the axis of minimum permeance is now vertical. Thus the rotor movement of one –half rotor slot pitch results in a 90 degree displacement of the permeance axes.

Suppose that a magnetic field is rotating in the machine. Whenever the rotating field rotates 90 degrees, the rotor will rotate one half of its slot pitch. When the rotating field completes one revolution, the rotor will rotate through an angle corresponding to two rotor slot pitches.

2. Air – Gap permeance Distribution

The fluxes in the air gap are assumed all in the radial direction. The permeance of air space between stator and rotor at any location is inversely proportional to the radial length of air space at that location. The stator and rotor slot depth are much larger in comparison with air gap length, the permeance of airspace can be considered as zero, where stator tooth surface is facing rotor tooth surface. The width of rectangular blocks is the widths of overlap between the stator and the rotor teeth. These widths of overlap vary linearly from a maximum and back to a minimum. The area of overlap is reduced a constant amount for each successive stator tooth until a minimum is reached.

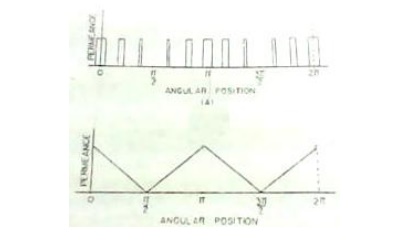
The permeance distribution curve is not convenient to use because it cannot be represented by simple mathematical function. When the rotor rotates, this permanence wave rotates at a much faster speed. Five times the rotor speed for the machine. The axes at which maximum and minimum permeance occur are tge direct and quadrature axes respectively of the vernier motor.

3. Design of Vernier Motor

In a poly phase reluctance motor the rotor has the same number of poles as the stator mmfwave.Similarly in a vernier motor the air gap permeance wave should have the same number of poles as the stator mmf wave. The number of stator and rotor slots has the following relation

N1 = N2 ± P

Where

N1 – Number of Stator Slots

N2 – Number of Rotor Slots

P – Number of poles of the rotating magnetic field.

When the rotor rotates through an angle corresponding to one rotor slot pitch, the permeance wave rotates through an angle corresponding to one pole pitch. The pole pitch of the permeance wave is the same as the pole pitch of the stator mmf wave, because they have the same number of poles. Also in a reluctance machine, the speed of the permeance wave is the speed of rotating mmf.

Therefore,

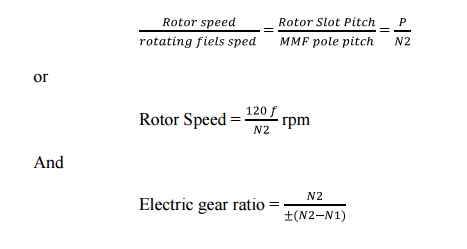
The rotor speed is independent of the number of poles of the machine when the speed of rotating magnetic field is reduced by increasing the number of poles of the machine. It cannot be expected that the speed of the rotor be reduced proportionately because when P is increased the difference between N2 and N1 should also be increased, and the electric gear ratio is reduced in the inverse proportion. Thus the rotor speed is not affected by the number of poles but depends on the number of rotor slots.

The main step in design is to calculate the direct and quadrature axes reactance‘s X_d

and X_q.

Xd = X1 +Xad

Xq = X1 + Xaq

Where X1 is the stator leakage reactance and Xad and Xaq are the direct and quadrature axes reactance of armature reaction.Xad is the ratio of the fundamental component of reactive armature voltage, produced by the mutual flux due to the fundamental direct axis component of armature current,Similarly Xaq is the ratio of the fundamental component of reactive armature voltage produced by the mutual flux due to the fundamental quadrature axis component of the armature current, to its component under steady state conditions and at rated frequency.