Chapter: Mechanical : Engineering Economics & Cost Analysis : Replacement And Maintenance Analysis
Types of Replacement Problem
Types of Replacement Problem
Replacement study can be classified into two categories:
(a) Replacement of assets that deteriorate with time (Replacement due to gradual failure, or wear and tear of the components of the machines).
This can be further classified into the following types:
(i) Determination of economic life of an asset.
(ii) Replacement of an existing asset with a new asset.
(b) Simple probabilistic model for assets which fail completely (replacement due to sudden failure).
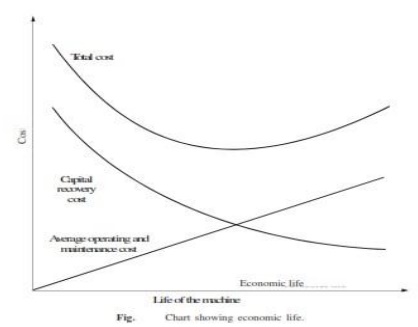
Determination of Economic Life of an Asset
Any asset will have the following cost components:
ü Capital recovery cost (average first cost), computed from the first cost (purchase price) of the machine.
ü Average operating and maintenance cost (O & M cost)
Total cost which is the sum of capital recovery cost (average first cost) and average maintenance cost.
EXAMPLE
A firm is considering replacement of an equipment, whose first cost is Rs. 4,000 and the scrap value is negligible at the end of any year. Based on experience, it was found that the maintenance cost is zero during the first year and it increases by Rs. 200 every year thereafter.
(a) When should the equipment be replaced if i = 0%?
(b) When should the equipment be replaced if i = 12%?
(a) When i = 0%. In this problem
(i) First cost = Rs. 4,000
(ii) Maintenance cost is Rs. 0 during the first year and it increases by Rs. 200 every year thereafter.
This is summarized in column B of Table
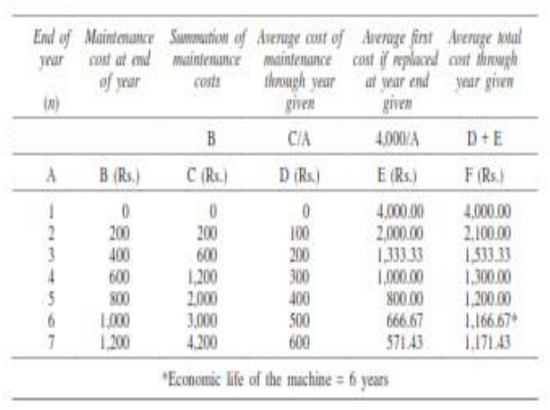
Column C summarizes the summation of maintenance costs for each replacement period. The value corresponding to any end of year in this column represents the total maintenance cost of using the equipment till the end of that particular year.
Average total cost = [ First cost (FC) + Summation of maintenance cost ] / Replacement period
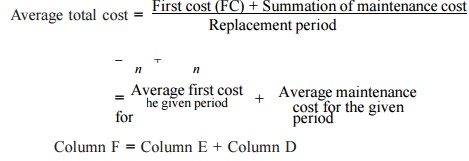
Column F = Column E + Column D
The value corresponding to any end of year (n) in Column F represents the average total cost of using the equipment till the end of that particular year.
For this problem, the average total cost decreases till the end of year 6 and then it increases. Therefore, the optimal replacement period is six years, i.e. economic life of the equipment is six years.
(b) When interest rate, i = 12%. When the interest rate is more than 0%, the steps to be taken for getting the economic life are summarized with reference to Table
Table Calculations to Determine Economic Life (First cost = Rs. 4,000, Interest = 12%)
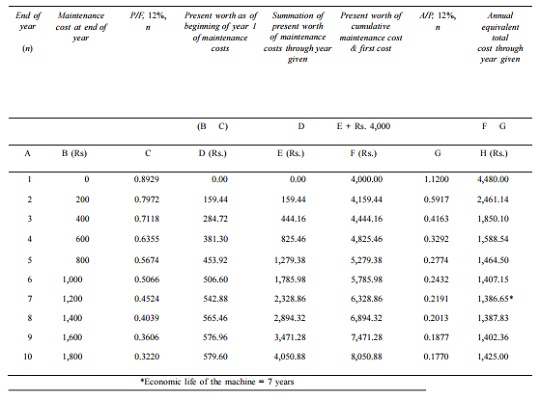
The steps are summarized now:
1. Discount the maintenance costs to the beginning of year 1.
Column D = Column B
1/ (1 + i)n
= Column B (P/F, i, n) = Column B Column C.
2. Find the summation of present worth of maintenance costs through the year given (Column E = Column D).
3. Find Column F by adding the first cost of Rs. 4,000 to Column E.
4. Find the annual equivalent total cost through the years given.
Column H = Column F i(1 + i)n / (1 + i)n − 1
= Column F (A/P, 12%, n) = Column F Column G
5. Identify the end of year for which the annual equivalent total cost is minimum.
For this problem, the annual equivalent total cost is minimum at the end of year 7. Therefore, the economic life of the equipment is seven years.
Replacement Of Exist Ing Asset With A New Asset
In this section, the c oncept of comparison of replacement of an existing asset with a new asset is presented. In this analysis, the annual equivalent cost of each alternative should be co mputed first.
Then the alternative which has the least cost should be selected as the best alternative. Before discu ssing details, some preliminary concepts whic h are essential for this type of replacem ent analysis are presented.
Capital Recovery with Return
Consider the follow ing data of a machine.
Let
P = purchase priice of the machine,
F = salvage valu e of the machine at the end of machine life,
n = life of the m achine in years, and
i = interest rate,, compounded annually
The corresponding cash flow diagram is shown in Fig
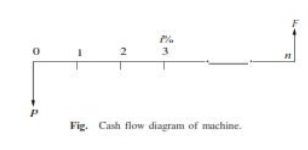
The equation for the annual equivalent amount for the above cash flow diagram is
AE(i) = (P – F ) (A/P, i, n) + F i
This equation represents the capital recovery with return.
Concept of Challenger and Defender
o If an existing equipment is considered for replacement with a new equipment, then the existing equipment is known as the defender and the new equipment is known as challenger.
o Assume that an equipment has been purchased about three years back for Rs. 5,00,000 and it is considered for replacement with a new equipment. The supplier of the new equipment will take the old one for some money, say, Rs. 3,00,000.
o This should be treated as the present value of the existing equipment and it should be considered for all further economic analysis.
o The purchase value of the existing equipment before three years is now known as sunk cost, and it should not be considered for further analysis.
EXAMPLE
Two years ago, a machine was purchased at a cost of Rs. 2,00,000 to be useful for eight years. Its salvage value at the end of its life is Rs. 25,000. The annual maintenance cost is Rs. 25,000.
The market value of the present machine is Rs. 1,20,000. Now, a new machine to cater to the need of the present machine is available at Rs. 1,50,000 to be useful for six years. Its annual maintenance cost is Rs. 14,000. The salvage value of the new machine is Rs. 20,000.
Using an interest rate of 12%, find whether it is worth replacing the present machine with the new machine.
Solution
Alternative 1—
Present machine
Purchase price = Rs. 2,00,000
Present value (P) = Rs. 1,20,000
Salvage value (F) = Rs. 25,000
Annual maintenance cost (A) = Rs. 25,000
Remaining life = 6 years
Interest rate = 12%
The cash flow diagram of the present machine is illustrated in Fig.
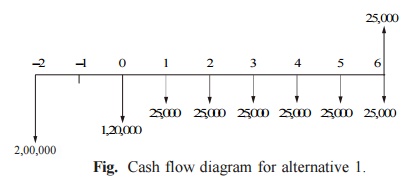
Fig. Cash flow diagram for alternative 1.
annual maintenance cost for the preceding periods are not shown in this figure. The annual equivalent cost is computed as
AE(12%) = (P – F)(A/P, 12%, 6) + F i + A
= (1,20,000 – 25,000)(0.2432) + 25,000 0.12 + 25,000
= Rs. 51,104
Alternative 2 —
New machine
Purchase price (P) = Rs. 1,50,000
Salvage value (F) = Rs. 20,000
Annual maintenance cost (A) = Rs. 14,000
Life = 6 years
Interest rate = 12%
The cash flow diagram of the new machine is depicted in Fig.
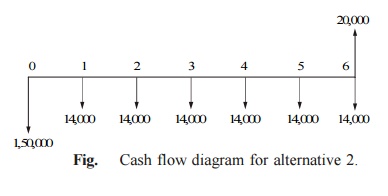
Fig. Cash flow diagram for alternative 2.
The formula for the annual equivalent cost is
AE(12%) = (P – F)(A/P, 12%, 6) + F i + A
= (1,50,000 – 20,000)(0.2432) + 20,000 0.12 + 14,000
= Rs. 48,016
Since the annual equivalent cost of the new machine is less than that of the present machine, it is suggested that the present machine be replaced with the new machine.
Electronic items like transistors, resistors, tubelights, bulbs, etc. could fail all of a sudden, instead of gradual deterioration. The failure of the item may result in complete breakdown of the system. The system may contain a collection of such items or just one item, say a tubelight.
Therefore, we use some replacement policy for such items which would avoid the possibility of a complete breakdown.
The following are the replacement policies which are applicable for this situation.
(i) Individual replacement policy. Under this policy, an item is replaced immediately after its failure.
(ii) Group replacement policy. Under this policy, the following decision is
made:
At what equal intervals are all the items to be replaced simultaneously with a provision to replace the items individually which fail during a fixed group replacement period?
There is a trade-off between the individual replacement policy and the group replacement policy. Hence, for a given problem, each of the replacement policies is evaluated and the most economical policy is selected for implementation. This is explained with two numerical problems.
EXAMPLE
The failure rates of transistors in a computer are summarized in Table .
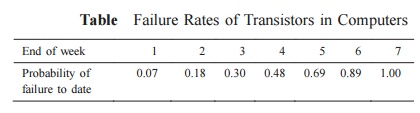
The cost of replacing an individual failed transistor is Rs. 9. If all the transistors are replaced simultaneously, it would cost Rs. 3.00 per transistor. Any one of the following two options can be followed to replace the transistors:
(a) Replace the transistors individually when they fail (individual replacement policy).
(b) Replace all the transistors simultaneously at fixed intervals and replace the individual transistors as they fail in service during the fixed interval (group replacement policy).
Find out the optimal replacement policy, i.e. individual replacement policy or group replacement policy. If group replacement policy is optimal, then find at what equal intervals should all the transistors be replaced.
Solution
Assume that there are 100 transistors in use.
Let,
pi be the probability that a transistor which was new when placed in position for use, fails during the ith week of its life. Hence,
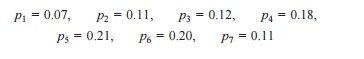
Since the sum of pis is equal to 1 at the end of the 7th week, the transistors are sure to fail during the seventh week.
Assume that
(a) transistors that fail during a week are replaced just before the end of the week, and
(b) the actual percentage of failures during a week for a sub-group of transistors with the same age is same as the expected percentage of failures during the week for that sub-group of transistors.
Let
Ni = the number of transistors replaced at the end of the ith week
N0 = number of transistors replaced at the end of the week 0 (or at the beginning of the first week).
= 100
N1 = number of transistors replaced at the end of the 1st week
= N0 p1 = 100 0.07 = 7
N2 = number of transistors replaced at the end of the 2nd week
= N0 p2 + N1 p1
= 100 0.11 + 7 0.07 = 12
N3 = N0 p3 + N1 p2 + N2 p1
= 100 0.12 + 7 0.11 + 12 0.07
= 14
N4 = N0 p4 + N1 p3 + N2 p2 + N3 p1
= 100 0.18 + 7 0.12 + 12 0.11 + 14 0.07
= 21
N5 = N0 p5 + N1 p4 + N2 p3 + N3 p2 + N4 p1
= 100 0.21 + 7 0.18 + 12 0.12 + 14 0.11 + 21 0.07
= 27
N6 = N0 p6 + N1 p5 + N2 p4 + N3 p3 + N4 p2 + N5 p1
= 100 0.2 + 7 0.21 + 12 0.18 + 14 0.12 + 21 0.11 + 27 0.07
= 30
N7 = N0 p7 + N1 p6 + N2 p5 + N3 p4 + N4 p3 + N5 p2
+ N6 p1
= 100 0.11 + 7 0.2 + 12 0.21 + 14 0.18 + 21 0.12
+ 27 0.11 + 30 0.07
= 25
Calculation of individual replacement cost
Expected life of each transistor = 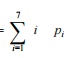
= 1 0.07 + 2 0.11 + 3 0.12 + 4 0.18
+ 5 0.21 + 6 0.2 + 7 0.11
= 4.39 weeks
Average No. of failures/week = 100/4.39 = 23
(approx.) Therefore,
Cost of individual replacement
= (No. of failures/week Individual replacement cost/transistor) = 23 9 = Rs. 207.
Determination of group replacement cost
Cost of transistor when replaced simultaneously = Rs. 3
Cost of transistor when replaced individually = Rs. 9
The costs of group replacement policy for several replacement periods are summarized in Table.
Table Calculations of Cost for Preventive Maintenance

From Table it is clear that the average cost/week is minimum for the fourth week. Hence, the group replacement period is four weeks.
Individual replacement cost/week = Rs. 207
Minimum group replacement cost/week = Rs. 196.50
Since the minimum group replacement cost/week is less than the individual replacement cost/week, the group replacement policy is the best, and hence all the transistors should be replaced once in four weeks and the transistors which fail during this four-week period are to be replaced individually.
Related Topics