Correlation and Regression analysis - SpearmanŌĆÖs Rank Correlation Coefficient | 11th Business Mathematics and Statistics(EMS) : Chapter 9 : Correlation and Regression analysis
Chapter: 11th Business Mathematics and Statistics(EMS) : Chapter 9 : Correlation and Regression analysis
SpearmanŌĆÖs Rank Correlation Coefficient
Rank correlation
SpearmanŌĆÖs Rank Correlation Coefficient
In 1904, Charles Edward Spearman,
a British psychologist found out the method of ascertaining the coefficient of
correlation by ranks. This method is based on rank. This measure is useful in
dealing with qualitative characteristics, such as intelligence, beauty,
morality, character, etc. It cannot be measured quantitatively, as in the case
of PearsonŌĆÖs coefficient of correlation.
Rank
correlation is applicable only to individual observations. The result we get
from this method is only an approximate one, because under ranking method
original value are not taken into account. The formula for SpearmanŌĆÖs rank
correlation which is denoted by Žü
(pronounced as row) is
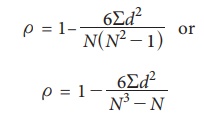
where d
= The difference of two ranks = R X
- RY and
N = Number of paired observations.
Rank
coefficient of correlation value lies between ŌĆō1 and +1. Symbolically, ŌĆō1ŌēżŽüŌēż+1
When we
come across spearmanŌĆÖs rank correlation, we may find two types of problem
(i) When
ranks are given
(ii) When
ranks are not given
Example 9.6
The
following are the ranks obtained by 10 students in Statistics and Mathematics
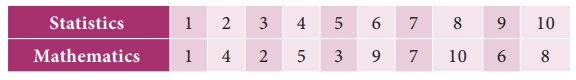
Find the
rank correlation coefficient.
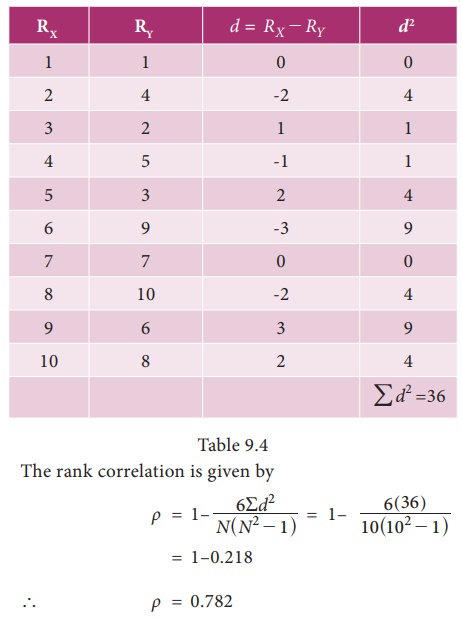
Solution:
Let RX is considered for the
ranks of Statistics and RY
is considered for the ranks of mathematics.
Example 9.7
Ten
competitors in a beauty contest are ranked by three judges in the following
order
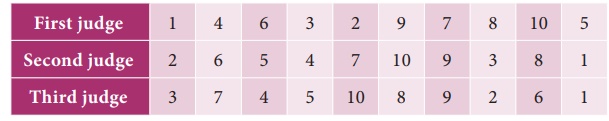
Use the
method of rank correlation coefficient to determine which pair of judges has
the nearest approach to common taste in beauty?
Solution:
Let RX,RY,RZ denote the ranks by First judge, Second
judge and third judge respectively

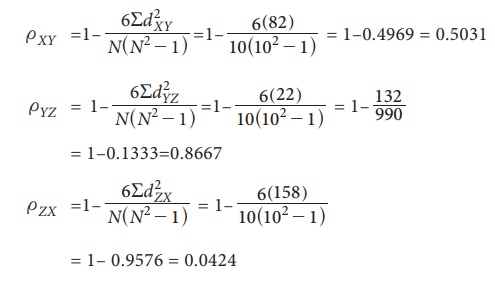
Since the
rank correlation coefficient between Second and Third judges i.e., ŽüYZ is positive and weight
among the three coefficients. So, Second judge and Third judge have the nearest
approach for common taste in beauty.
Example 9.8
Calculate
rank correlation coefficient of the following data
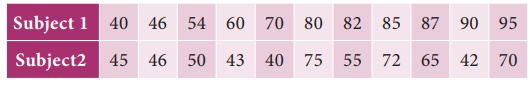
Solution:
Let X is considered for Subject1 and Y is considered for Subject2

Exercise 9.1
1. Calculate
the correlation co-efficient for the following data
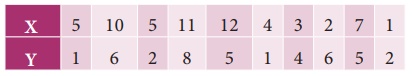
2. Find
coefficient of correlation for the following:
3. Calculate
coefficient of correlation for the ages of husbands and their respective wives:
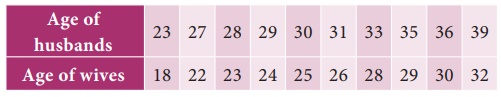
4. Calculate
the coefficient of correlation between X
and Y series from the following data
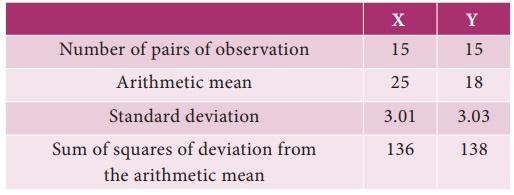
Summation of product deviations of X and Y series from their respective arithmetic means is 122.
5. Calculate
correlation coefficient for the following data

6. Find
coefficient of correlation for the following:

7. An
examination of 11 applicants for a accountant post was taken by a finance
company. The marks obtained by the applicants in the reasoning and aptitude
tests are given below.
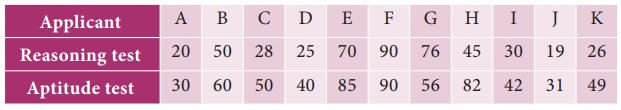
Calculate SpearmanŌĆÖs rank correlation
coefficient from the data given above.
8. The
following are the ranks obtained by 10 students in commerce and accountancy are
given below
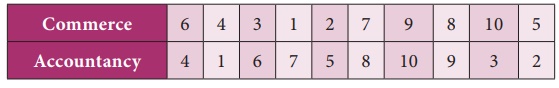
To what extent is the knowledge
of students in the two subjects related?
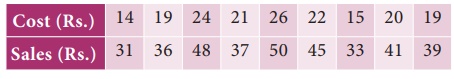
9. A random
sample of recent repair jobs was selected and estimated cost and actual cost
were recorded.
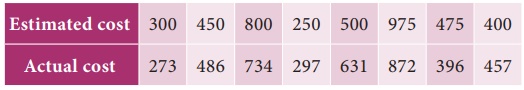
Calculate the value of spearmanŌĆÖs
correlation coefficient.
10. The
rank of 10 students of same batch in two subjects A and B are given below.
Calculate the rank correlation coefficient.
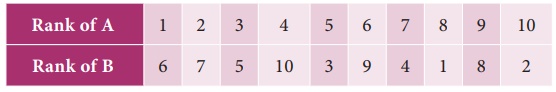
Related Topics