Refractometry

REFRACTOMETRY

INTRODUCTION

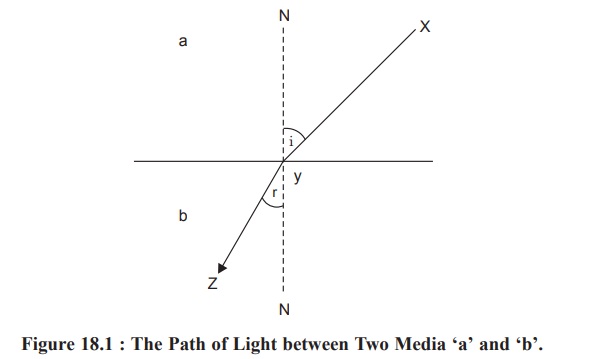
Light passes more rapidly through a vacuum than through a substance (medium). It has been observed that when a ray of light happens to pass from one medium (a) into another medium (b) it is subjected to refraction (Figure 18.1). In other words, the ray travels at a lower velocity in the relatively more optically dense medium (b) than in medium (a) which is less optically dense. It is a common practice to compare the refractive indices of liquids to that of air.

According to Snell’s Law we have :

        .....................(1)

where, i = Angle of incidence,

r = Angle of refraction, and

n = Refractive index of medium (b) relative to medium (a)

Critical Angle vis-a-vis Refractive Index

Figure 18.2, represents the critical angle which is used invariably in refractometry. Considering a narrow band of rays, x-y, held near to the boundary between the two media ‘a’ and ‘b’ (Figure 18.2), and viewed at Z, one may observe a band of light. This particular band has a sharp edge at y, where the actual ray (y-y) may be seen. However, no rays are to be seen in the y-y′ region. Therefore, we have :

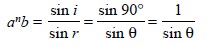       ...................(2)

 Thus, a measurement of the critical angle  θ  may ultimately offer the exact refractive index of medium (b).

It is pertinent to mention here that the refractive index of a substance is not a static (constant) property of the substance but it alters with (a) wavelength and (b) temperature.

Therefore, conventionally the temperature at which the refractive index is measured is usually desig-nated as a superscript numerical on n ; whereas the wave-length of light employed as a subscript capital.

Thus, we have : n_D²⁰ , where 20 specifies the temperature expressed in (°C) at which RI has been measured and D represents the sodium D-light (λ = 589.3 nm).